Question

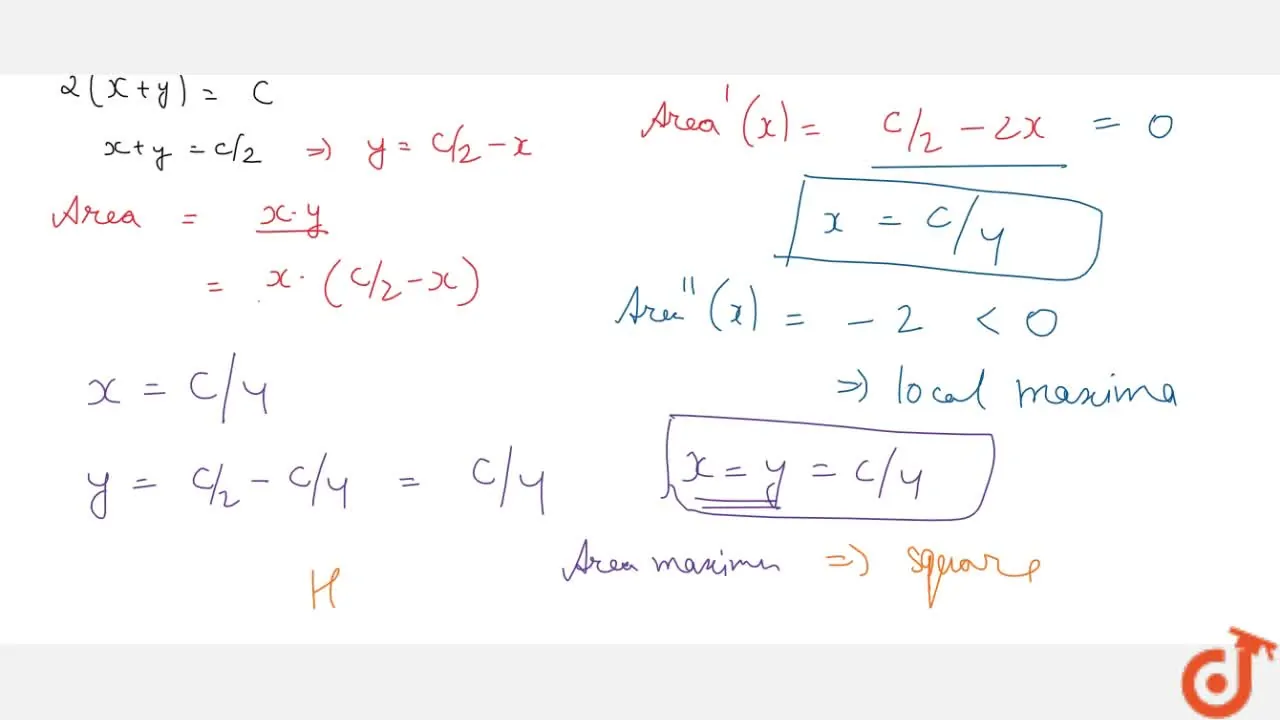
Show that all the
rectangles with a given perimeter, the square has the largest area.

Explanation

Let x and y be length and breadth

And A and P be area and perimeter

`A=x cdot y text { and } P=2(x+y) `

`Rightarrow A=x(frac{P}{2}-x) (because y=frac{P}{2}-x) `

`Rightarrow A =frac{P}{2} x-x^{2} quad Rightarrow frac{d A}{d x}=frac{P}{2}-2 x`

For maximum and minimum of `A`.

`Rightarrow quad frac{P}{2}-2 x=0 quad Rightarrow quad x=frac{P}{4}`

Again `frac{d^{2} A}{d x^{2}}=-2`

`Rightarrow quad(frac{d^{2} A}{d x^{2}})_{x=frac{P}{4}}=0`

Hence, `A` is maximum for `x=frac{P}{4}`

`therefore quad y=frac{P}{2}-frac{P}{4}=frac{P}{4}`
Hence it is proved that with given perimeter, rectangle having largest area must be square.