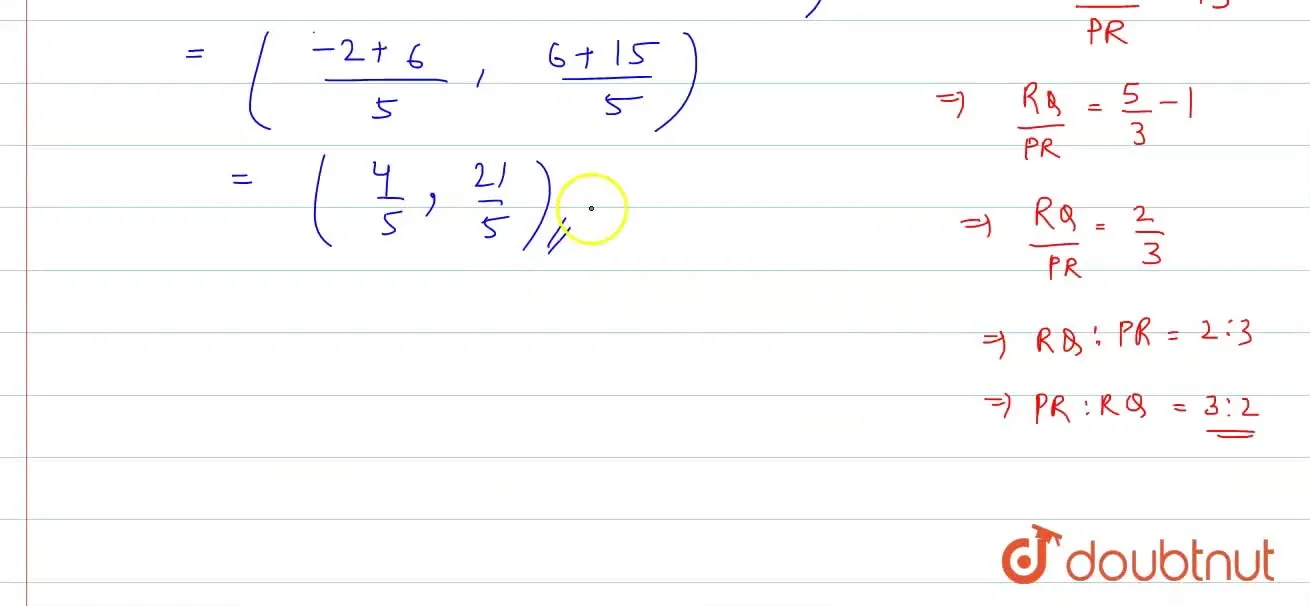Find the coordinates of the point R on the line segment joining the points P(−1,3) and Q (2,5) such that PR =35 PQ.
According to the question ,
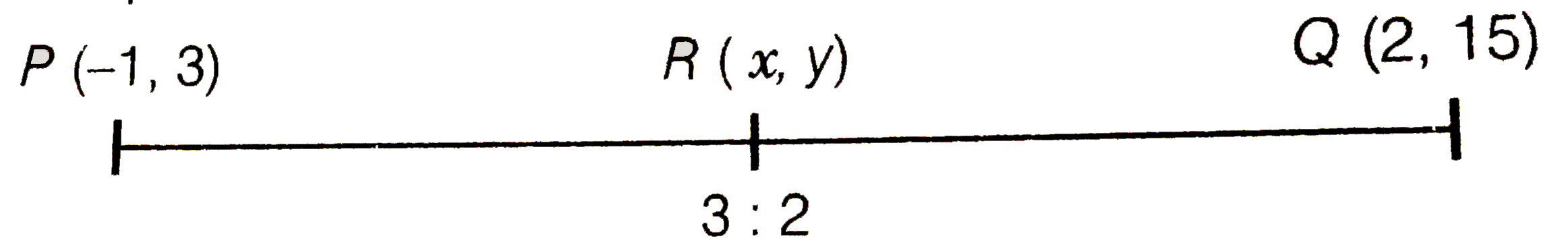
Given that , PR =35PQ
⇒PQPR=53
⇒PR+RQPR=53
⇒1+RQPR=53
⇒PQPR=53−1=23
∴PQ:PR=2:3
or ∴PR:RQ=3:2
Suppose , R (x,y) be the point which divides the line segment joining the points P (-1,3) and Q(2,5) in the ration 3:2.
∴(x,y)={3(2)+2(−1)3+2,3(5)+2(3)3+2}
[∵ by internal section formula , ∴(x,y)={m2x1+m1x2m1+m2,m2y1+m1y2m1+m2}]
=(6−25,15+65)=(45,215)
Hence , the required coordinates of the point R is (45,215).
Related Playlists
- BOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 10.4 Long Answer type Questions7videos
CONSTRUCTIONS
- BOOK - NCERT EXEMPLARCHAPTER - INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONSEXERCISE - Introduction To Trigonometry And Its Applications60videos
INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONS
Similar Questions
Write coordinates of the mid point P of the line segment joining the point A(-2,-1) and B(4,5)
View SolutionFind the coordinates of the points which trisect the line segment joining the points (2,3)and(6,5).
View SolutionFind the coordinates of a point P, which lies on the line segment joining the points A(-2,-3) and B(2. -4) such that AP=37AB.
View SolutionThe mid point of the line segment joining the points (−5,7)and(−1,3) is
View SolutionFind the coordinates of the points of trisection of the line segment joining the points A(7, -2) and B(1, 5).
View SolutionFind the coordinates of the point of bisection of the line segment joining the points (7, - 2) and (-5, -4).
View SolutionFind the coordinates of the points of trisection of the line segment joining the points A(-5, 6) and B(4, -3).
View SolutionThe coordinates of the point P dividing the line segment joining the points A(1, 3) and B(4, 6) in the ratio 2:1 is
View SolutionFind the point P divides the line segment joining the points (-1,2) and (4,-5) in the ratio 3:2
View SolutionFind the coordinates of that point, which is the line segment joining points (−1,7) and (4,−3) in the ratio of 2:3.
View Solution