Let there be n resistors R1...Rn with Rmax=max(R1....Rn) and Rmin=min{R1...Rn}. Show that when they are connected in parallel the resultant resistance Rp=Rmin and when they are connected in series, the resultant resistance RS>Rmax. Interpret the result physically.
When all resistances are connected in parallel, the resultant resistance Rp is given by
1Rp=1R1+.....+1Rn
On multiplying both sides by Rmin we have
RminRp=RminR1+RminR∘+....+RminRn
Here, in RHSlt there exist one term RminRmin=1 and other terms are positive, so we have
RminRp=RminR1+RminR2+....+RminRn>1
This shows that the resultant resistance Rp<Rmin
thus, in parallel combination, the equivalent resistance of resistors is less than the minimum resistance available in combination of resistors. Now, in series combination, the equivalent resistance is given by
RS=R1+...+Rn
Here, in RHS, there exist one term having resistance Rmax
So, we have
or RS=R1+...+Rmax+...+...+Rn
RS=R1+...+Rmax...+Rn=Rmax+...(R1+...+)Rn
or RS≥Rmax
or RS=Rmax(R1+...+Rn)
Thus, in series combination, the equivalent resistance of resistors of resistors is greater than the maximum resistance available in combination resistors. Physical interpretation.
In Fig (b), Rmin provides an equivalent route as in Fig. (a) for current. But in addition there are (n-1) routes by the remaining (n-1) resistors. Current in fig. (b) is greater than current in Fig. (a). Effective resistance in Fig (b) <Rmin Second circuit evidently affords a greater resistance.
In figure. (d), Rmax provides an equivalent route as in fig. (c) for current. Current in figure. (d)< current in figure (c) Effective resistance in fig. (b) <Rmax second circuit evidently affords a greater resistance.
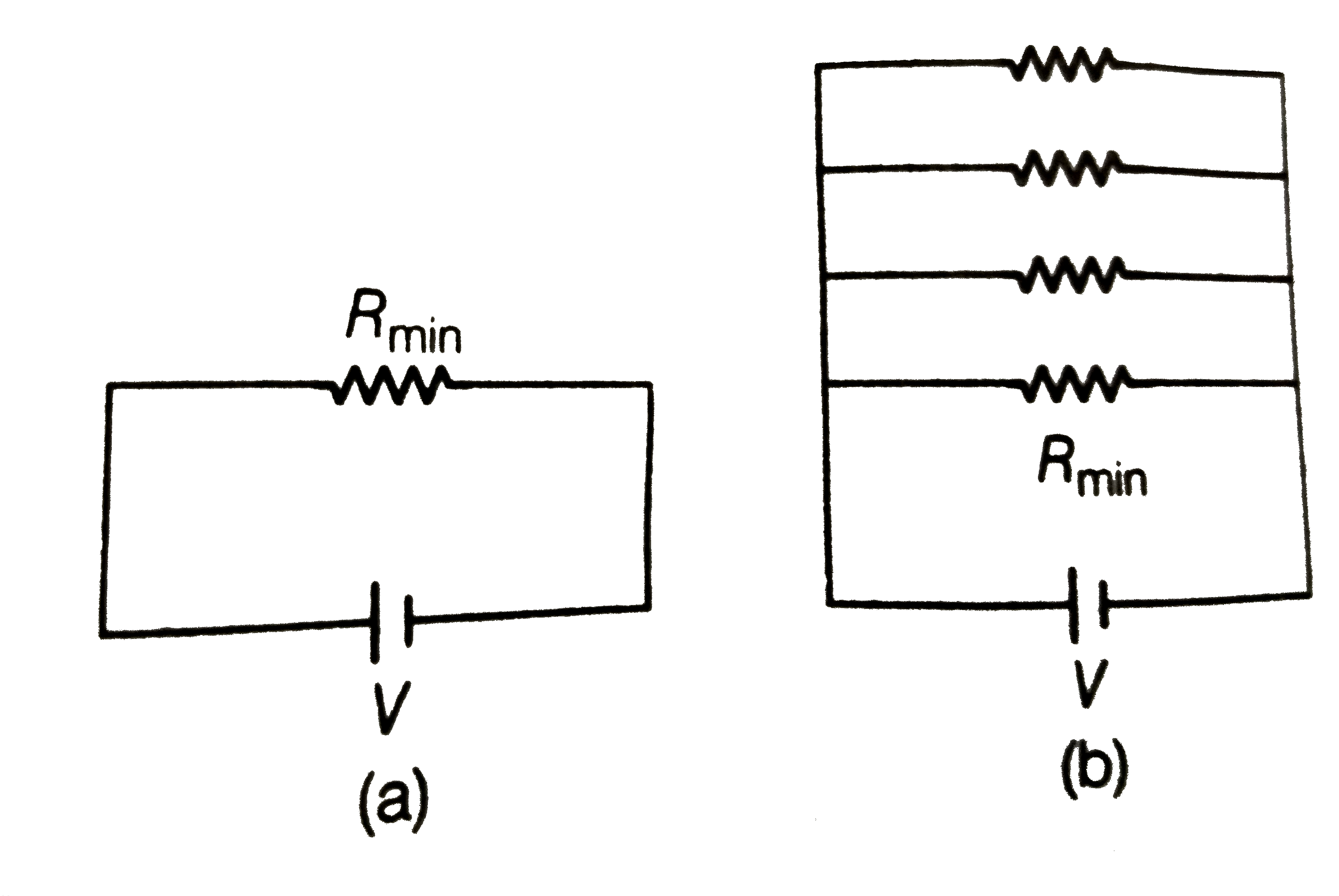
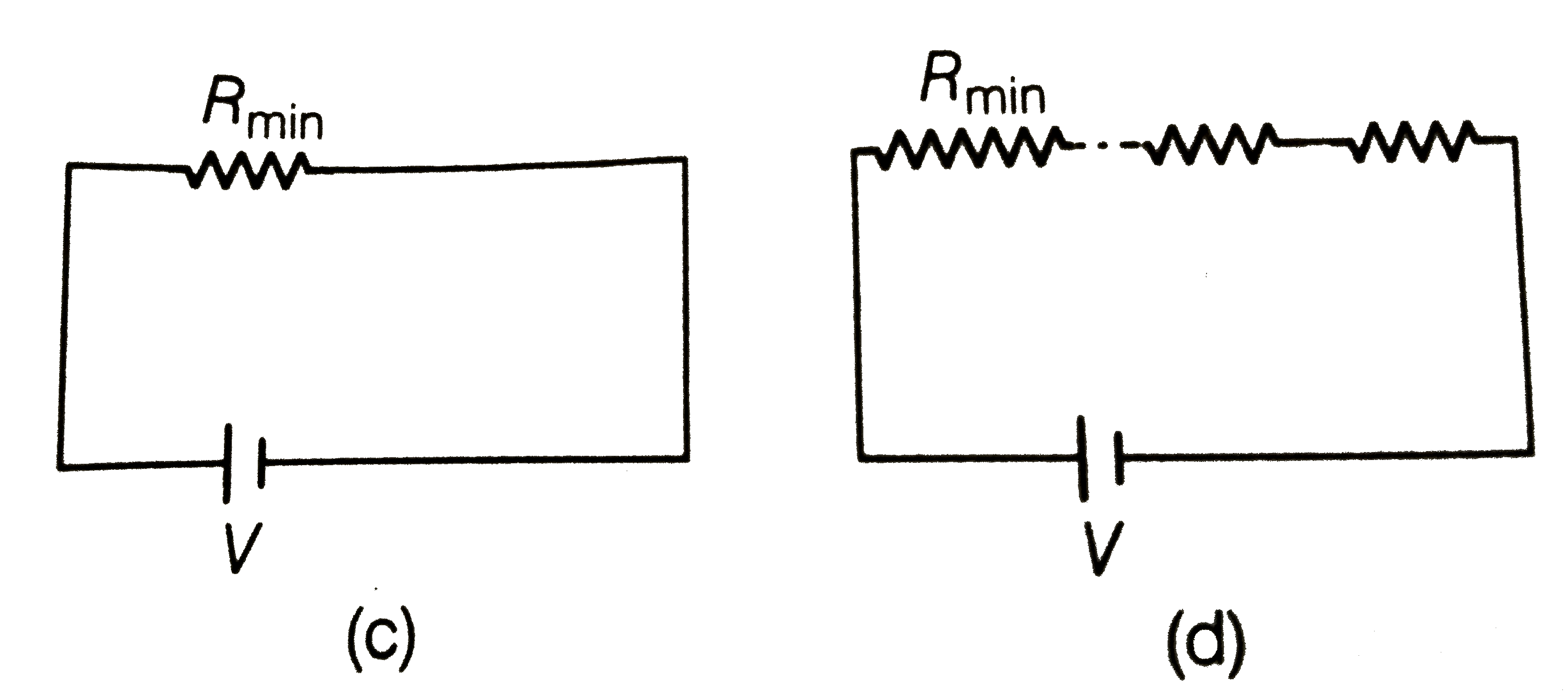 .
.
Topper's Solved these Questions
Similar Questions
If resistors of resistance R1 and R2 are connected in parallel,then resultant resistance is "
View SolutionThe resultant resistance of n wires each of resistance r ohms is R, when they are connected in parallel. When these n resistances are connected in series, the resultant resistance will be
View Solution
Knowledge Check
Two resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
AR>R1+R2BR1<R<R2CR2<R<(R1+R2)DR<R1There are n similar conductors each of resistance R . The resultant resistance comes out to be x when connected in parallel. If they are connected in series, the resistance comes out to be
Ax/n2Bn2xCx/nDnxTwo resistors of resistances R1=(300±3)Ω and R2=(500±4)Ω are connected in series. The equivalent resistance of the series combination is
A(800±1)ΩB(800±7)ΩC(200±7)ΩD(200±1)Ω
The resultant resistance of n wires each of resistance r ohms is R ,when they are connected in parallel.When these n resistances are connected in series,the resultant resistance will be
View SolutionWhen two resistors of resistances R1andR2 are connected in parallel, the net resistance is 3Ω. When connected in series, its value is 16Ω. Calculate the values of R1andR2.
View SolutionTwo resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View SolutionThe resultant resistance value of n resistance each of r ohms and connected is series is X. When those n , resistance are connected in parallel , the resultant values is .
View SolutionA resistance R2 is connected in parallel with a resistance R1 what resistance R3 must be connected in series with the combination of R1andR2 so that the equivalent resistance is equal to the resistance R1 -
View Solution