Find the equation of the circle which touches the x - axis and whose center is (1,2).
A
x2+y2−2x−4y+1=0
B
x2−y2+2x−6y+2=0
C
x2−y2+4x−6y+5=0
D
none of these
Video Solution
Text Solution
The correct Answer is:A
Given that , centre of the circle is (1,2)
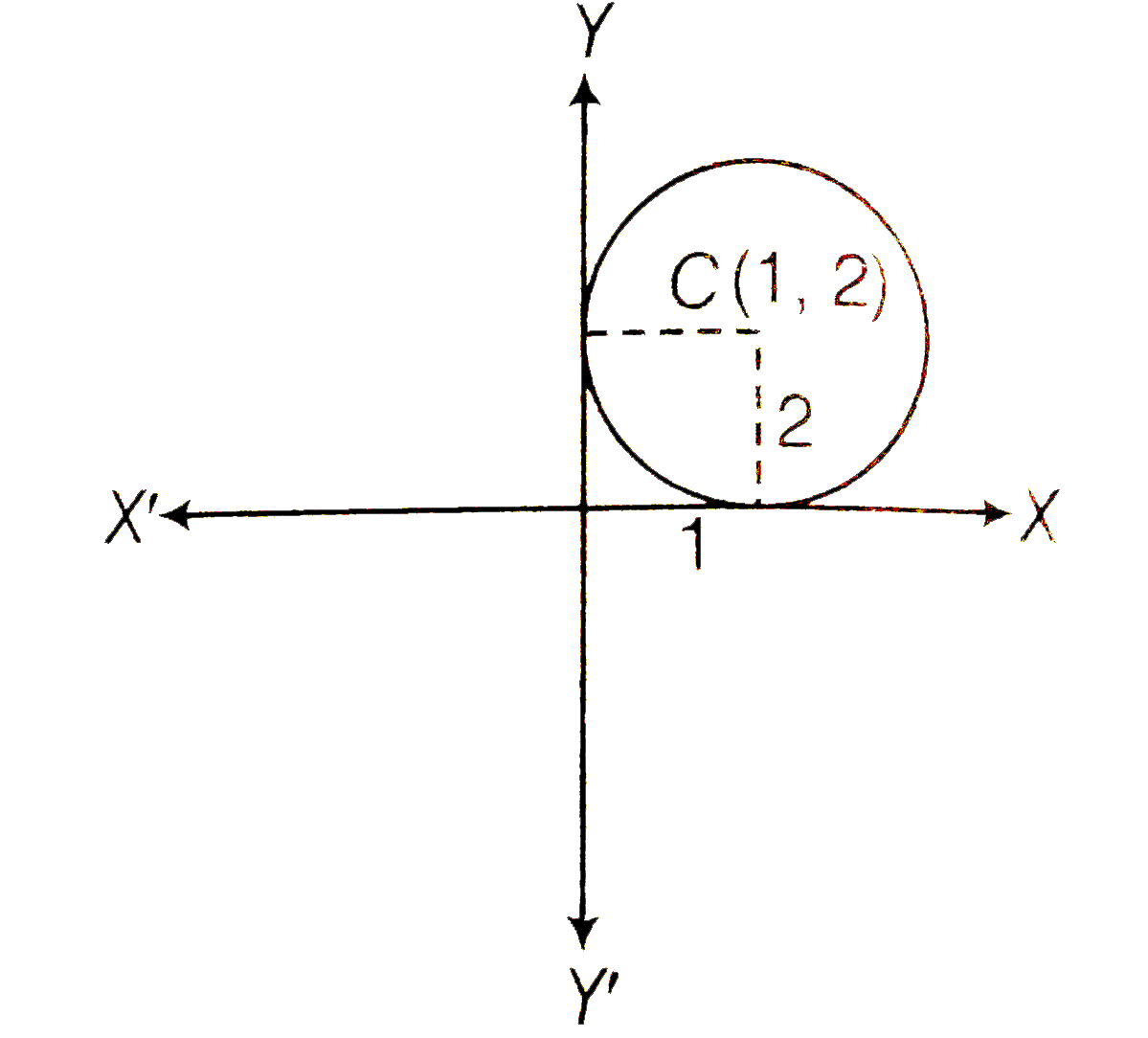
∵ Radius =2
So, the equation of the circle is
(x−1)2+(y−)2=22
⇒x2−2x+1+y2−4y+4=4
⇒x2−2x+y2−4y+1=0
⇒x2+y2−2x−4y+1=0
|
Topper's Solved these Questions
- CONIC SECTIONSBOOK - NCERT EXEMPLARCHAPTER - CONIC SECTIONSEXERCISE - Long answer
10 Videos
- CONIC SECTIONSBOOK - NCERT EXEMPLARCHAPTER - CONIC SECTIONSEXERCISE - True/False
8 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - NCERT EXEMPLARCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - OBJECTIVE TYPE QUESTIONS
16 Videos
- INTRODUCTION TO THREE DIMENSIONAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - Fillers
16 Videos
Similar Questions
Knowledge Check
Find the equation of a circle which touches the X−aξs and whose centre is (1,2).
A(x−1)2+(y−2)2=22Bx2+y2=22C(x−2)2+(y−1)2=22Dnone of theseThe equation of the circle which touches both axes and whose centre is (x1,y1) is
Ax2+y2+2x1(x+y)+x21=0Bx2+y2−2x1(x+y)+x21=0Cx2+y2=x21+y21Dx2+y2+2xx1+2yy1=0The equation of the circle which touches both the axes and whose radius is a, is
Ax2+y2−2ax−2ay+a2=0Bx2+y2+ax+ay−a2=0Cx2+y2+2x+2ay−a2=0Dx2+y2−ax−ay+a2=0
(i) Find the equation of a circle which touches both the axes and whose centre is (2,2).
(ii) Find the equation of a circle, touching the lines x=0, y=0 and x=6.
View SolutionFind the equation of the circle which touches the coordinate axes and whose centre lies on the line x−2y=3.
View SolutionFind the equation of the circle which touches : y-axis and has centre at (2, 3)
View SolutionFind the equation of a circle which touches the X-axis and whose centre is (asinθ,acosθ).
View SolutionFind the equation of a circle which touches both the axes and whose radius is 2 units
View Solution
