A galvanometer having full scale deflection current of 10 mA and resistance 8Ω is joined in circuit as shown. When the terminals A and B are used and C remains open, the range of the ammeter is I. When the terminals A and C are used and B remains open, then the range of the ammeter is
I
I2
I3
2I3
The correct Answer is:B
To solve the problem step by step, we will analyze the circuit configurations and apply the principles of current division and resistance in series and parallel.
Step 1: Understand the Circuit Configuration
When terminals A and B are used with terminal C open, the galvanometer is connected in such a way that it measures the current flowing through the circuit. The galvanometer has a full-scale deflection current of 10 mA and a resistance of 8 ohms.
Step 2: Calculate the Total Resistance in the Circuit (AB Configuration)
In the configuration with terminals A and B, we have:
- The galvanometer resistance (R_g) = 8 ohms
- Two resistors of 2 ohms each in parallel with the galvanometer.
The total resistance (R_total) when using A and B can be calculated as follows:
1. The two 2-ohm resistors in parallel give a combined resistance (R_parallel):
Rparallel=R1⋅R2R1+R2=2⋅22+2=1 ohm
2. The total resistance in the circuit when A and B are used:
Rtotal=Rg+Rparallel=8+1=9 ohms
Step 3: Determine the Current through the Galvanometer (AB Configuration)
Using Ohm's law, the current flowing through the galvanometer (I_g) when the total current (I) is flowing through the circuit can be expressed as:
Ig=I⋅RparallelRg+Rparallel
Substituting the values:
Ig=I⋅19=I9
Step 4: Analyze the Second Configuration (AC Configuration)
When terminals A and C are used and terminal B is open, the configuration changes:
- The galvanometer is still 8 ohms.
- The two 2-ohm resistors are now in series, giving a total resistance of 4 ohms.
Step 5: Calculate the Total Resistance in the Circuit (AC Configuration)
The total resistance (R_total) when using A and C:
Rtotal=Rg+Rseries=8+4=12 ohms
Step 6: Determine the Current through the Galvanometer (AC Configuration)
Using Ohm's law again, the current through the galvanometer (I_g) when the total current (I') is flowing through the circuit can be expressed as:
Ig=I′⋅RseriesRg+Rseries
Substituting the values:
Ig=I′⋅412=I′3
Step 7: Equate the Galvanometer Currents from Both Configurations
Since the galvanometer measures the same current (I_g) in both configurations, we can set the two equations equal:
I9=I′3
Step 8: Solve for I'
Cross-multiplying gives:
3I=9I′⟹I′=I3
Conclusion
The range of the ammeter when terminals A and C are used is:
Range=I′=I3
When terminals A and B are used with terminal C open, the galvanometer is connected in such a way that it measures the current flowing through the circuit. The galvanometer has a full-scale deflection current of 10 mA and a resistance of 8 ohms.
In the configuration with terminals A and B, we have:
- The galvanometer resistance (R_g) = 8 ohms
- Two resistors of 2 ohms each in parallel with the galvanometer.
1. The two 2-ohm resistors in parallel give a combined resistance (R_parallel):
Rparallel=R1⋅R2R1+R2=2⋅22+2=1 ohm
2. The total resistance in the circuit when A and B are used:
Rtotal=Rg+Rparallel=8+1=9 ohms
Using Ohm's law, the current flowing through the galvanometer (I_g) when the total current (I) is flowing through the circuit can be expressed as:
Ig=I⋅RparallelRg+Rparallel
Substituting the values:
Ig=I⋅19=I9
When terminals A and C are used and terminal B is open, the configuration changes:
- The galvanometer is still 8 ohms.
- The two 2-ohm resistors are now in series, giving a total resistance of 4 ohms.
The total resistance (R_total) when using A and C:
Rtotal=Rg+Rseries=8+4=12 ohms
Using Ohm's law again, the current through the galvanometer (I_g) when the total current (I') is flowing through the circuit can be expressed as:
Ig=I′⋅RseriesRg+Rseries
Substituting the values:
Ig=I′⋅412=I′3
Since the galvanometer measures the same current (I_g) in both configurations, we can set the two equations equal:
I9=I′3
Cross-multiplying gives:
3I=9I′⟹I′=I3
The range of the ammeter when terminals A and C are used is:
Range=I′=I3
Topper's Solved these Questions
Similar Questions
Knowledge Check
A milli- ammeter of range 10mA and resistance 9Ω are joined in a circuit as shown in the fig. The meter gives full scale deflection, when current in the main circuit is I, and A and D are used as terminals. The value of I is
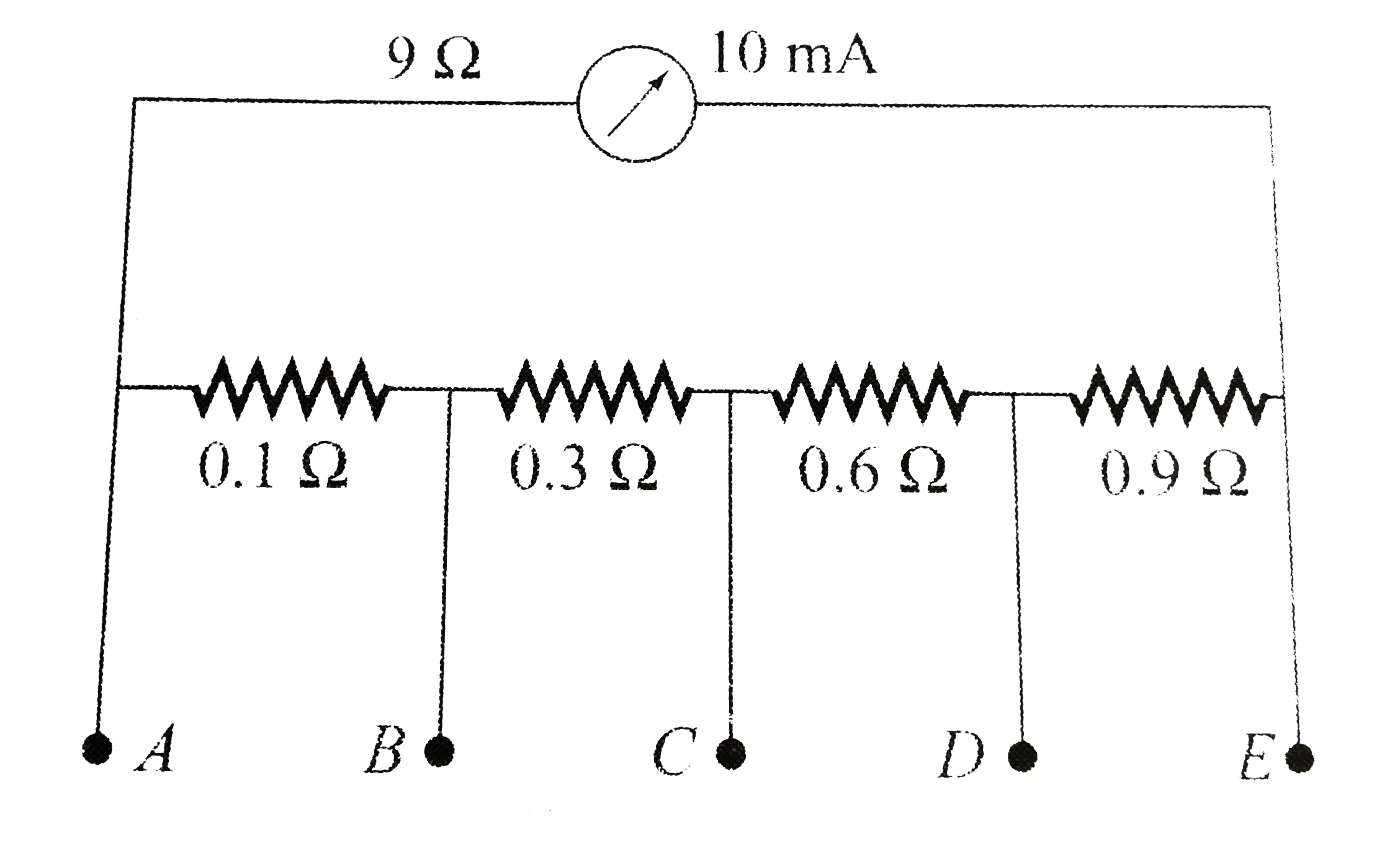 A1.09AB10.9 ACzeroD0.109 A
A1.09AB10.9 ACzeroD0.109 AA milli-ammeter of range 10mA and resistance 9Ω is joined in a circuit as shown in the figure. The meter gives full scale deflection, when circuit in the main circuit is 'I' and A and D are used as terminals. The value of I is
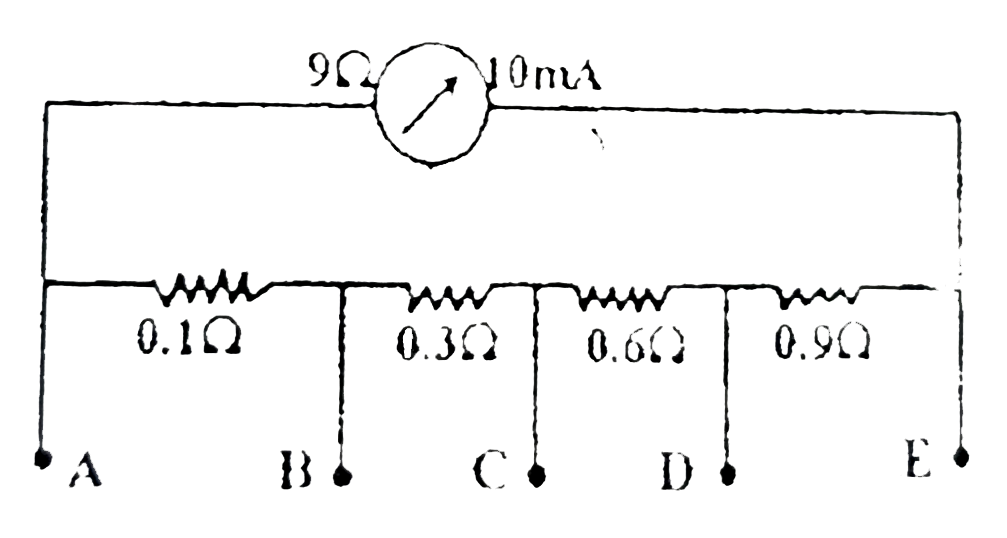 A1.09AB10.9ACzero.D0.109A
A1.09AB10.9ACzero.D0.109AA galvanometer gives full scale deflection when the current passed through it is 3 mA. Its resistance is 100 Ω with connecting additional resistance in series, then it can be used as voltmeter of range
A3.0 VB0.3 VC0.020 VD0.003 V
A galvanometer of resistance 120Ω gives full scale deflection for a current of 5mA. How can it be converted into an ammeter of range 0 to 5A? Also determine the net resistance of the ammeter.
View SolutionA galvanometer of resistance 500Ωgives a full scale deflection, for a current of 20mA. Even without using a high resistance in series, it can be used as a voltmeter. The range of the voltmetr will be
View SolutionA milliammeter of range 10mA and resistance 9Ω is joined in a circuit as shown. The metre gives full-scale deflection for curretn I when A and B are used as its terminals, i.e., current enters at A and leaves at B (C is left isolated). The value if I is
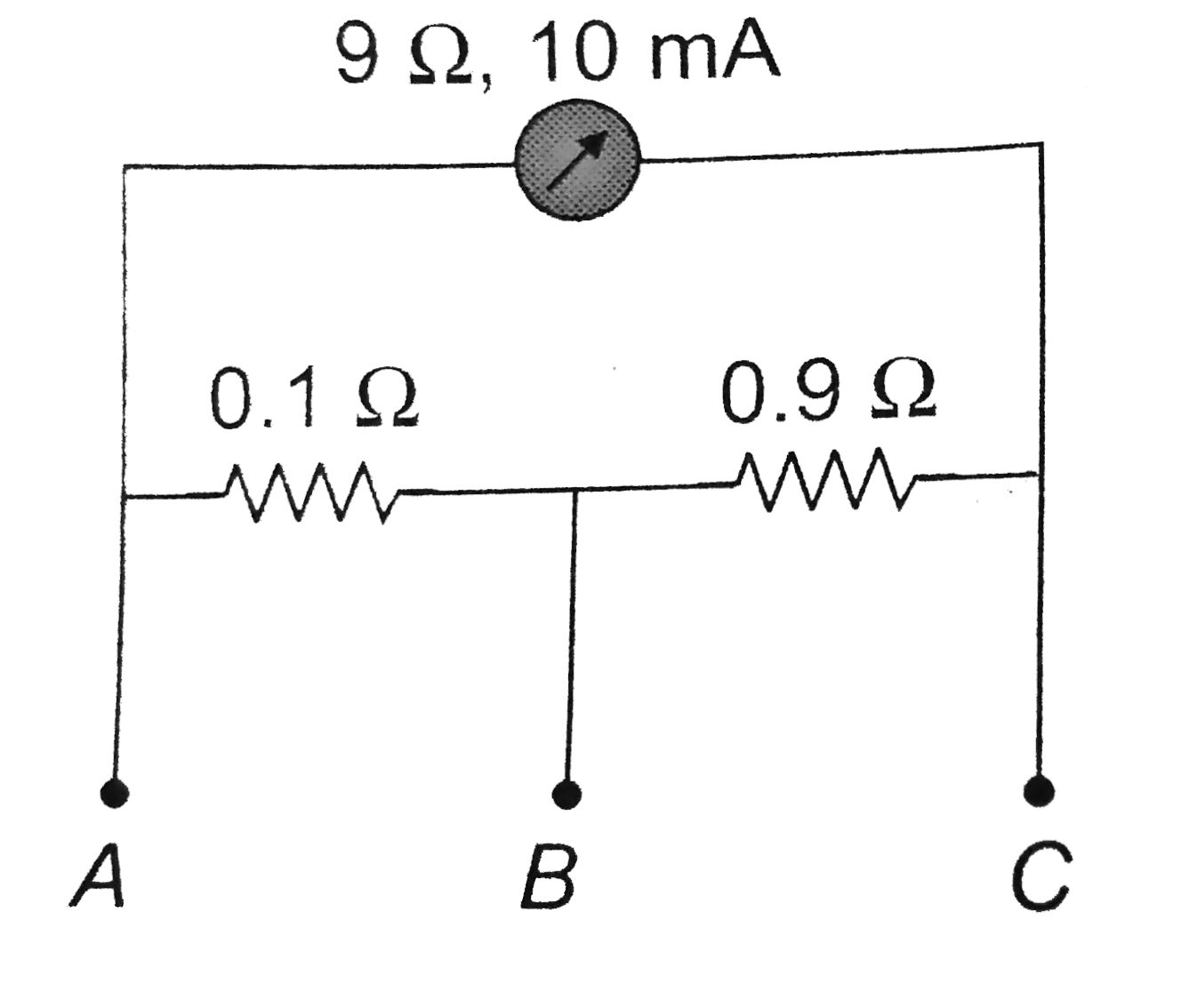
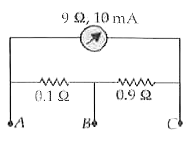
View SolutionA milliammeter of range 10 mA and resistance 9 Ω is joined in a circuit as shown in the figure. The metre gives full scale deflection for current / when A and B are used as its terminals, i.e., current enters at A and leaves at BC is left isolated). The value of /is
View SolutionA galvanometer gives full scale deflection when current passed throguht it is 1 m A. its resistance is 100Ω. Without connecting additional resistance in series with it , can be used as a voltmeter of range
View Solution
