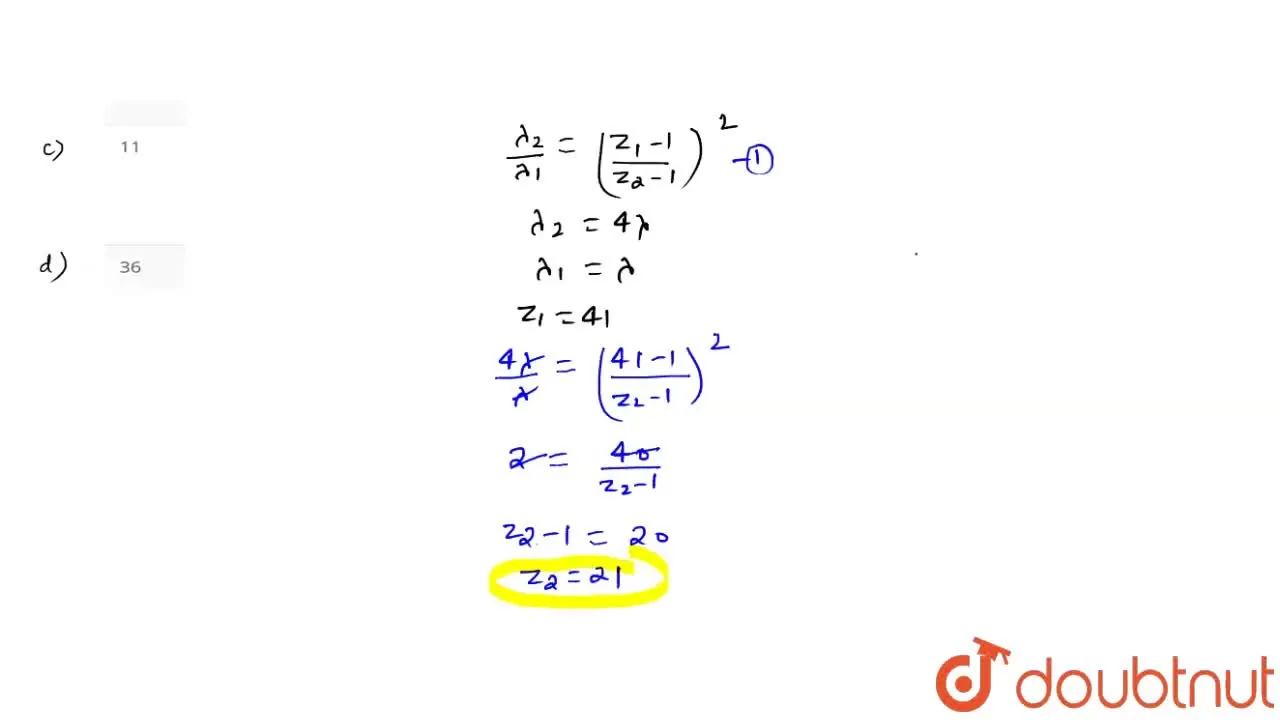
If the wavelength of Kα radiation emitted an atom of atomic number Z = 41 is λ , then the atomic number for an atom that emits Kα radiation with the wavelength 4λ, is
21
28
11
36
The correct Answer is:A
Step by step video, text & image solution for If the wavelength of K_(alpha) radiation emitted an atom of atomic number Z = 41 is lambda , then the atomic number for an atom that emits K_(alpha) radiation with the wavelength 4lambda, is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
ka wavelength emitted by an atom of atomic number E=11 is λ find the atomic number for an atomic that amils ka radiation with wavwlength 43.
View SolutionKα wavelength emitted by an atom of atomic number Z=11 is λ. Find the atomic number for an atom that emits Kα radiation with wavelength 4λ. (a) Z=6 (b) Z=4
(c ) Z=11 (d) Z=44.View SolutionThe wavelength of Kα line for an element of atomic number 43isλ. Then the wavelength of Kα line for an element of atomic number 29 is
View SolutionAn element of atomic number 9 emits Kα X-ray of wavelength λ. Find the atomic number of the element which emits Kα X-ray of wavelength 4λ.
View SolutionIn X-ray spectrum wavelength λ of line Kα depends on atomic number Z as
View SolutionThe value of wavelength radiation emitted by an H-atom, if atom is excited to state with principal quantum number four is :
View SolutionThe wavelength of the Kα line for an element of atomic number 57 is λ . What is the wavelength of the Kα line for the element of atomic number29 ?
View SolutionThe atomic number (2) of an element whose k, wavelength is λ is 11. The atomic number of an element whose wavelength is 4λ is equal to
View SolutionThe wavelength of the radiation emitted when an electron falls from Bohr 's orbit 4 to 2 in H atom is
View SolutionThe wavelength of the Kα line for an element of atomic number 57 is λ . What is the wavelength of the Kα line for the element of atomic number29 ?
View Solution