The magnetic field induction at the centre of a current - carrying circular coil (coil 1) and a closed coil (coil 2), shaped as a quarter of a disc is found to be equal in magnitude. If both the coils have equal area, then find the ratio of the currents flowing in coil 2 and coil 1.
The correct Answer is:2
To solve the problem, we need to find the ratio of the currents flowing in a circular coil (coil 1) and a quarter disc coil (coil 2) given that the magnetic field induction at their centers is equal and both coils have equal areas.
1. Identify the Areas of the Coils:
- For coil 1 (circular coil), the area A1 is given by:
A1=πR21
- For coil 2 (quarter disc), the area A2 is:
A2=14πR22
2. Set the Areas Equal:
Since both coils have equal areas:
A1=A2⟹πR21=14πR22
Dividing both sides by π:
R21=14R22
Taking the square root:
R1=12R2⟹R2R1=2
3. Magnetic Field Induction for Coil 1:
The magnetic field induction B1 at the center of a circular coil is given by:
B1=μ0I12R1
4. Magnetic Field Induction for Coil 2:
The magnetic field induction B2 at the center of a quarter disc is given by:
B2=μ0I28R2
5. Set the Magnetic Fields Equal:
Since B1=B2:
μ0I12R1=μ0I28R2
Canceling μ0 from both sides:
I12R1=I28R2
6. Substituting R2 in terms of R1:
From the previous step, we know R2=2R1:
I12R1=I28⋅2R1
Simplifying:
I12R1=I216R1
Canceling R1 from both sides:
I12=I216
7. Finding the Ratio of Currents:
Rearranging gives:
I2=8I1
Thus, the ratio of the currents I2I1 is:
I2I1=8
Final Answer:
The ratio of the currents flowing in coil 2 and coil 1 is:
I2I1=8:1
- For coil 1 (circular coil), the area A1 is given by:
A1=πR21
- For coil 2 (quarter disc), the area A2 is:
A2=14πR22
Since both coils have equal areas:
A1=A2⟹πR21=14πR22
Dividing both sides by π:
R21=14R22
Taking the square root:
R1=12R2⟹R2R1=2
The magnetic field induction B1 at the center of a circular coil is given by:
B1=μ0I12R1
The magnetic field induction B2 at the center of a quarter disc is given by:
B2=μ0I28R2
Since B1=B2:
μ0I12R1=μ0I28R2
Canceling μ0 from both sides:
I12R1=I28R2
From the previous step, we know R2=2R1:
I12R1=I28⋅2R1
Simplifying:
I12R1=I216R1
Canceling R1 from both sides:
I12=I216
Rearranging gives:
I2=8I1
Thus, the ratio of the currents I2I1 is:
I2I1=8
The ratio of the currents flowing in coil 2 and coil 1 is:
I2I1=8:1
Topper's Solved these Questions
Similar Questions
Knowledge Check
The magnetic induction at the centre of a current carrying circular of coil radius r, is
ADirectly proportional to rBInversely proportional rCDirectly proportional to rDInversely proportional to rThe magnetic field at the centre of current carrying coil is
Aμ0ni2rBμ02πnirCμ0ni4rDμ0niThe direction of magnetic moment of a current carrying circular coil is
Aalong the circumference, in clockwise direction.Balong the axis, perpendicular to the plane.Calong anticlockwise direction.Dalong the equator in straight line. The circumference.
Draw the magnetic field due to a current-carrying circular coil. State the clock rule to find the polarities of the faces of the coil.
View SolutionCalculate the magnetic field induction at the centre of a square shaped coil of side 10 cm, if it carries of current of 1A.
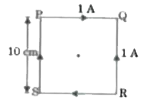
View SolutionThe magnetic field due to current carrying circular coil having n turns is
View SolutionThe magnetic induction at a point on the axis of a circular current carrying coil at a distance equal to the radius of coil carrying a current of 0.5 A is
View SolutionThe magnetic moment of a circular coil carrying current is
View Solution
