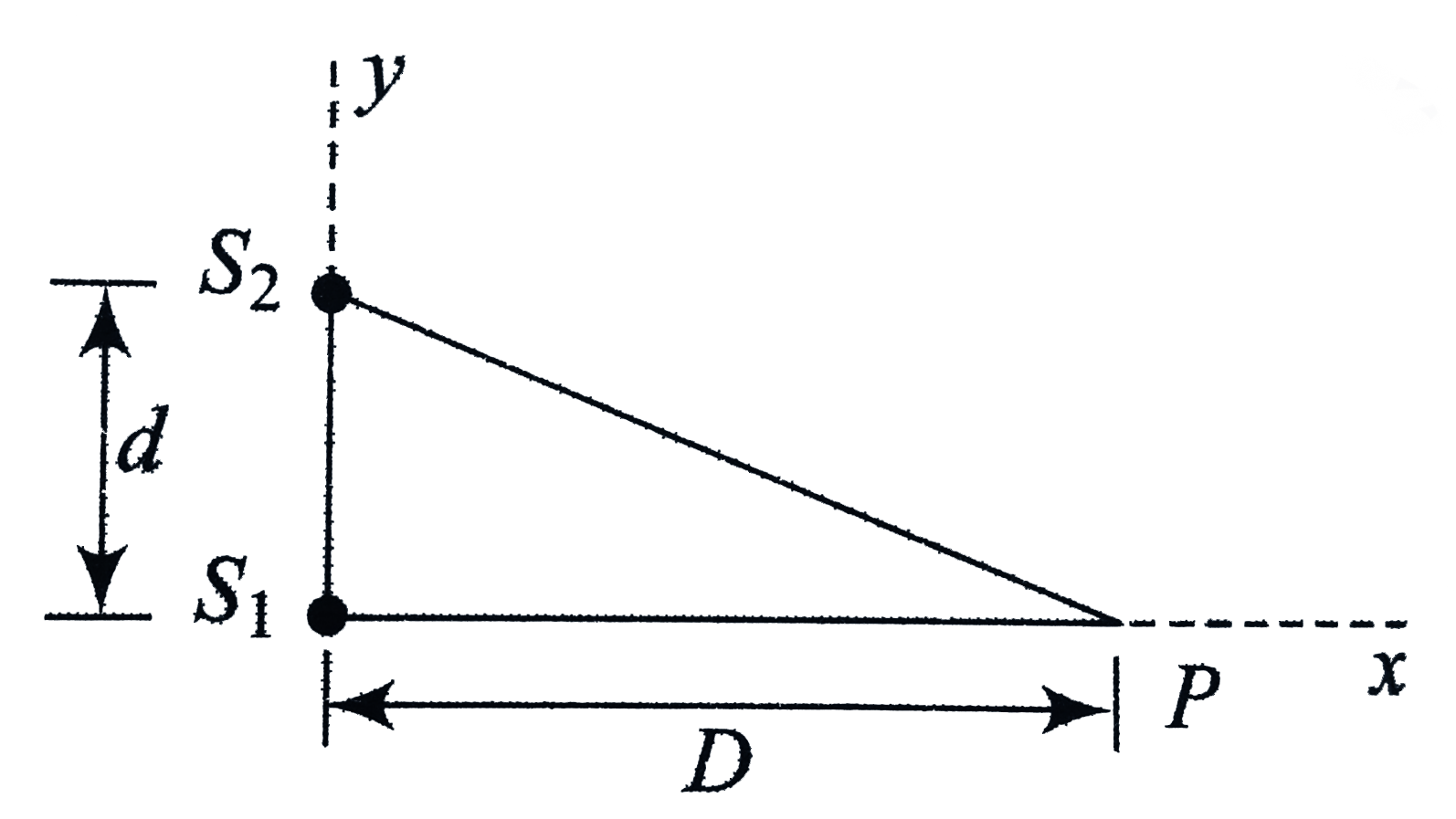
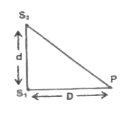
S1andS2 are two sources of light separated by a distance d. A detector can move along S2 P perpendicular to S1S2. What should be the minimum and maximum path difference at the detector?

Minimum path difference is zero (when p is at infinity
Maximum path difference =d
Topper's Solved these Questions
- ELECTROMAGNETIC WAVES AND OPTICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROMAGNETIC WAVES AND OPTICSEXERCISE - SHORT ANSWER QUESTIONS (3 Marks)
6 Videos
- ELECTROMAGNETIC WAVES AND OPTICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROMAGNETIC WAVES AND OPTICSEXERCISE - LONG ANSWER QUESTION
4 Videos
- ELECTROMAGNETIC WAVES AND OPTICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROMAGNETIC WAVES AND OPTICSEXERCISE - SHORT ANSWER QUESTION 1 MARK
5 Videos
- DUAL NATURE OF MATTER AND RADIATION & ATOMS AND NUCLEIBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - DUAL NATURE OF MATTER AND RADIATION & ATOMS AND NUCLEIEXERCISE - Long Answer Question
22 Videos
- ELECTRONIC DEVICES BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTRONIC DEVICES EXERCISE - NUMERICALS
7 Videos
Similar Questions
Two sources S1andS2 emitting light of wave length 600 nm are placed a distance 1.0x10−2 cm apart. A detector can be moved on the litre line S1P which is perpendicular to S1S2. a.What would be the minimum and maximum path differrence at the detector as it is moved alog the line S1P? b. Locate the position of the farthest minimum detect.
View SolutionTwo sources S1 and S2 emitting light of wavelength 600 nm placed a distance 1.0×10−2 cm apart.
A detector can be moved on line S1P which is perpendicular to S1S2
(a) What would be the minimum and maximum path difference at the detector as it moves along line S1P ?
(b) Locate the position of the farthest minima detected
 .
.
View Solution
Knowledge Check
S1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
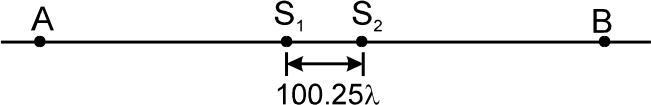 A∞B19C0D9
A∞B19C0D9Two coherent sources emit light of wavelength λ. Separation between them, d=4λ.
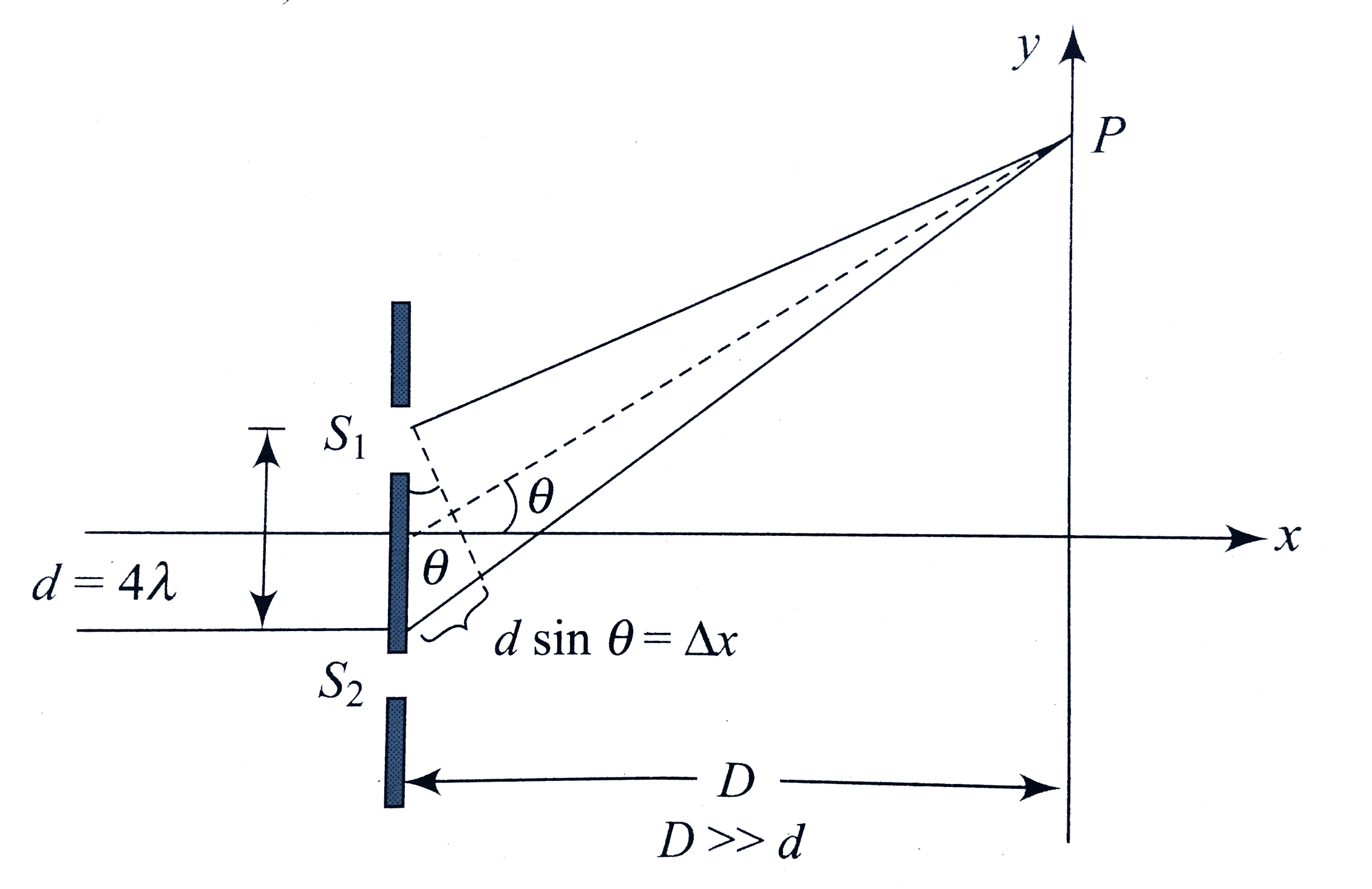
If a detector moves along the y-axis, what is the maximum number of minima observed?
If the detector moves along 'x-axis beginning from S2, the maximum number of minima observed isA2B3C5D4Consider two coherent, monochromatic (wavelength λ) sources S1andS2, separated by a distance d. The ratio of intensities of S1 and that of S2 (which is responsible for interference at point P, where detector is located) is 4. The distance of point P from S1 is (if the resultant intensity at point P is equal to 94 times of intensity of S1) (Given : ∠S2S1P=90∘,d>0andn is a positive integer)
Ad2−n2λ22nλBd2+n2λ22nλCnλd√d2−n2λ2D2nλd√d2−n2λ2
In the shown fig. S1 and S2 are two identical coher- ent sources of sound, separated by a distance d. A receiver moves along the line XY (which is parallel to line S1 S2) to detect the intensity of sound at various points on the line. Distance of line XY from line S1 S2 isD(>>d). Point O is the foot of perpendicular bisector of the line S1 S2 on XY. Distance of first intensity maxima (on line XY) measured from O is y. Find percentage change in value of y if the temperature of air increases by 1%.
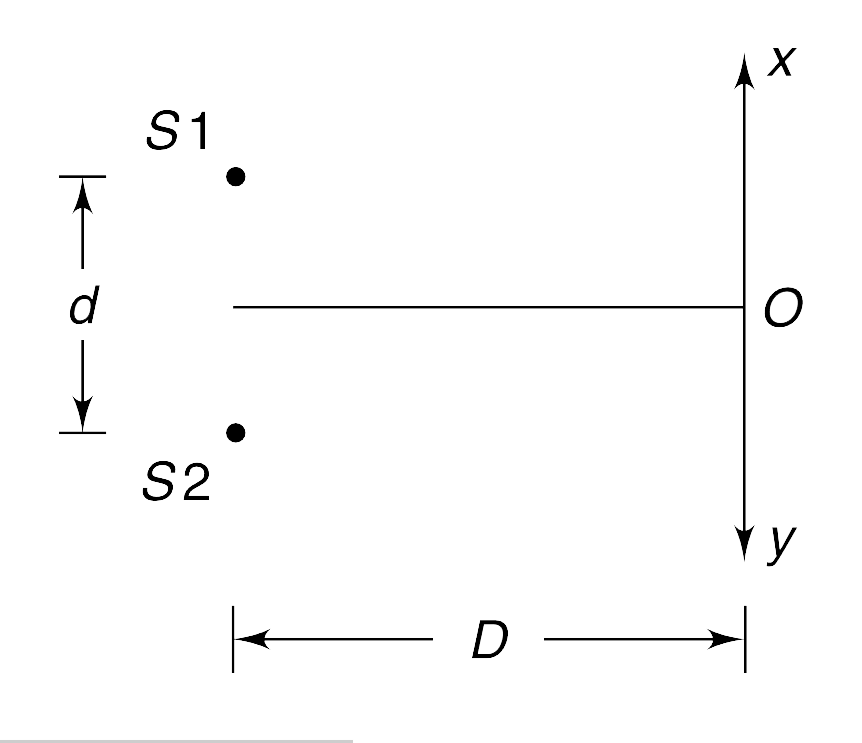
View SolutionTwo sources S1 and S2 emitting light of wave lengths 600 nm are placed a distance 1.0 x 102 cm apart..A detector can be moved on the line which is perpendicular to SiS2 . Find out the position of first.minimum detected.
View SolutionIn Fig. , two point sources S1 and S2 which are in phase and separated by distance D=1.5λ , emit identical sound waves of wavelength λ.
(a) What is the path length difference of the waves from S1 and S2 at point P1, which lies on the perpendicular bisector of distance D, at a distance greater than D from the sources ? (That is, what is the difference in the distance from source S1 to point P1 and the distance from source S2 to P1?) What type of interference occurs at P1 ?
(b) What are the path length difference and type of interference at point P2 in fig.
(c ) Figure shows a circle with a radius much greater than D, centered on the midpoint between sources S1 and S2. What is the number of points N around this circle at which the interference is fully constructive? (That is, at how many points do the waves arrive exactly in phase?)
View SolutionTwo sources of sound, S1andS2 , emitting waves of equal wavelength 20.0 cm, are placed with a separation of 20.0 cm between them. A detector can be moved on a line parallel to S_1S_2 and at a distance of 20.0 cm from P it. Initially, the detector is equidistant from the two sources. Assuming that the waves emitted by the sources are in phase, find the minimum distance through wffich the detector should be shifted to detect a minimum of sound.
View SolutionTwo source of sound, S1 and S2, emitting waves of equal wavelength 2cm, are placed with a separation of 3cm between them. A detector can be moved on a line parallel to S1S2 and at a distance 24cm from it. Initially, the detecor is equidistant from the two sources. Assuming that the waves emitted by the sources are in phase, find the minimum distance through which the detector should be shifted to detect a minimum of sound.
View Solution
