Find the standard deviation for the following data:
10, 20, 30, 40, 50, 60, 70, 80, 90
The correct Answer is:
To find the standard deviation of the given data set: 10, 20, 30, 40, 50, 60, 70, 80, 90, we will follow these steps:
Step 1: Calculate the Mean
The mean (average) is calculated by summing all the values and dividing by the number of values.
Mean(xˉ)=Sum of all valuesn
Where n is the number of values.
Sum=10+20+30+40+50+60+70+80+90=450
n=9
xˉ=4509=50
Step 2: Calculate the Deviations from the Mean
Next, we find the deviations of each value from the mean, and then square these deviations.
Deviations=(xi−xˉ)
10−50=−4020−50=−3030−50=−2040−50=−1050−50=060−50=1070−50=2080−50=3090−50=40
Now, squaring these deviations:
(−40)2=1600(−30)2=900(−20)2=400(−10)2=10002=0102=100202=400302=900402=1600
Step 3: Sum of Squared Deviations
Now, we sum the squared deviations:
Sum of squared deviations=1600+900+400+100+0+100+400+900+1600
Calculating this gives:
Sum=1600+900+400+100+0+100+400+900+1600=5000
Step 4: Calculate the Variance
The variance is calculated by dividing the sum of squared deviations by the number of values.
Variance(σ2)=Sum of squared deviationsn
σ2=50009≈555.56
Step 5: Calculate the Standard Deviation
The standard deviation is the square root of the variance.
σ=√σ2=√50009≈23.57
Final Answer
The standard deviation of the given data set is approximately 23.57.
---
The mean (average) is calculated by summing all the values and dividing by the number of values.
Next, we find the deviations of each value from the mean, and then square these deviations.
Now, we sum the squared deviations:
The variance is calculated by dividing the sum of squared deviations by the number of values.
The standard deviation is the square root of the variance.
The standard deviation of the given data set is approximately 23.57.
Topper's Solved these Questions
- STATISTICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATISTICSEXERCISE - Section - C (Long Answer Type-I Questions) (4 Mark)
10 Videos
- STATISTICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATISTICSEXERCISE - Section - D (Long Answer Type-II Questions) (6 Mark)
10 Videos
- STATISTICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATISTICSEXERCISE - Section - D (Long Answer Type-II Questions) (6 Mark)
10 Videos
- SETS AND FUNCTIONSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SETS AND FUNCTIONSEXERCISE - LONG ANSWER TYPE QUESTIONS
21 Videos
- STRAIGHT LINES BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STRAIGHT LINES EXERCISE - SECTION-D (LONG ANSWER TYPE QUESTIONS )
23 Videos
Similar Questions
Calculate the mean and the standard deviation for the following distribution : Marks [20,30,40,50,60,80,90],[,-,-,-,-,-,-,-],[,30,40,50,60,70,90,[10],[0]], No.of Student [s],3,6,13,15,14,5,4]
View SolutionFind the variance and standard deviation of the following data : 4,6,10,12,14,18,20
View Solution
Knowledge Check
Find the mode of the following data:
110,80,70,90, 120,90, 80,70,60,80A80B110C90D120What is the standard deviation of the following data ?
Measurement0−1010−2020−3030−40Frequency1342A81B7.6C9D2.26
Calculate the mean deviation (about mean) and standard deviation of the following data:
25,50,48,70,45,33,51,31,60
View SolutionUsing step deviation method find the standard deviation and its coeficient of the following data.Class 20−30,40−50,50−60,60−70,70−80,80−90 and Frequency 3,61,132,153,140,51,2
View SolutionCalculate the mean and standard deviation for the following distribution: Marks:, 20-30, 30-40, 40-50, 50-60, 60-70, 70-80, 80-90 No. of students:, 3, 6, 13, 15, 14, 5, 4
View SolutionCalculate the mean, variance and standard deviation for the following distribution :
View SolutionCalculate the median from the following data: Marks below: 10 20 30 40 50 60 70 80 No. of students: 15 35 60 84 96 127 198 250
View SolutionFind the mean deviation about median for the following data
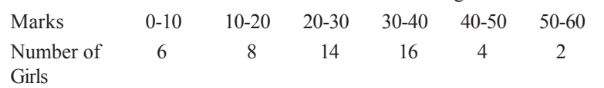
View Solution