Question

Draw a circle of radius 4 cm. Construct a pair of tangents to it, the angle between which is ${60}^{\circ }$. Also justify the construction. Measure the distance between the centre of the circle and the point of intersection of tangents.

Explanation

In order to draw the pair of tangents, we follow the following steps.
Steps of construction
1. Take a point O on the plane of the paper and draw a circle of radius OA=4 cm.
2. Produce OA to B such that OA=AB=4 cm.
3. Taking A as the centre draw a circle of radius AO=AB=4 cm.
Suppose it cuts the circle drawn in step 1 at P and Q.
4. Join BP and BQ to get desired tangents.
Justification In `DeltaOAP`, we have
OA=OP=4cm (`:.` Radius )
Also, AP=4cm (`:.` Radius of circle with centre A )
`:. DeltaOAP` is equilateral
`implies anglePAO=60^(@)`
`implies angleBAP=120^(@)`

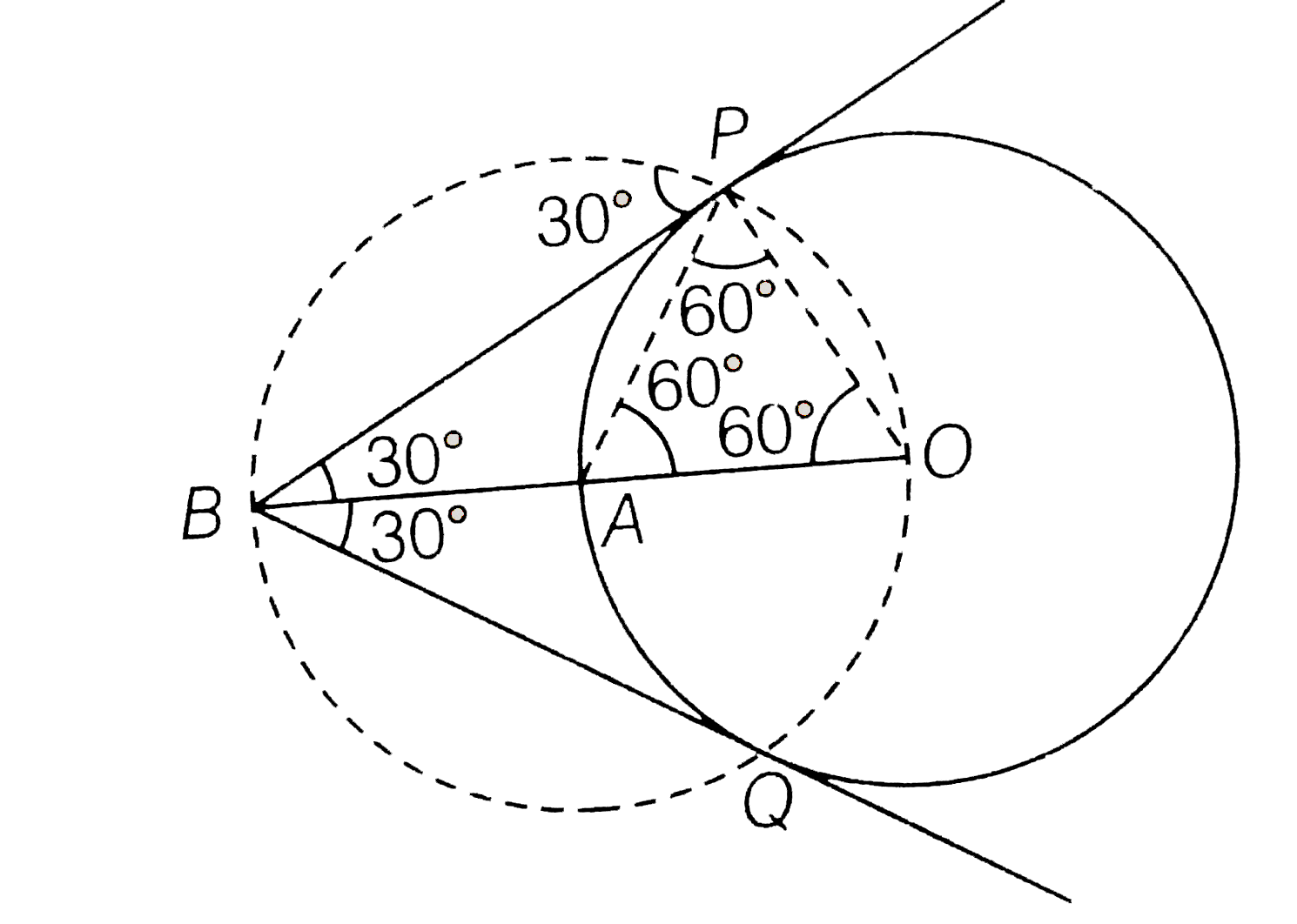
In `DeltaBAP`, we have
`BA=AP " and " angleBAP=120^(@)`
`: angleABP= angleAPB =30^(@)`
`implies anglePBQ=60^(@)`
Alternate method
Steps of construction
1. Take a point O on the plane of the paper and draw a circle with centre O and radius OA=4 cm.
2. At O construct radii OA and OB such that to `angleAOB` equal `120^(@)` i.e., supplement of the angle between the tangents.
3. Draw perpendicular to OA and OB at A and B, respectively. Suppose these perpendicular intersect at P. Then, PA and PB are required tangents.

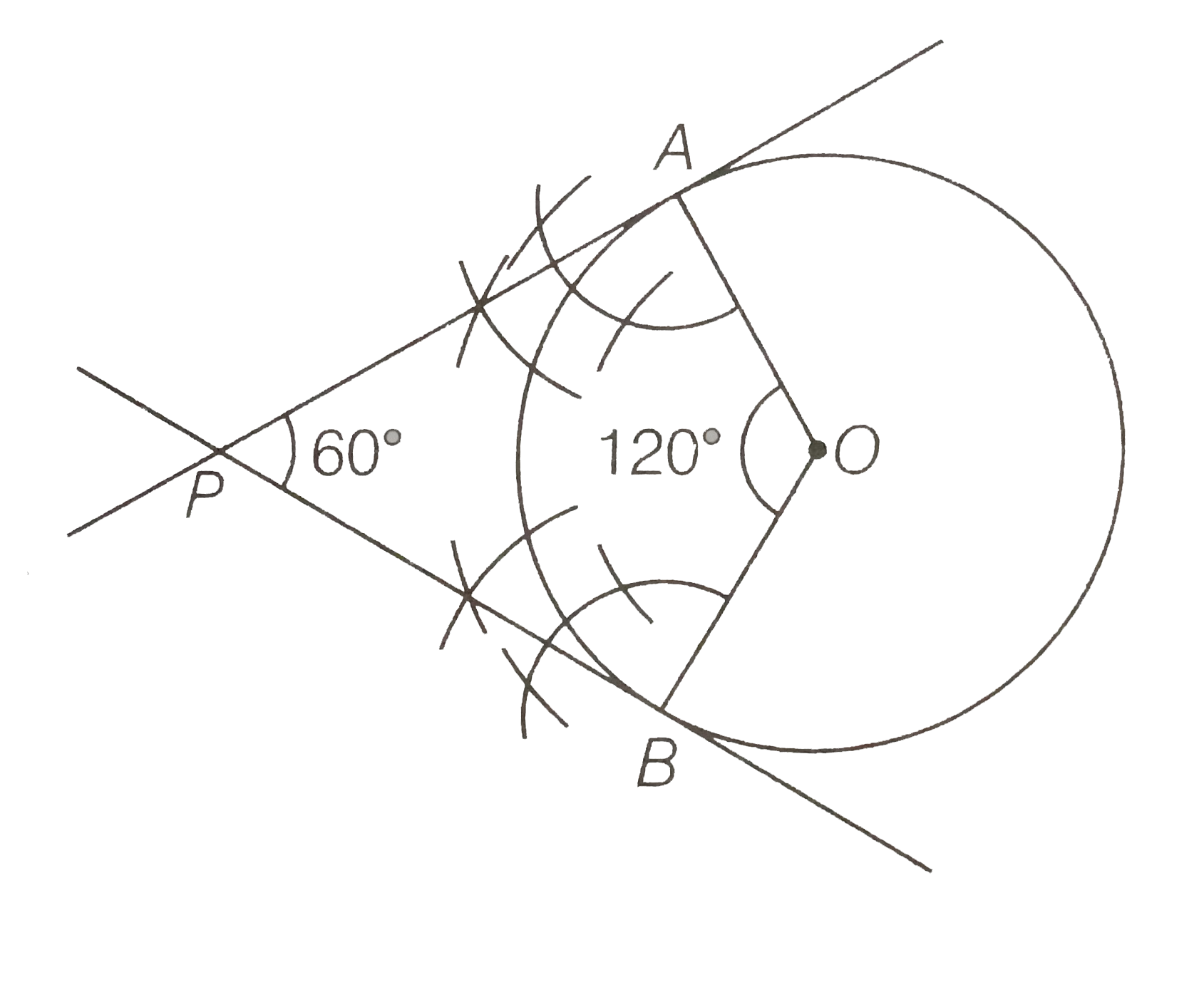
Justification
In quadrilateral OAPB, we have
`angleOAP=angleOBP=90^(@)`
and `angle AOB=120^(@)`
`:. angleOAP+angleOBP+angleAOB+angleAPB=360^(@)`
`implies 90^(@)+90^(@)+120^(@)+angleAPB=360^(@)`
`:. angle APB=360^(@)-(90^(@)+90^(@)+120^(@))`
`=360^(@)-300^(@)=60^(@)`