If a man standing on a platform 3 m above the surface of a lake observes a cloud and its reflection in the lake, then the angle of elevation of the cloud is equal to the angle of depression of its reflection.

Video Solution

Text Solution

Verified by Experts

False,
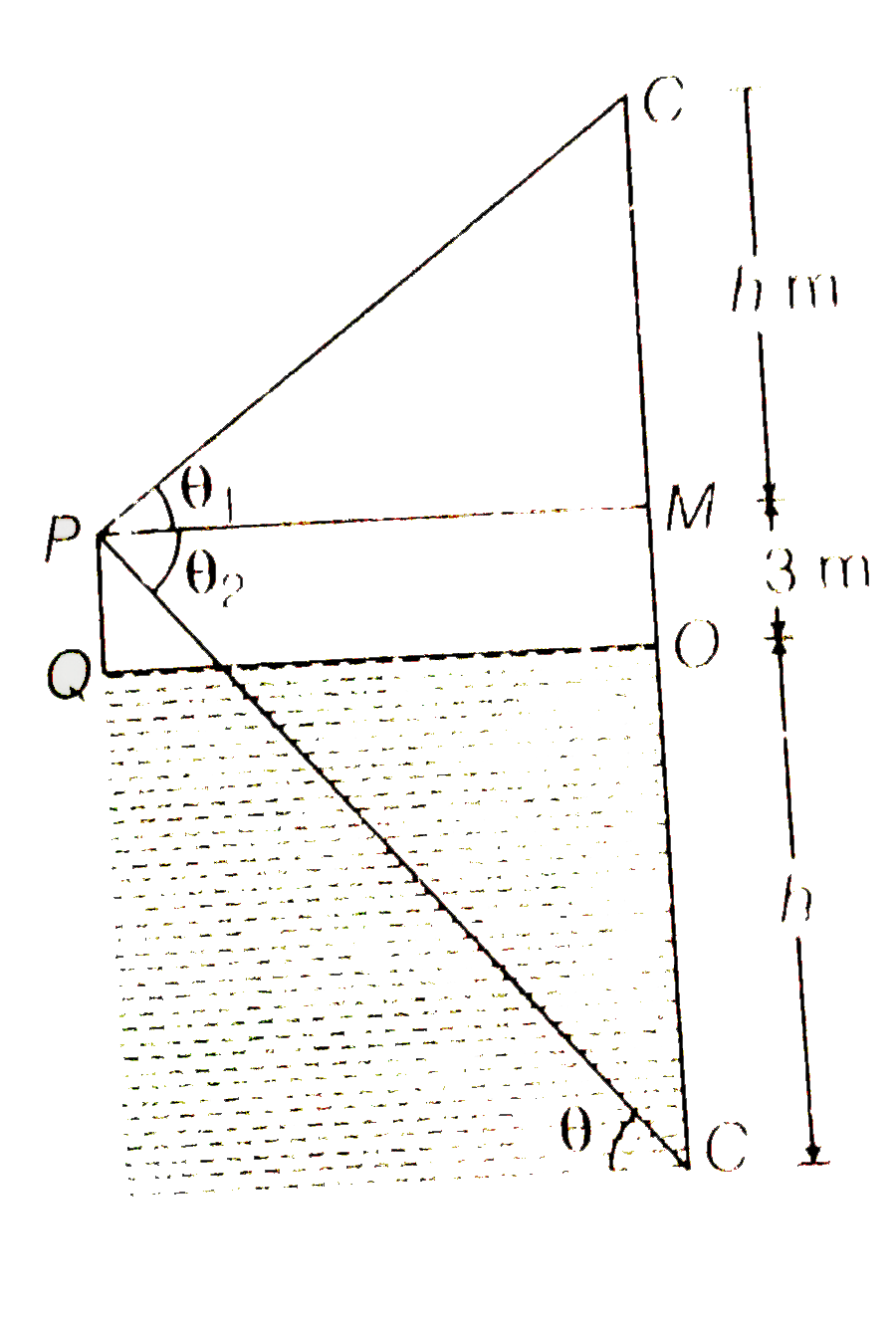
From figure, we observe that, a man standing on a platform at point P, 3 m above the surface of a lake observes a cloud at point C. Let the height of the cloud from the surface of the platfom is h and angle of elevatin of the cloud is $θ_{1}$ .
Now at same point P a man observes a cloud reflection in the lake at this time the height of reflection of cloud in lake is (h+3) because in lake platform height is also added to reflection of cloud.
So, angle of depression is different in the lake from the angle of elevatino of the cloud above the surface of a lake.
In $Δ M P C, {tan θ}_{1} = \frac{C M}{P M} = \frac{h}{P M}$
$\Rightarrow \frac{{tan θ}_{1}}{h} = \frac{1}{P M}$ ..................(i)
In $Δ C P M, {tan θ}_{2} = \frac{C M}{P M} = \frac{O C + O M}{P M} = \frac{h + 3}{P M}$
$\Rightarrow \frac{{tan θ}_{2}}{h + 3} = \frac{1}{P M}$ ...........(ii)
From eqs. (i) and (ii),
$\frac{{tan θ}_{1}}{h} = \frac{{tan θ}_{2}}{h + 3}$
$\Rightarrow {tan θ}_{2} = \frac{h + 3}{h} {tan θ}_{1}$
Hence, $θ_{1} \neq θ_{2}$

Alternate method:
False, we know that, if P is a point above the lake at a distance d, then the reflection of the point in the lake would be at the same distance d. Also, the angle of elevation and depression from the surface of the lake is same.
Here, the man is standing on a platform 3m above the surface, so its angle of elevation to the cloud and angle of depression to the reflection of the cloud is not same.

Updated on:21/07/2023

A helicopter is in a stationary position at a certain height over the lake. At a point 200 m above the surface of the lake, the angle of elevation of the helicopter is $45^{\circ}$ . At the same time, the angle of depression of its reflection in the lake is $75^{\circ}$ . Calculate the height of the helicopter from the surface of the lake :
A $\frac{200}{\sqrt{3}}$
B $200 \sqrt{3} m$
C200 m
D150 m
The angle of elevation of a cloud from a point h m above the level of water in a lake is $α$ and the angle of depression of its reflection in the lake is $β$ . Then the height of the cloud above the water level is
A $\frac{h sin (β - α)}{sin (β + α)}$
B $\frac{h sin (β + α)}{sin (β - α)}$
C $\frac{h sin (α + β)}{sin (α + β)}$
DNone
If the angle of elevation of a cloud from a point 200m above a lake is $30^{\circ}$ and the angle of depression of its reflection in the lake is $60^{\circ}$ .Then the height of the cloud above the lake is :
A100 m
B200 m
C300 m
D400 m