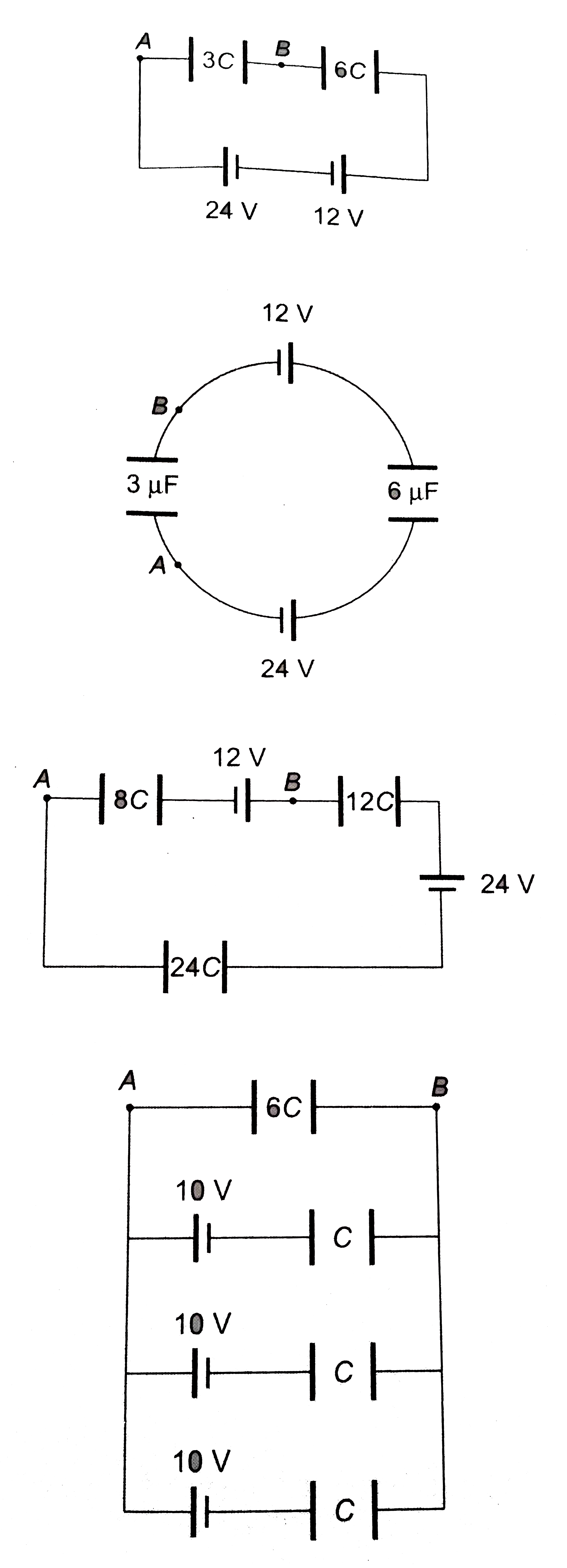
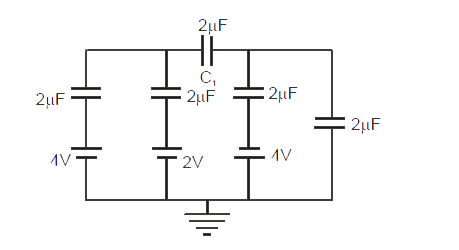
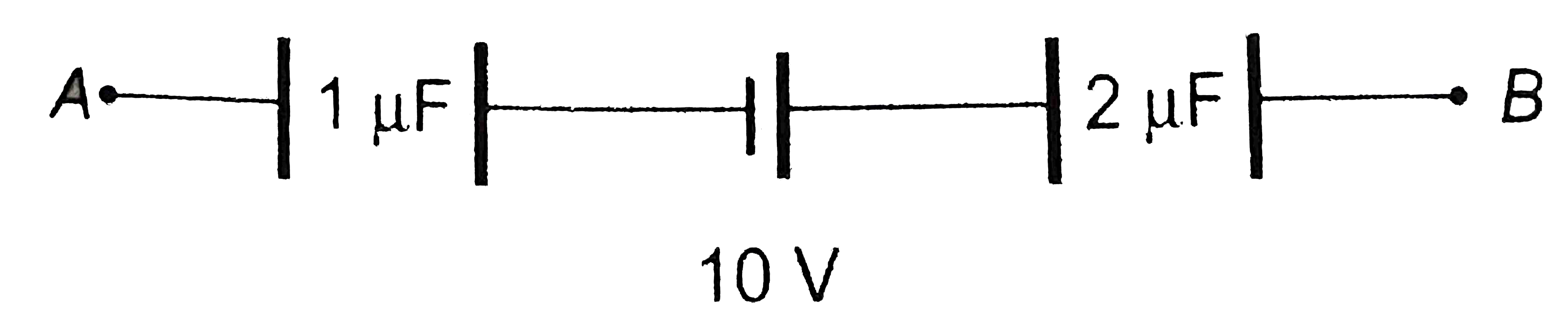 Find voltage across capacitor C1.
Find voltage across capacitor C1.
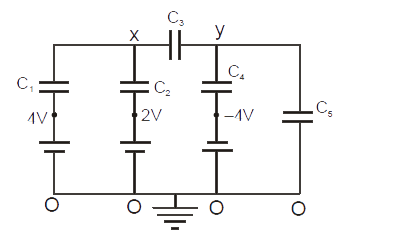
Now from charge conservation at anode x and y for x (x−4)C1+(x−2)C2+(x−y)C3=0
⇒2(x−4)+2(x−2)+(x−y)2=0
6x−2y−12=0...........(1)
For y (y−x)C3+[y−(−4)]C4+(y−0)C5=0
⇒(y−x)2+(y+4)2+y2=0
=6y−2x+8=0.............(2)
eq. (1) & (2)
y=-3/4 x=7/4
So potential difference =x−y=74−(−34)=52volt
Topper's Solved these Questions
Similar Questions
Knowledge Check
R1=R2=R3=R4=2ohm. Find voltage across capacitor at steady state
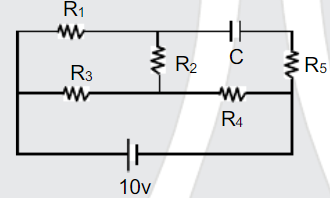 A0VB2VC4VD8V
A0VB2VC4VD8VIn the circuit shown in the figure, the total charge is 750μC and the voltage across capacitor C2 is 20 V. Then the charge on capacitor C2 is:
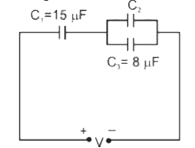 A650μCB450μCC590μCD160μC
A650μCB450μCC590μCD160μCIn the given circuit the R.M.S values of voltages across the capacitor C, inductor L and resistor R1 are 12V, 10V and 5 V respectively. Then the peak voltage across R2 is
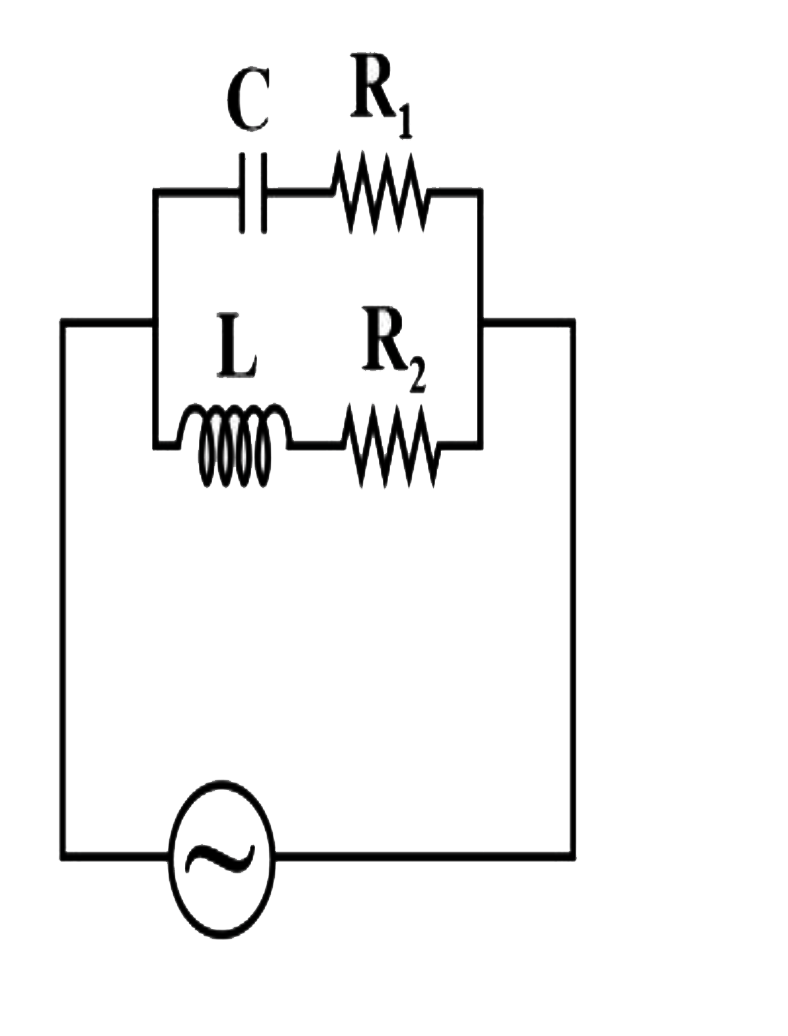 A7√2VB√69VC√138VDnone of these
A7√2VB√69VC√138VDnone of these
Find how the voltage across the capacitor C varies with time t after closing of the switch Sw at the moment t=0.
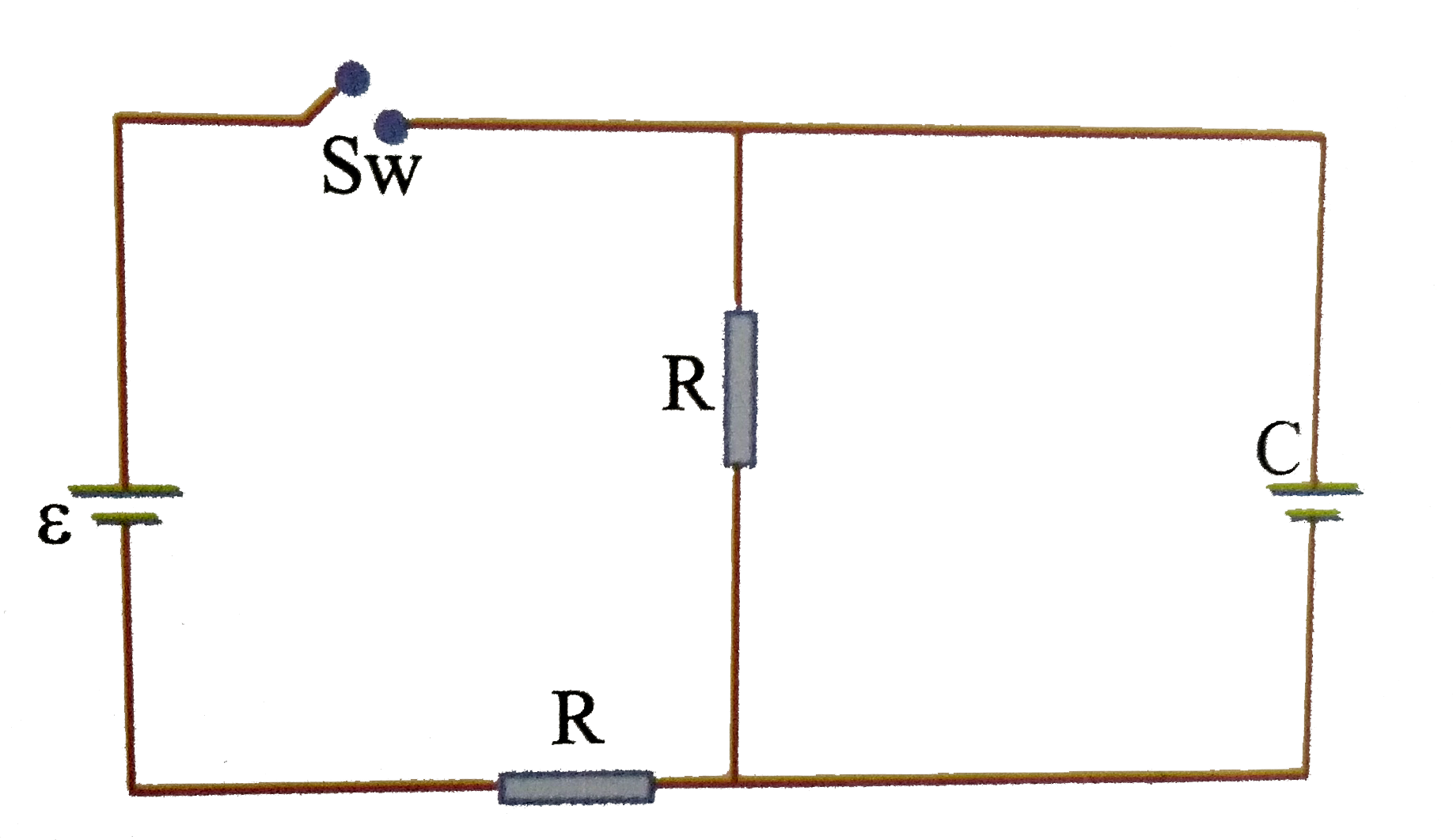 .
.
View SolutionThe voltage applied across a capacitor having a capacitance of 10μF is varies as shown in figure:
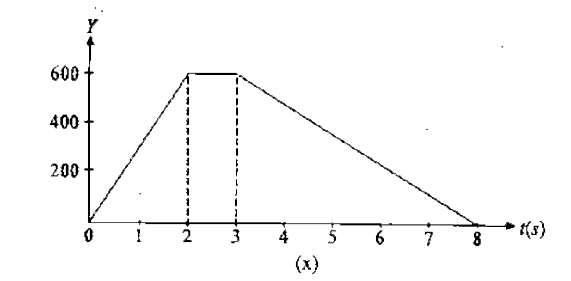
At the time instant when the terminal voltage across capacitor is 600V calculate
(a) The charge on capacitor ltBrgt (b) The energy stored in the capacitor
(c) Draw the curve of current in connecting wires as a function of time.
View SolutionA circuit has section AB as shown in figure. The emf of the source equals E=10V, the capacitor capacitances are equal to C1=1.0μF and C2=2.0μF, and the potential difference VA−VB=5.0V. Find the voltage across each capacitor.
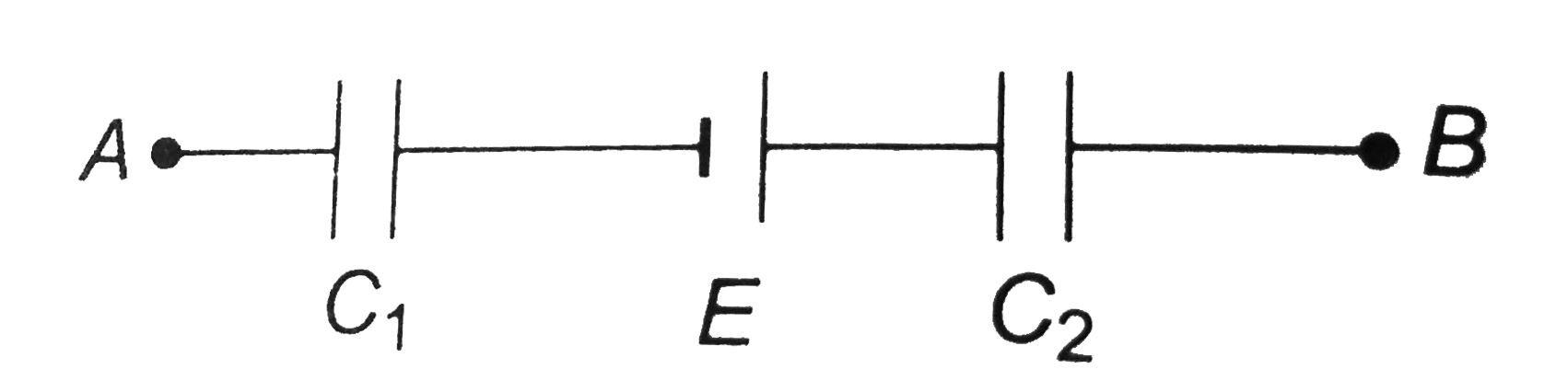
View SolutionA series R−C circuit is connected to an alternating voltage source. Consider two situations
(a) When capacitor is air filled.
(b) When capacitor is mica filled.
current through resistor is i and voltage across capacitor is V then
View SolutionA series R−C circuit is connected to an alternating voltage source. Consider two situations
(a) When capacitor is air filled.
(b) When capacitor is mica filled.
current through resistor is i and voltage across capacitor is V then
View Solution