Find the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is λ
−→dE=dExˆi+dEyˆj on solving Enet=KλR=(ˆi+ˆj),
Electric field due to ring on its axis :
Enet=KQx[R2+x2]3/2
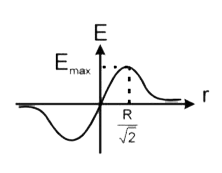
E will be max when dEdx=0, that is at x=R√2 and Emax=2KQ3√3R2
Case (i) : if x>>R,E=KQx2 Hence the ring will act like a point charge
Case (ii) : if x<<R,E=KQxR3
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - SELF MISCELLANEOUS PROBLEM (Example )
15 Videos
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - SELF PRACTICE PROBLEM
27 Videos
- ELECTRONICS - SEMI CONDUCTORBOOK - MOTIONCHAPTER - ELECTRONICS - SEMI CONDUCTOREXERCISE - EXERCISE - 3
29 Videos
- ELECTROSTATICS - I BOOK - MOTIONCHAPTER - ELECTROSTATICS - I EXERCISE - EXERCISE - 4 (Level -II) PREVIOUS YEAR - JEE ADVANCED
13 Videos
Similar Questions
Knowledge Check
Electric field at a point of distance r from a uniformly charged wire of infinite length having linear charge density λ is directly proportional to
Ar−1BrCr2Dr−2The electric field at the centre of a uniformly charged ring is zero. What is the electric field and potential at the centre of a half ring if the charge on it be Q and its radius be R?
A14πε0QπR2,14πε0QπRB14πε0QR2,14πε02QπRC14πε02QπR2,14πε0QRD14πε02QR2,14πε03QπR
In the given arrangement find the electric field at C in the figure. Here the U-shaped wire is uniformly charged with linear charge density λ. [0]
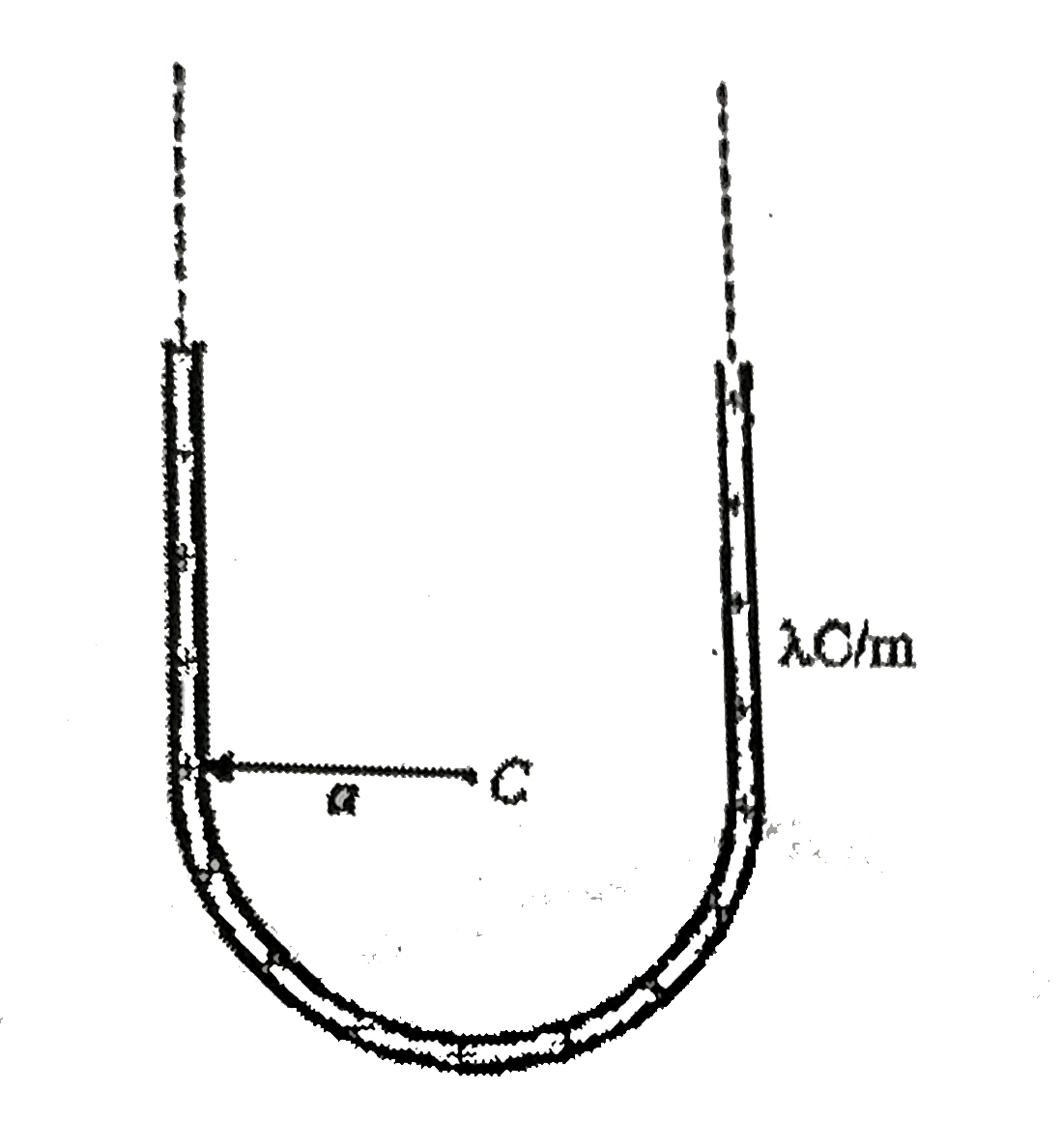
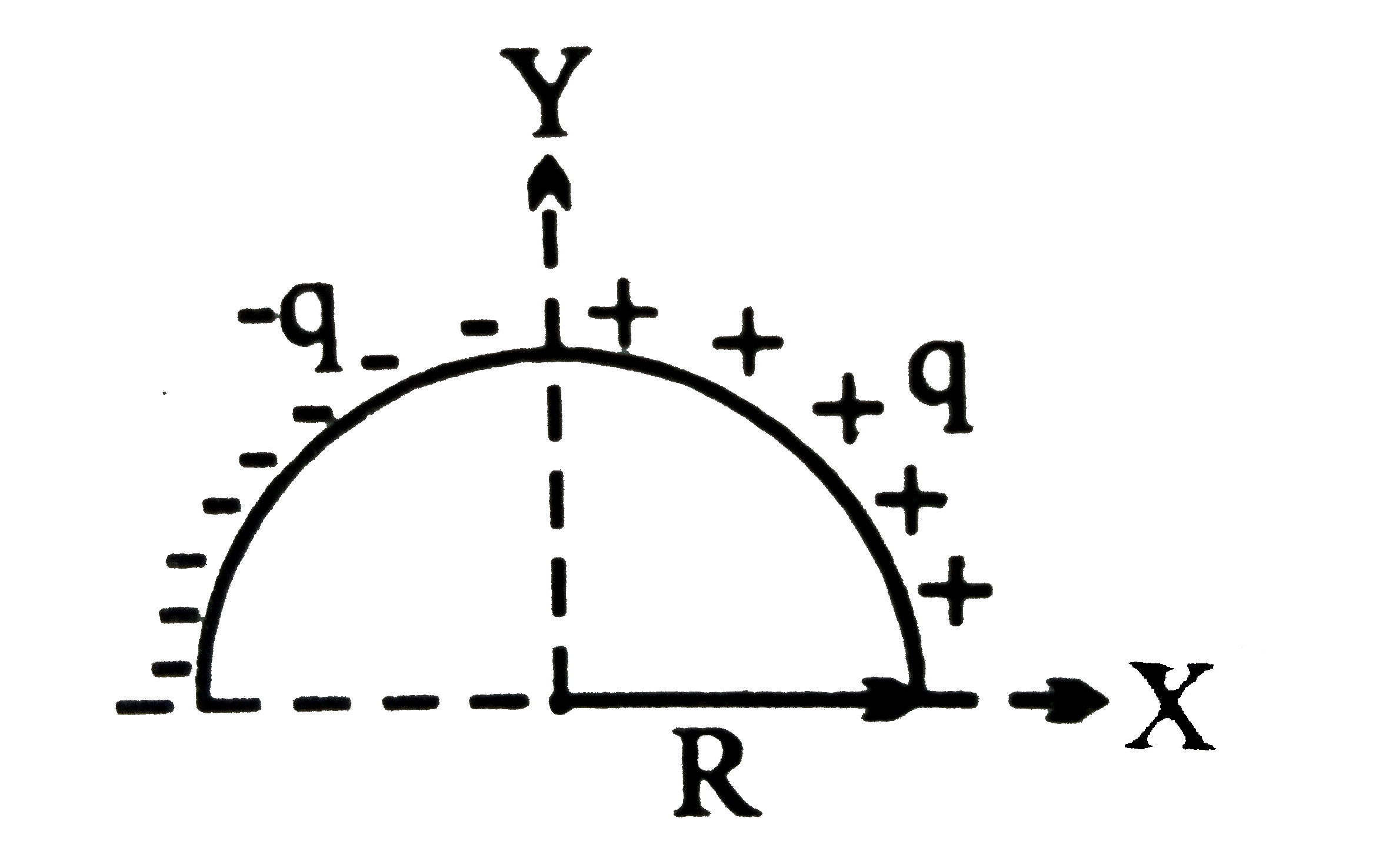
View SolutionElectric field at centre O of semicircule of radius 'a' having linear charge density λ given is given by

View SolutionElectric field at the centre of uniformly charge hemispherical shell of surface charge density σ is σnε0 then find the value of n.

View SolutionIf coulomb's law were F=KqQr3, calculate the electric field due to a uniformly charged line charge at a distance d from it. The linear charge density on the line charge is λ C/m, and it is of infinite length.
View SolutionDraw the electric field vs distance (from the centre ) graph for (i) a long charged rod having linear charge density λ>0 (ii) spherical shell of radius R and charge Q>0 .
View SolutionFind the electric field due to an infinitely long cylindrical charge distribution of radius R and having linear charge density λ at a distance half of the radius from its axis.
View Solution


 .
.