Figure shows a square loop carrying current I is present in the magnetic field which is given by →B=B0zLˆj+B0yLˆk where B0 is positive constant. Which of the following statement(s) is/are correct?
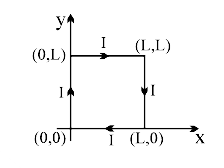
force on side (0,0) to (0,L) is (B0IL2)ˆi
force on side (0,L) to (L,L) is −B0ILˆj
Net magnetic force on loop is zero
force on side (L,0) to (0,0) is zero
The correct Answer is:A, B, D
Step by step video, text & image solution for Figure shows a square loop carrying current I is present in the magnetic field which is given by vecB=(B_(0)z)/(L)hatj+(B_(0)y)/(L)hatk where B_(0) is positive constant. Which of the following statement(s) is/are correct? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MAGNETISM Book:MOTIONChapter:MAGNETISM Exercise:EXERCISE-3 (Level-I)
Explore 46 Videos
- MAGNETISM Book:MOTIONChapter:MAGNETISM Exercise:EXERCISE-3 (Level-II)
Explore 23 Videos
- MAGNETISM Book:MOTIONChapter:MAGNETISM Exercise:EXERCISE-2 (Level-I)
Explore 40 Videos
- LOGIC GATESBook:MOTIONChapter:LOGIC GATESExercise:EXERCISE - 2
Explore 10 Videos
- MAGNETISM -1Book:MOTIONChapter:MAGNETISM -1Exercise:Exercise - 3 SECTION -A
Explore 56 Videos
Similar Questions
The figure shows a loop of wire carrying a current i as shown. There exists a uniform magnetic field along x-axis given by →B=B0ˆi. If the length of each side is a, then
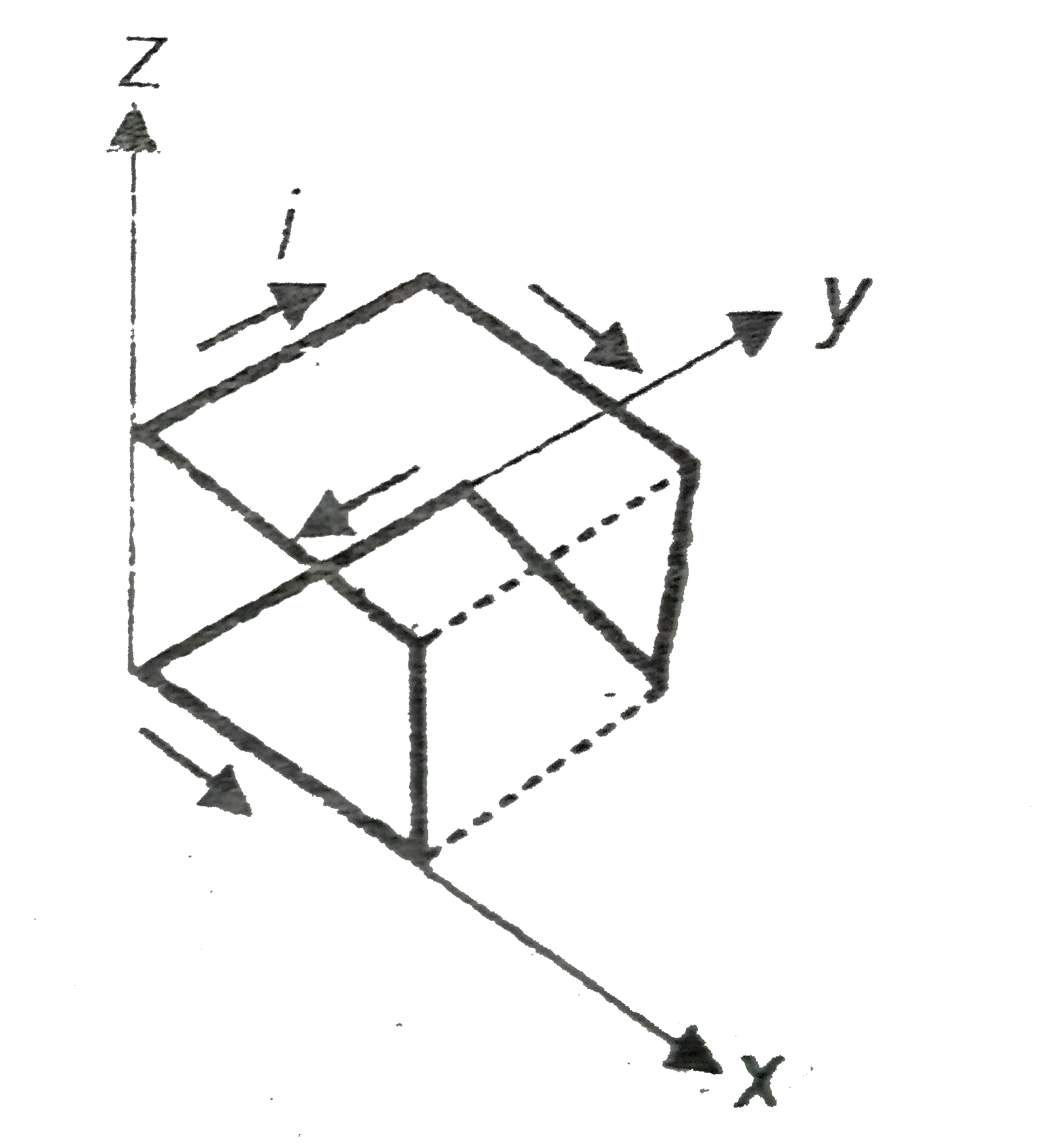
View SolutionA positive charge particle to charge q& mass m is released at origin.There are unifrom magnetic and electric field in the space given by →E=E0ˆj&→B=B0ˆk, where E0&B0 are constants.Find the y coordinate of the particle at time t.
View Solution
Knowledge Check
Given a+b+c+d=0. Which of the following statements(s) is (are) correct?
Aa,b,c, and d much each be a null vectorBThe magnitude of a+c equals the magnitude of b+dCThe magnitude of a can never be greater than the sum of the magnitudes of b,c and dDb+c must lie in the plane of a and d if a and d are not colinear and in the line of a and d if they are collinearFigure shows sqare current caarrying coil of edge lengthL. The magnetic field on the coil is given by →B=B0yLˆi+B0xLˆj where B0 is a positive constant.
 AIf coil is free to rotate about x axis torque on the coil is given by 12iAB0ˆiBIf coil is free to rotate about y-axis torque on coil is given by −12iAB0ˆjCResultant force on coil is zero.DEquation for the torque →μ×→B where mu is magnetic moment of coil is not valid on the coil.
AIf coil is free to rotate about x axis torque on the coil is given by 12iAB0ˆiBIf coil is free to rotate about y-axis torque on coil is given by −12iAB0ˆjCResultant force on coil is zero.DEquation for the torque →μ×→B where mu is magnetic moment of coil is not valid on the coil.A square of side x m lies in the x-y plane in a region, when the magnetic field is given by →B=B0(3ˆi+4ˆj+5ˆk) T, where B0 is constant. The magnitude of flux passing through the square is
A5B0x2WbB3B0x3WbC2B0x2WbDB0x2Wb
A square loop EFGH of side a, mass m and total resistance R is falling under gravity in a region of transverse non - uniform magnetic field given by B=By0a =, where B0 is a positive constant and y is the position of side EF of the loop. If at some instant the speed of the loop is v, then the total Lorentz acting on the loop is
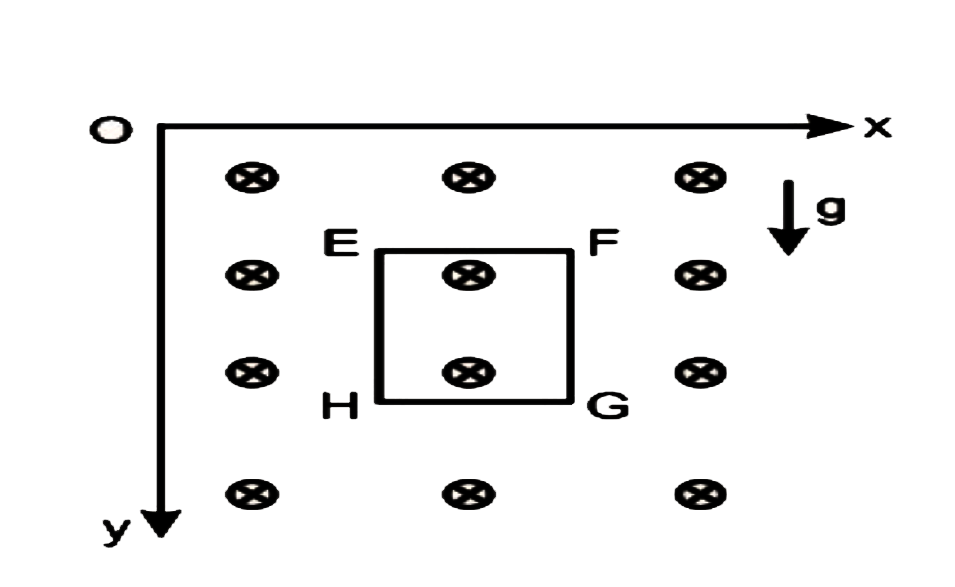
View SolutionA circular loop of radius R carrying current I is kept in XZ plane. A uniform and constant magnetic field →B=(B0ˆi+2B0ˆj+3B0ˆk) exists in the region (B0− a positive constant). Then the magnitude of the torque acting on the loop will be
View SolutionA square of side L metre lies in the x - y plane in a region, where the magnetic field is given by →B=B0(2ˆi+3ˆj+4ˆk)T, where B0, is constant. The magnitude of flux passing through the square is [Exemplar Problem]
View SolutionA square of side L meters lies in the x-y plane in a region, where the magnetic field is give by B=B0(2ˆi+3ˆj+4ˆk)T, where B0 is constant. The magnitude of flux passing through the square is
View SolutionWhen current (I) in R-L series circuit becomes constant, where L is a pure inductor, which of the following given statements is/are correct?

View Solution