The circumcentre of the $Δ A B C is O. Prove that ∠ O B C + ∠ B A C = 90^{\circ}$ .

Video Solution

Text Solution

Verified by Experts

Given A circle is circumscribed on a $Δ A B C$ having centre O.

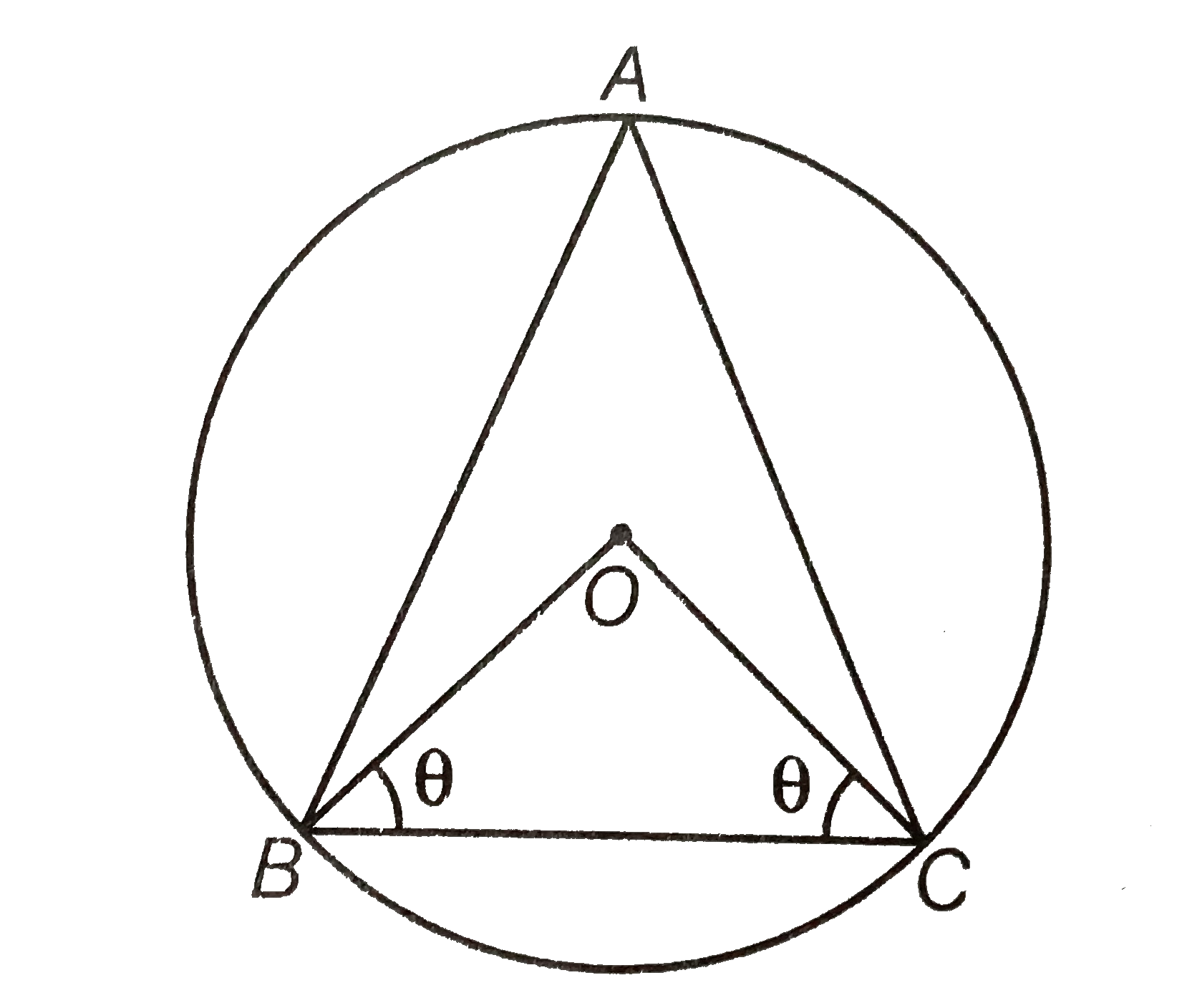
To prove $∠ O B C + ∠ B A C = 90^{\circ}$
Construction Join BO and CO.
Proof Let $∠ O B C = ∠ O C B = θ$ …(i)
In $Δ O B C, ∠ B O C + ∠ O C B + ∠ C B O = 180^{\circ}$
[by angle sum property of a triangle is $180^{\circ}$ ]
$\Rightarrow ∠ B O C + θ + θ = 180^{\circ}$
$\Rightarrow ∠ B O C = 180^{\circ} - 2 θ$
We know that, in a circle, the angle subtended by an arc at the centre is twice the angle subtended by it at the remaining part of the circle.
$∴ ∠ B O C = 2 ∠ B A C$
$\Rightarrow ∠ B A C = \frac{∠ B O C}{2} = \frac{180^{\circ} - 2 θ}{2} = 90^{\circ} - θ$
$\Rightarrow ∠ B A C + θ = 90^{\circ}$
$∴ ∠ B A C + ∠ O B C = 90^{\circ}$ [from Eq. (i)]

Updated on:21/07/2023

O is the circumcentre of $Δ A B C$ , given $∠ B A C = 85^{\circ} and ∠ B C A = 55^{\circ}$ , find $∠ O A C$ .
A $40^{\circ}$
B $50^{\circ}$
C $60^{\circ}$
D $80^{\circ}$
O is the circumcentre of the isosceles $Δ A B C$ . Given that AB = AC = 17 cm and BC = 6 cm. The radius of the circle is
A3.015 cm.
B3.205 cm.
C3.025 cm.
D3.125 cm.
O is the circumcentre of a triangle ABC. If $∠ B A C = 85^{\circ} and ∠ B C A = 75^{\circ}$ then what is the value of $∠ O A C$ ?
A $40^{\circ}$
B $60^{\circ}$
C $70^{\circ}$
D $90^{\circ}$