Three immiscible liquids of densities d1>d2>d3 and refractive indices μ1>μ2>μ3 are put in a beaker. The height of each liquid column is h3. A dot is made at the bottom of the beaker. For near normal vision, find the apparent depth of the dot.
Let the apparent depth be O1 for the object seen form m2 , then
O1=μ2μ1h3
Since, apparent depth =real depth /refractive index μ.
Since , the image formed by Medium 1, O2 act as an obeject for Medium 2
If seen from μ3, the apparent depth is O2.
Simiarly, the image formed by Mediuam 2, O2, act as an object for Medium 3
O2=μ3μ2(h3+O1)
=μ3μ2(h3+μ2μ1h3)=h3(μ3μ2+μ3μ1)
Seen from outside , the apparent height is
O3=1μ3(h3+O2)=1μ3[h3+h3(μ3μ2+μ3μ1)]
=h3(1μ1+1μ2+1μ3)
This is the required expression of apparent depth.
Topper's Solved these Questions
- NUCLEIBOOK - NCERT EXEMPLARCHAPTER - NUCLEIEXERCISE - Nuclei
40 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITBOOK - NCERT EXEMPLARCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITEXERCISE - Semiconductor Electronics: Material,Devices And Simple Circuit
26 Videos
Similar Questions
Three immiscible liquids of densities d1>d2>d3 and refractive indices μ1>μ2>μ3 are put in a beaker. The height of each liquid column is h3. A dot is made at the bottom of the beaker. For near normal vision, find the apparent depth of the dot.
View SolutionTwo immiscible liquids of densities d and 2d are taken in a cylindrical vessel with equal heights each h. The velocity efflux of the liquid coming out of the hole made to the wall of the vessel at its bottom is
View Solution
Knowledge Check
There are three optical media 1,2 and 3 with the refractive indices μ1>μ2>μ3.
(TIR → total internal reflection)Awhen a ray of light travels from 3 to 1 no TIR will placeBcritical angle between 1 and 2 is less than the critical angle between 1 and 3Ccritical angle between 1 and 2 is more than the critical angle between 1 and 3Dchances of TIR are move when ray of light travels from 1 to 3 as compared to the case when it travel from 1 to 2Two non-mixing liquids of densities ρ and (n>1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats with its axis vertical and length pL(p<1) in the denser liquid. The density d is equal to :
A{1+(n+1)p}ρB{2+(n+1)p}ρC{2+(n−1)p}ρD{1+(n−1)p}ρTwo non-mixing liquids of densities ρ and (n>1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats with its axis vertical and length pL(p<1) in the denser liquid. The density d is equal to :
A{1+(n+1)p}ρB{2+(n+1)p}ρC{2+(n−1)p}ρD{1+(n−1)p}ρ
Path of ray of light passing through three liquids of refractive indices μ1,μ2,μ3 is as shown in Fig. Which liquid has the smallest index of refraction ?
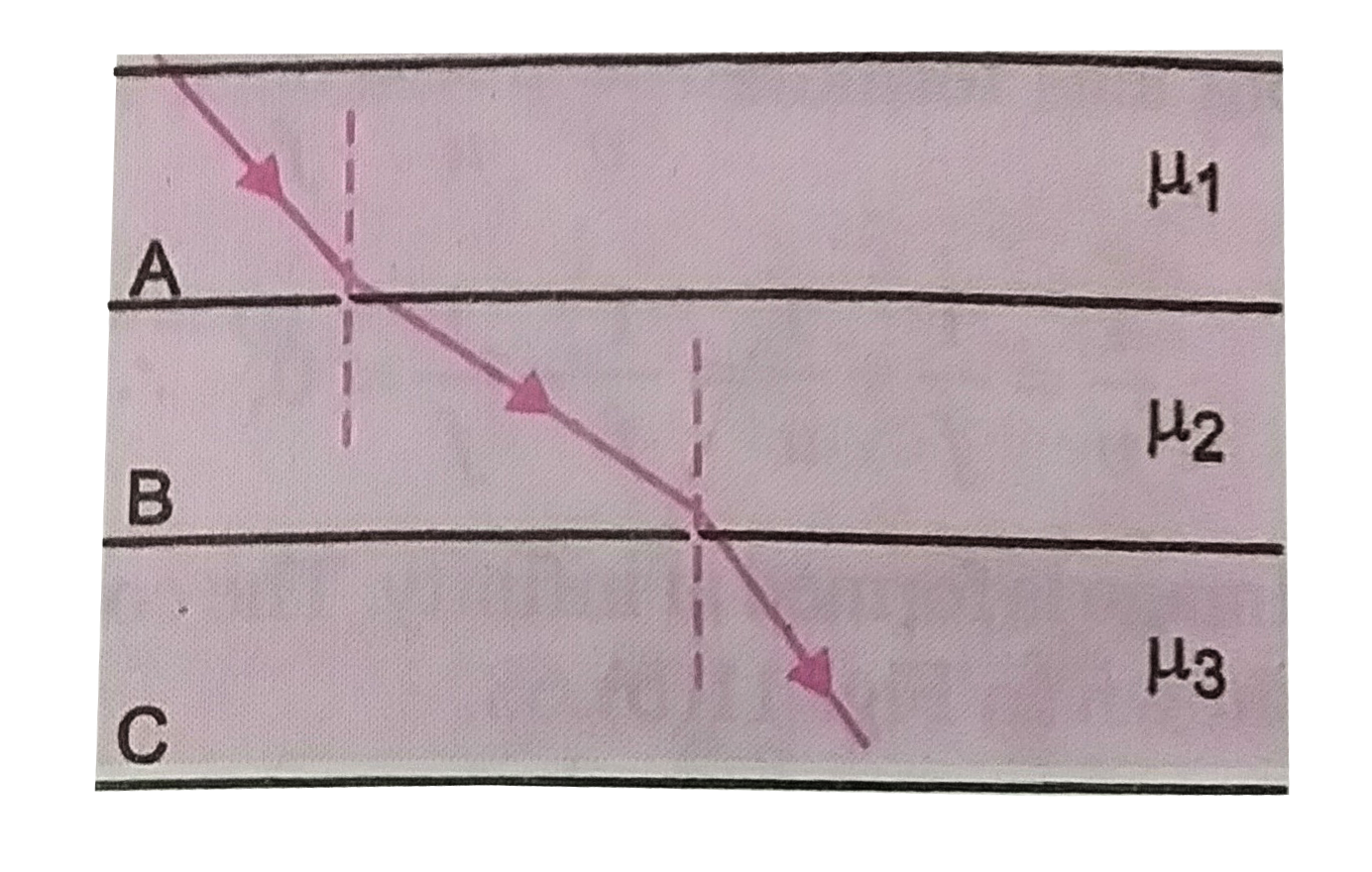 .
.
View SolutionThree immiscible transparent liquids with refractive indices 3/2,4/3 and 6/5 are arranged one on top of another. The depth of the liquid are 3cm,4cm and 6cm respectively. The apparent depth of the vessel is
View SolutionA beaker is filled with two immiscible transparent liquids of refractive indices μ1 and μ2 having thickness of layers d1 and d2 respectively. The apparent depth of the bottom of the beaker is
View SolutionThe length of the optical path of two media in contact of length d1 and d2 of refractive indices μ1 and μ2 respectively, is
View SolutionThe length of the optical path of two media in contact of length d1 and d2 of refractive indices μ1 and μ2 respectively, is
View Solution
