In the above question, the angular velocity will:
increase
decrease
not change
nothing can be said
The correct Answer is:B
To solve the problem regarding the angular velocity when an object sticks to the rim of a rotating body, we can follow these steps:
1. Understand the System: We have a rotating object with an initial angular velocity ω and a moment of inertia I=mr2, where m is the mass of the rotating object and r is its radius.
2. Moment of Inertia Before Collision: The initial moment of inertia of the rotating object is given by:
I=mr2
3. Moment of Inertia After Collision: When an additional mass m sticks to the rim of the rotating object, the new moment of inertia I′ becomes:
I′=mr2+m⋅r2=(m+m)r2=2mr2
4. Conservation of Angular Momentum: Angular momentum before and after the collision must be conserved. The initial angular momentum Li is given by:
Li=I⋅ω=mr2⋅ω
The final angular momentum Lf after the mass sticks is:
Lf=I′⋅ω′=2mr2⋅ω′
5. Setting Up the Equation: According to the conservation of angular momentum:
Li=Lf
Substituting the expressions for angular momentum:
mr2⋅ω=2mr2⋅ω′
6. Solving for Final Angular Velocity: We can simplify the equation by dividing both sides by mr2 (assuming m≠0 and r≠0):
ω=2ω′
Rearranging gives us:
ω′=ω2
7. Conclusion: The angular velocity after the mass sticks to the rim will be half of the initial angular velocity:
ω′=ω2
Final Answer:
The angular velocity will decrease to half of its initial value.
I=mr2
I′=mr2+m⋅r2=(m+m)r2=2mr2
Li=I⋅ω=mr2⋅ω
The final angular momentum Lf after the mass sticks is:
Lf=I′⋅ω′=2mr2⋅ω′
Li=Lf
Substituting the expressions for angular momentum:
mr2⋅ω=2mr2⋅ω′
ω=2ω′
Rearranging gives us:
ω′=ω2
ω′=ω2
The angular velocity will decrease to half of its initial value.
Similar Questions
Knowledge Check
In the above question, if the angular velocity is kept same but the radius of the path is halved, the new force will be
A2FBF2CF/2DF/4In the above question, the velocity
Aincreases between points O and ABincreases between points A and BCdecreases between points A and BDis zero throughoutIn the above question, the velocity of end O when end A hits the gournd is
AzeroBalong the horizontalCalong the verticalDat some inclination of the ground (≠90∘)
In the above problem the angular velocity of the system after the particle sticks to it will be?
View SolutionIn the above question, the value of maximum velocity of the particle will be :
View SolutionIn the above question, the velocity of end A at the instant it hits the ground is
View SolutionA disc of mass M and radius R can rotate freely in a vertical plane about a horizontal axis at O distance r from the centre of the disc as shown in Fig. The disc is released from rest in the shown position. Answer the following questions based on the above information
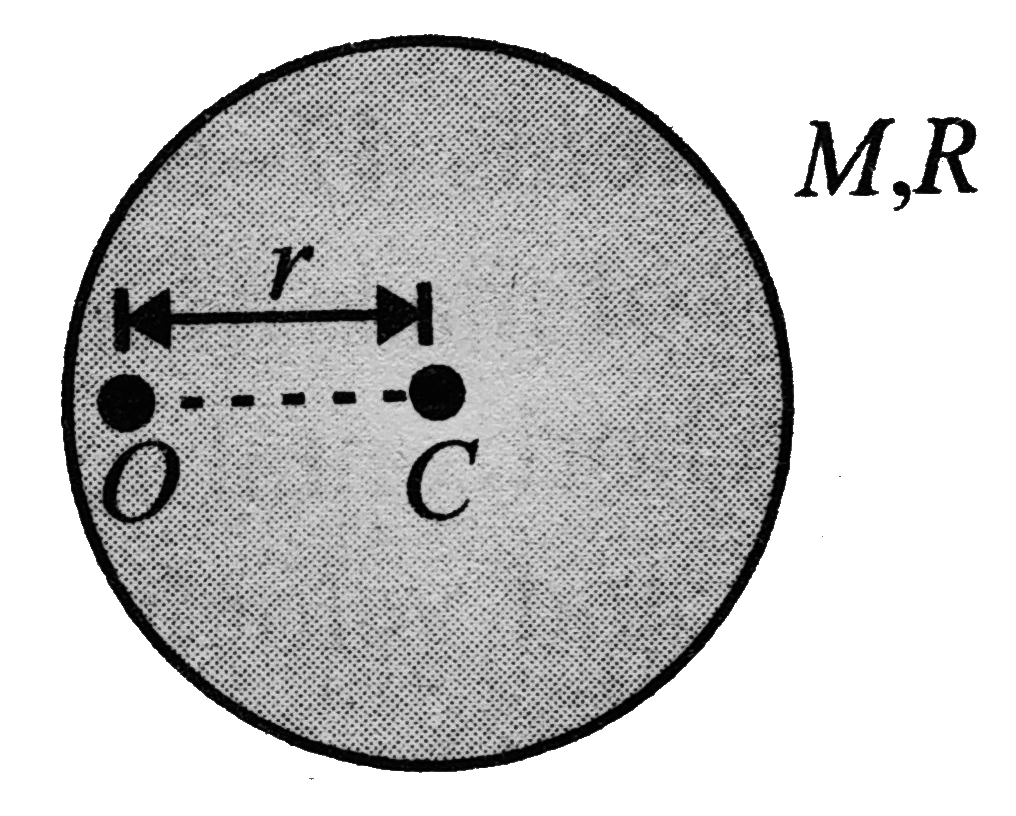
The angular velocity of the disc in the above described case is
View SolutionIn the above question, the velocity of the rear 2kg block after it separates from the spring will be-
View Solution
