Two elastic bodies P and Q having equal mases are moving along the same line with velocities of 16 m/s and 10 m/s respectively. Their velocities after the elastic collision will be in m/s :
0 and 25
5 and 20
10 and 16
20 and 5
The correct Answer is:C
To solve the problem of finding the velocities of two elastic bodies P and Q after an elastic collision, we can follow these steps:
1. Identify the Given Data:
- Mass of body P (m1) = m
- Mass of body Q (m2) = m
- Initial velocity of body P (u1) = 16 m/s
- Initial velocity of body Q (u2) = 10 m/s
2. Conservation of Momentum:
In an elastic collision, the total momentum before the collision is equal to the total momentum after the collision. Therefore, we can write:
m⋅u1+m⋅u2=m⋅v1+m⋅v2
Since the masses are equal, we can simplify this to:
u1+u2=v1+v2
Substituting the values:
16+10=v1+v2
26=v1+v2(Equation 1)
3. Relative Velocity in Elastic Collisions:
For elastic collisions, the relative velocity of separation is equal to the relative velocity of approach. This can be expressed as:
v2−v1=−(u2−u1)
Substituting the values:
v2−v1=−(10−16)
v2−v1=6(Equation 2)
4. Solving the Equations:
Now we have two equations:
- Equation 1: v1+v2=26
- Equation 2: v2−v1=6
From Equation 2, we can express v2 in terms of v1:
v2=v1+6
Substitute this expression for v2 into Equation 1:
v1+(v1+6)=26
2v1+6=26
2v1=20
v1=10 m/s
5. Finding v2:
Now substitute v1 back into the expression for v2:
v2=v1+6=10+6=16 m/s
Final Result:
The velocities after the elastic collision are:
- Velocity of body P (v1) = 10 m/s
- Velocity of body Q (v2) = 16 m/s
- Mass of body P (m1) = m
- Mass of body Q (m2) = m
- Initial velocity of body P (u1) = 16 m/s
- Initial velocity of body Q (u2) = 10 m/s
In an elastic collision, the total momentum before the collision is equal to the total momentum after the collision. Therefore, we can write:
m⋅u1+m⋅u2=m⋅v1+m⋅v2
Since the masses are equal, we can simplify this to:
u1+u2=v1+v2
Substituting the values:
16+10=v1+v2
26=v1+v2(Equation 1)
For elastic collisions, the relative velocity of separation is equal to the relative velocity of approach. This can be expressed as:
v2−v1=−(u2−u1)
Substituting the values:
v2−v1=−(10−16)
v2−v1=6(Equation 2)
Now we have two equations:
- Equation 1: v1+v2=26
- Equation 2: v2−v1=6
v2=v1+6
v1+(v1+6)=26
2v1+6=26
2v1=20
v1=10 m/s
Now substitute v1 back into the expression for v2:
v2=v1+6=10+6=16 m/s
The velocities after the elastic collision are:
- Velocity of body P (v1) = 10 m/s
- Velocity of body Q (v2) = 16 m/s
Similar Questions
Two objects having equal masses are moving with uniform velocities of 2 m/s and 6 m/s respectively. Calculate the ratio of their kinetic energies.
View SolutionA railway carriage of mass 10gand200g are moving along the same line in the same direction with velocities of 2m/sand1m/s respectively. They collide and after the collison, the first object moves with a velocity of 1.67m/s in the same direction. Determine the velocity of the second object.
View Solution
Knowledge Check
Two perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
A0and25B5and20C10and15D20and5Two equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
A+4m/s for bothB−3m/sand+5m/sC−4m/sand+4m/sD−5m/sand+3m/sTwo particles A and B of masses 10 kg and 38 kg, respectively, are moving along the same straight line with velocities 15 m/s and 3 m/s, respectively, in the same direction. After elastic collision, the velocities of A and B are vAand vB, respectively, in the direction of initial motion. Then
 AvA=20m/s,vB=8msBvA=–4m/s,vB=8m/sCvA=16ms,vB=28m/sDvA=–5m/s,vB=10m/s
AvA=20m/s,vB=8msBvA=–4m/s,vB=8m/sCvA=16ms,vB=28m/sDvA=–5m/s,vB=10m/s
Two objects of masses 100g and 200g are moving along the same line in the same line in the same direction with velocities of 2m/s and 1m/s, respectively. They collide and after the collison, the first object moves at a velocity of 1.67m/s in the same direction. Determine the velocity of the second object.
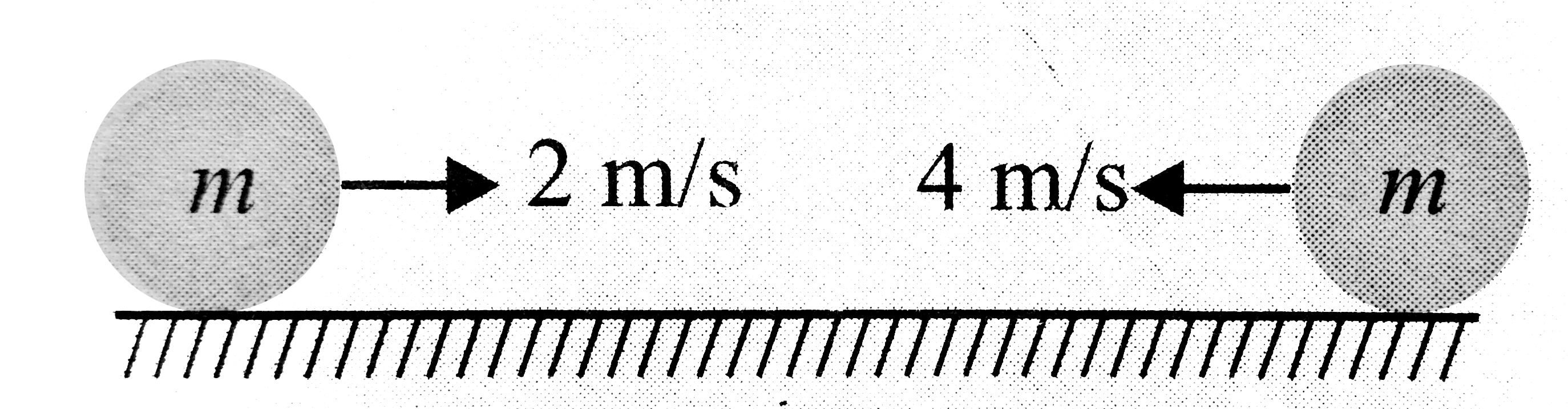
View SolutionTwo identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
View SolutionTwo balls of masses 10 kg and 20 kg approach each other with velocities 30 m/s and 20 m/s respectively . Calculate their velocities after they undergo a perfectly eleastic collision .
View SolutionA 10kg ball and a 20kg ball approach each other with velocities 20m/s and 10m/s respectively. What are their velocities after collision, if the collision is perfectly elastic ?
View SolutionTwo particles P and Q simultaneously start moving from point A with velocities 15m/s and 20m/s respectively. The two particles move with acceleration equal in magnitude but opposite in direction. When P overtakes Q and B then its velocity is 30m/s the velocity of Q at point B will be
View Solution
