Two particles P and Q simultaneously start moving from point A with velocities 15m/s and 20m/s respectively. The two particles move with acceleration equal in magnitude but opposite in direction. When P overtakes Q and B then its velocity is 30m/s the velocity of Q at point B will be
30m/s
5m/s
10m/s
15m/s
The correct Answer is:B
To solve the problem, we will analyze the motion of both particles P and Q step by step.
Step 1: Understand the Initial Conditions
- Particle P starts with an initial velocity uP=15m/s.
- Particle Q starts with an initial velocity uQ=20m/s.
- Both particles start from the same point A at the same time.
Hint: Identify the initial velocities of both particles and note that they start from the same position.
Step 2: Define the Accelerations
- Let the acceleration of particle P be a (positive).
- Since the accelerations are equal in magnitude but opposite in direction, the acceleration of particle Q will be −a (negative).
Hint: Remember that the direction of acceleration affects the velocity change over time.
Step 3: Use the Velocity Formula for Particle P
- When particle P overtakes particle Q at point B, its final velocity is given as vP=30m/s.
- We can use the equation of motion:
v=u+at
For particle P:
30=15+at
Rearranging gives:
at=30−15=15(1)
Hint: Use the equation of motion to relate final velocity, initial velocity, acceleration, and time.
Step 4: Use the Velocity Formula for Particle Q
- Let the final velocity of particle Q at point B be vQ.
- For particle Q, we can use the same equation of motion:
vQ=uQ+(−a)t
Substituting the known values:
vQ=20−at(2)
Hint: Set up the equation for particle Q similarly, noting that its acceleration is negative.
Step 5: Substitute the Value of at from Equation (1) into Equation (2)
- From Equation (1), we have at=15.
- Substitute this into Equation (2):
vQ=20−15
Simplifying gives:
vQ=5m/s
Hint: Substitute the known value of at to find the final velocity of particle Q.
Conclusion
The velocity of particle Q at point B is 5m/s.
Final Answer: The velocity of Q at point B will be 5m/s.
- Particle P starts with an initial velocity uP=15m/s.
- Particle Q starts with an initial velocity uQ=20m/s.
- Both particles start from the same point A at the same time.
- Let the acceleration of particle P be a (positive).
- Since the accelerations are equal in magnitude but opposite in direction, the acceleration of particle Q will be −a (negative).
- When particle P overtakes particle Q at point B, its final velocity is given as vP=30m/s.
- We can use the equation of motion:
v=u+at
For particle P:
30=15+at
Rearranging gives:
at=30−15=15(1)
- Let the final velocity of particle Q at point B be vQ.
- For particle Q, we can use the same equation of motion:
vQ=uQ+(−a)t
Substituting the known values:
vQ=20−at(2)
- From Equation (1), we have at=15.
- Substitute this into Equation (2):
vQ=20−15
Simplifying gives:
vQ=5m/s
The velocity of particle Q at point B is 5m/s.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 3 C.W
25 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Single Type Question
23 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 1 C.W
20 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(H.W)
31 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION
6 Videos
Similar Questions
Two identical bodies A and B moving with velocities 13 m/s and - 15 m/s respectively collide head-on elastically. What will be their velocities after collision?
View SolutionTwo particles 1 and 2 are thrown in the directions shown in figure simultaneously with velocities 5m/sand20m/s. Initially, particle 1 is at height 20 m from the ground. Taking upwards as the positive direction, find
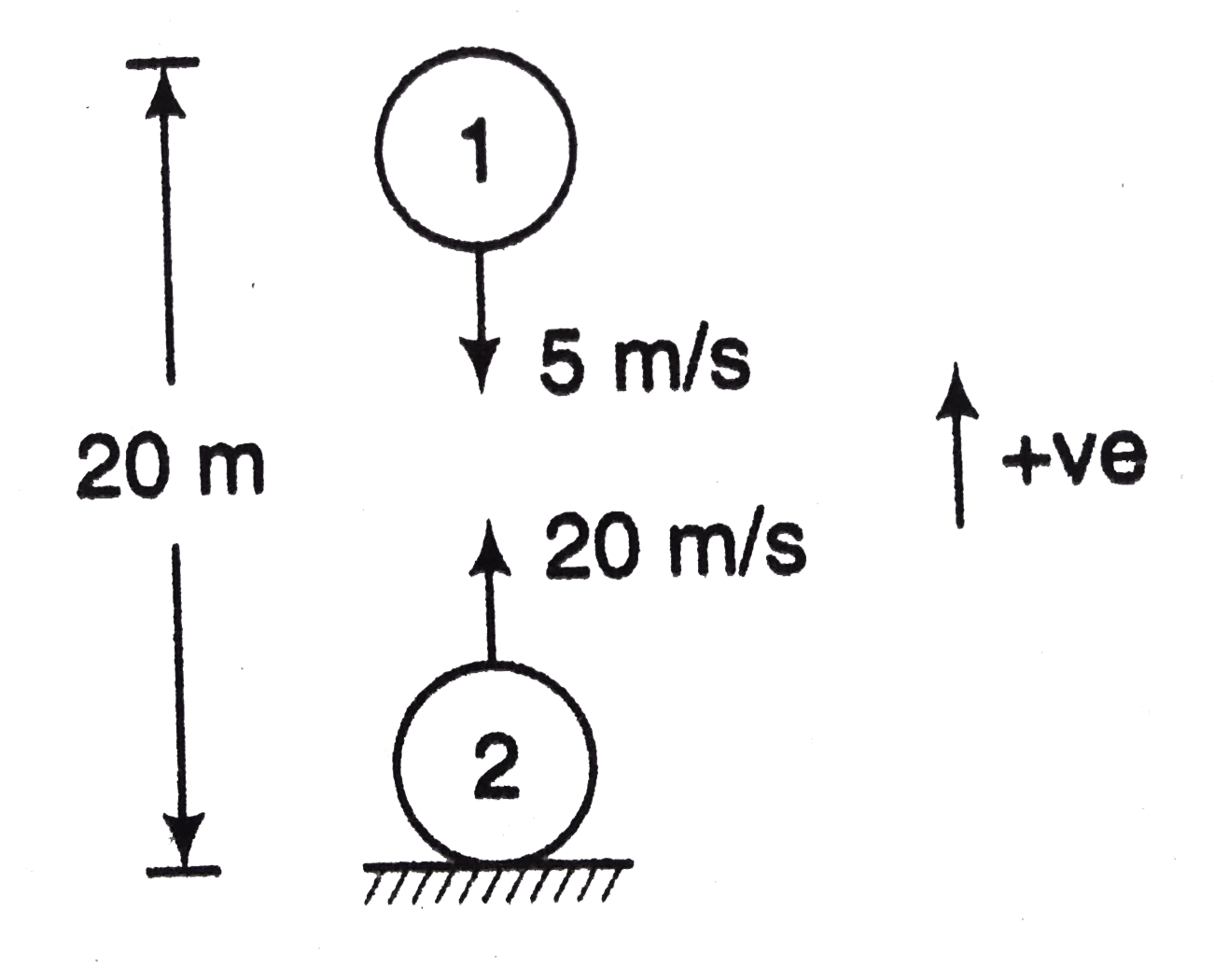
(a) acceleration of 1 with respect to 2
(b) initial velocity of with respect to 1
(c) velocity of 1 with respect to 2 after time t=12s
(d) time when the particles will collide.
View Solution
Knowledge Check
Two elastic bodies P and Q having equal mases are moving along the same line with velocities of 16 m/s and 10 m/s respectively. Their velocities after the elastic collision will be in m/s :
A0 and 25B5 and 20C10 and 16D20 and 5Two particles A and B are projected simultaneously in the direction shown in the figure with velocities VA=20m/s and VB=10m/s respectively. If they collide in air after 1/2 sec. then
 Athe angle θ is 60∘Bthe distance x is 5√3mCthe angle θ is 30∘DThe distance x is 15√3m
Athe angle θ is 60∘Bthe distance x is 5√3mCthe angle θ is 30∘DThe distance x is 15√3mTwo perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
A0and25B5and20C10and15D20and5
Two smooth spheres A and B of masses 4kg and 8kg move with velocities 9m/s and 3m/s in opposite directions. If A rebounds on its path with velocity 1m/s, then
View SolutionA particle is moving with velocity 5 m/s towards cast and its velocity changes to 5 m/s north in 10 s, find the acceleration .
View SolutionTwo cars A and B cross a point P with velocities 10m/s and 15m/s. After that they move with different uniform accelerations and the car A overtakes B with a speed of 25ms−1. What is velocity of B at that instant?
View SolutionA particle is moving with velocity 5 m/s towards cast and its velocity changes to 5 m/s north in 10 s, find the acceleration .
View SolutionA particle is moving with uniform acceleration along a straight line ABC. Its velocity at A and B are 6 m/s and 9 m/s respectively if AB:BC=5:16 then its velocity at C is
View Solution
