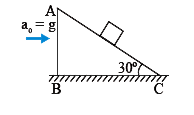
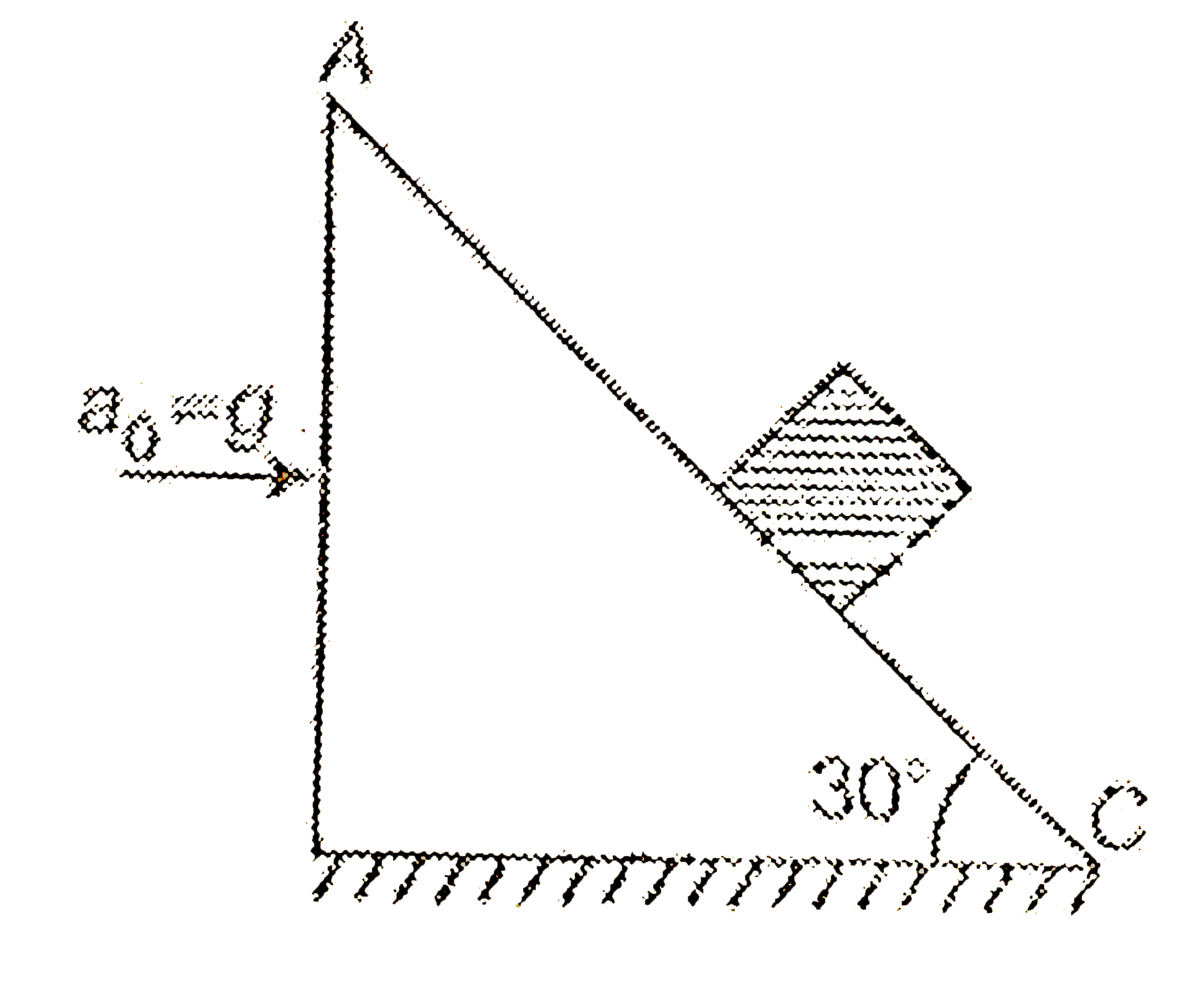
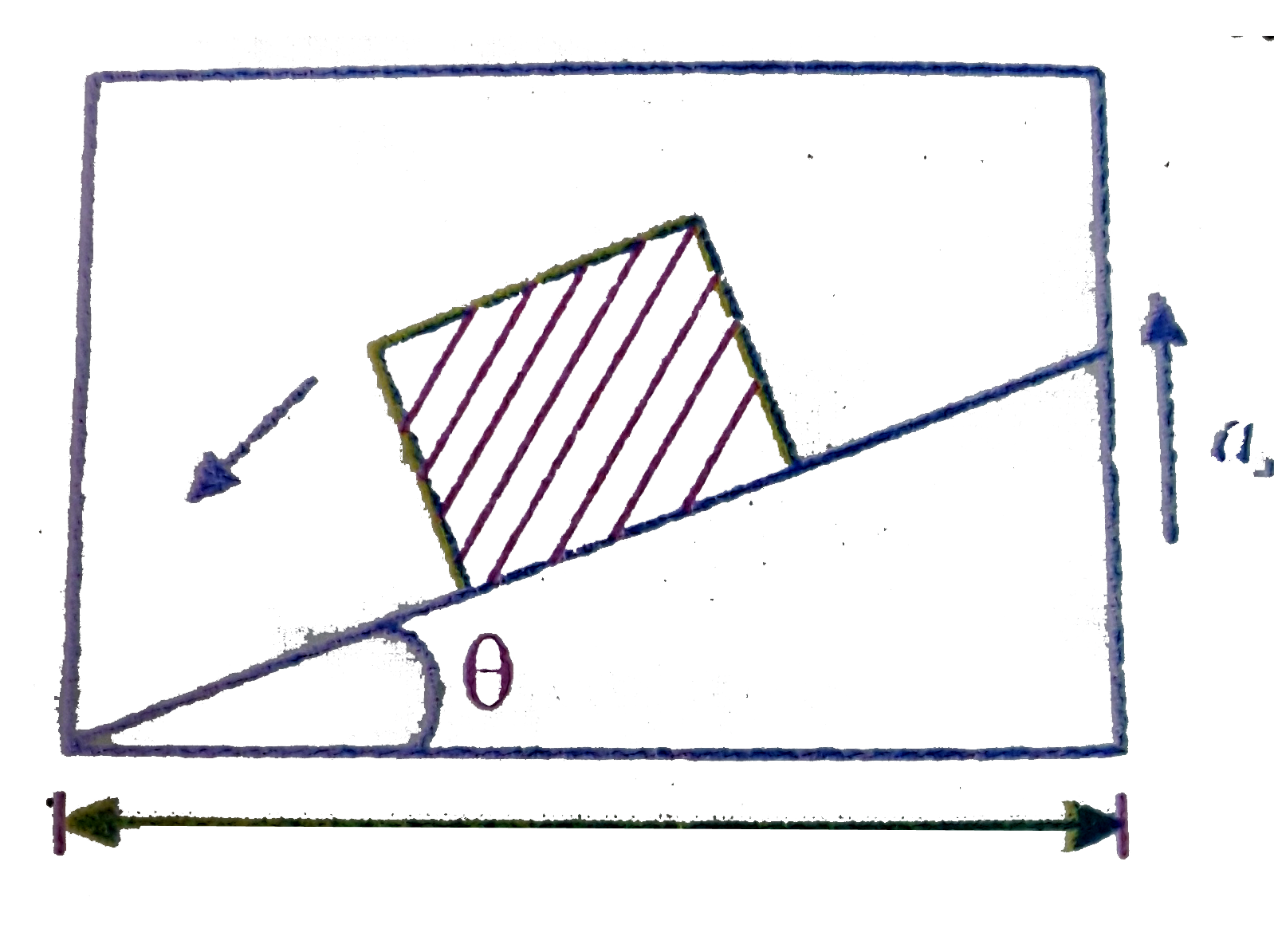
A block is placed on an inclined plane moving towards right horizontally with an acceleration a0=g. The length of the plane AC = 1m. Friction is absent everywhere. The time taken by the block to reach from C to A is : (g=10m/s2)
1.2s
0.74 s
2.56 s
0.42 s
The correct Answer is:2
Step by step video, text & image solution for A block is placed on an inclined plane moving towards right horizontally with an acceleration a_(0) = g. The length of the plane AC = 1m. Friction is absent everywhere. The time taken by the block to reach from C to A is : ( g = 10 m//s^(2)) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
A block is placed on an inclined plane moving towards right horizontally with an acceleration a0=g. The length of the plane AC=1m. Friction is absent everywhere. Find the time taken (in seconds) by the block to reach from C to A.
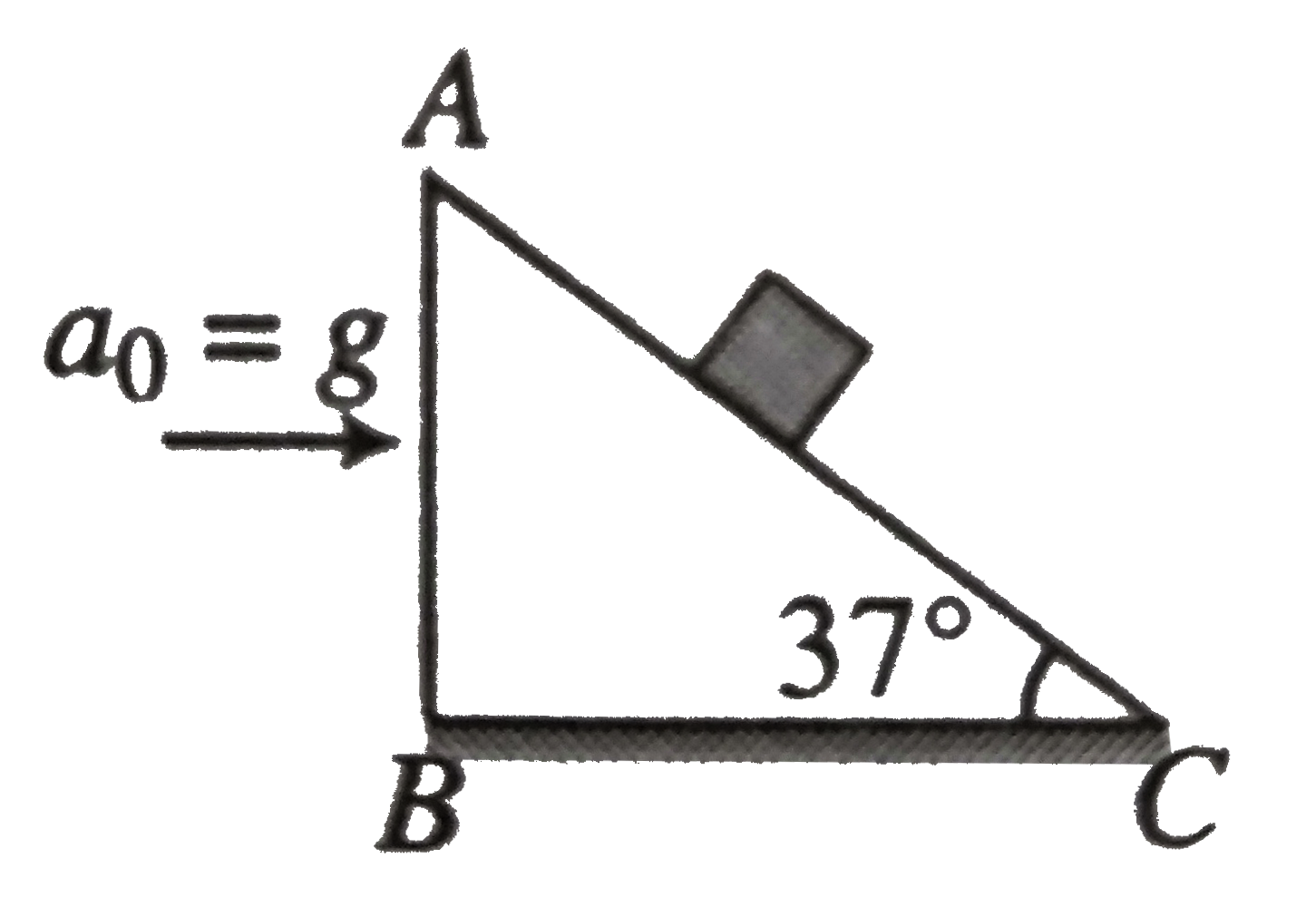
View SolutionA block is placed on an inclined plane moving towards right with an acceleration a0=g The length of the inclined plane is l0. All the surfaces are smooth Find the time taken by the block to reach from bottom to top.
 .
.
View Solution
Knowledge Check
A block is placed on an inclined plane. The block is moving towards right horizontal with an acceleration a0=g. The length of the inclined plane (AC) is equal to 1 m. Whole the situation are shown in the figure. Assume that all the surfaces are frictionless. The time taken by the block to reach from C to A is (Take,g=10m/s2)
 A0.74 sB0.9 sC0.52 sD1.24 s
A0.74 sB0.9 sC0.52 sD1.24 sA block is placed on an inclined plane. The block is moving towards right horizontal with an acceleration a0=g. The length of the inclined plane (AC) is equal to 1 m. Whole the situation are shown in the figure. Assume that all the surfaces are frictionless. The time taken by the block to reach from C to A is (Take,g=10m/s2)
 A0.74 sB0.9 sC0.52 sD1.24 s
A0.74 sB0.9 sC0.52 sD1.24 sA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
AmgBmgcosθCmgcosθDmgtanθ
A block slides down from top of a smooth inclined plane of elevation ● fixed in an elevator going up with an acceleration a0 The base of incline hs length L Find the time taken by the block to reach the bottom
 .
.
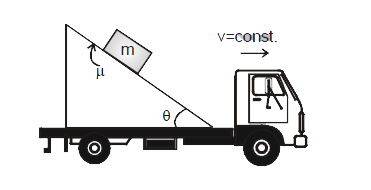
View SolutionA block of mass m is placed on an inclined plane which is moving with constant velocity v in horizontal direction as shown in figure. Then find out work done by the friction in time t if the block is at rest with respect to the incline plane.
View SolutionA body of mass m is placed over a smooth inclined plane of inclination θ, which is placed over a lift which is moving up with an acceleration a0 . Base length of the inclined plane is L. Calculate the velocity of the block with respect to lift at the bottom, if it is allowed to slide down from the top of the plane from rest :-
View SolutionA smooth wedge of mass M is pulled towards left with an acceleration a=gcotθ on a horizontal surface and a block of mass m is released w.r.t. wedge. Then answer the following
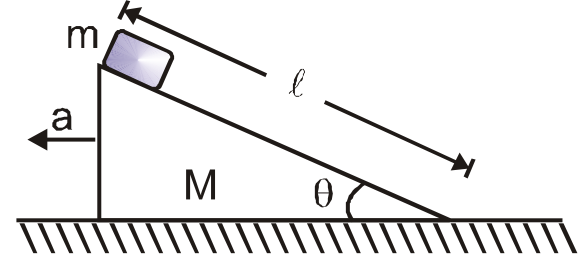
Time taken by the block to reach the ground is
View SolutionA block of 4kg slides down an inclined plane which makes an angle θ=60∘ with the horizontal. The co-effcient of friction between the block and the plane is μ=3/4. The force exerted by the block on the plane is (g=10m/s2)
View Solution
