A block of mass m is placed on an inclined plane which is moving with constant velocity v in horizontal direction as shown in figure. Then find out work done by the friction in time t if the block is at rest with respect to the incline plane.
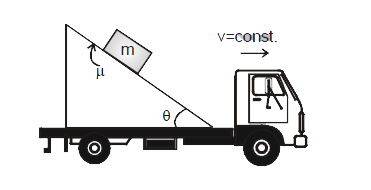
F.B.D. of block with respect to ground
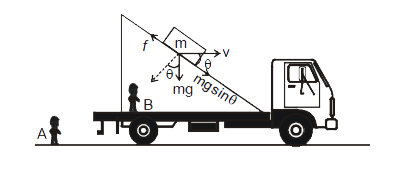
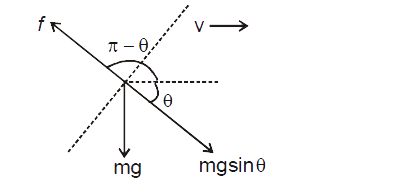
Block is at rest with respect to wedge
⇒ f = mg sin θ
In time t the displacement of block with respect to ground d = vt
Work done by friction for man A
Wf = (component of friction force along displacement) ×displacement
Wf = mg sinθ.vt cos(180∘−θ))
Wf=−mg vt cosθsinθ
Wf for man B = 0 (displacement is zero with respect to man B)
Topper's Solved these Questions
Similar Questions
An inclined plane is moving up with constant velocity v. A block kept on incline is at rest. Calculate the work done by gravity, friction force, and normal reaciton on block in time interval of t.
View SolutionA heavy block of length b and height h is placed at rest on a rough inclined plane of inclination θ with the horizontal, as shown in figure.

View Solution
Knowledge Check
A block of mass m in the equilib rium on a rough inclined plane with inclination α and coefficient of friction as shown in the figure (μ<tanα). A force F is applied on the block which makes an angle θ with the horizontal as shown in diagram.
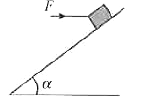 ANormal force on the block mgcosα+Fsinθ.BMinimum force f to keep the blockin equilibrium Fmin=mg(sinα−μcosα)2μ√1+μ2Cμ=tanθDNone of these
ANormal force on the block mgcosα+Fsinθ.BMinimum force f to keep the blockin equilibrium Fmin=mg(sinα−μcosα)2μ√1+μ2Cμ=tanθDNone of theseA block of mass 'M' is slipping down on a rough inclined of inclination α with horizontal with a constant velocity. The magnitude and direction of total reaction from the inclined plane on the block is :
AMgsinα down the inclinedBless than Mgsinα down the inclinedCMg upwardsDMg down wardsAn inclined plane is moving with Constant velocity v=4m/s on a horizoontal surface as shown in figure. If a block of mass 2kg is kept at top of the incline and their is no friction between the block and the incline, then the distance travelled by the incline till the block reaches bottom of the inclined is (g=10m/s2)
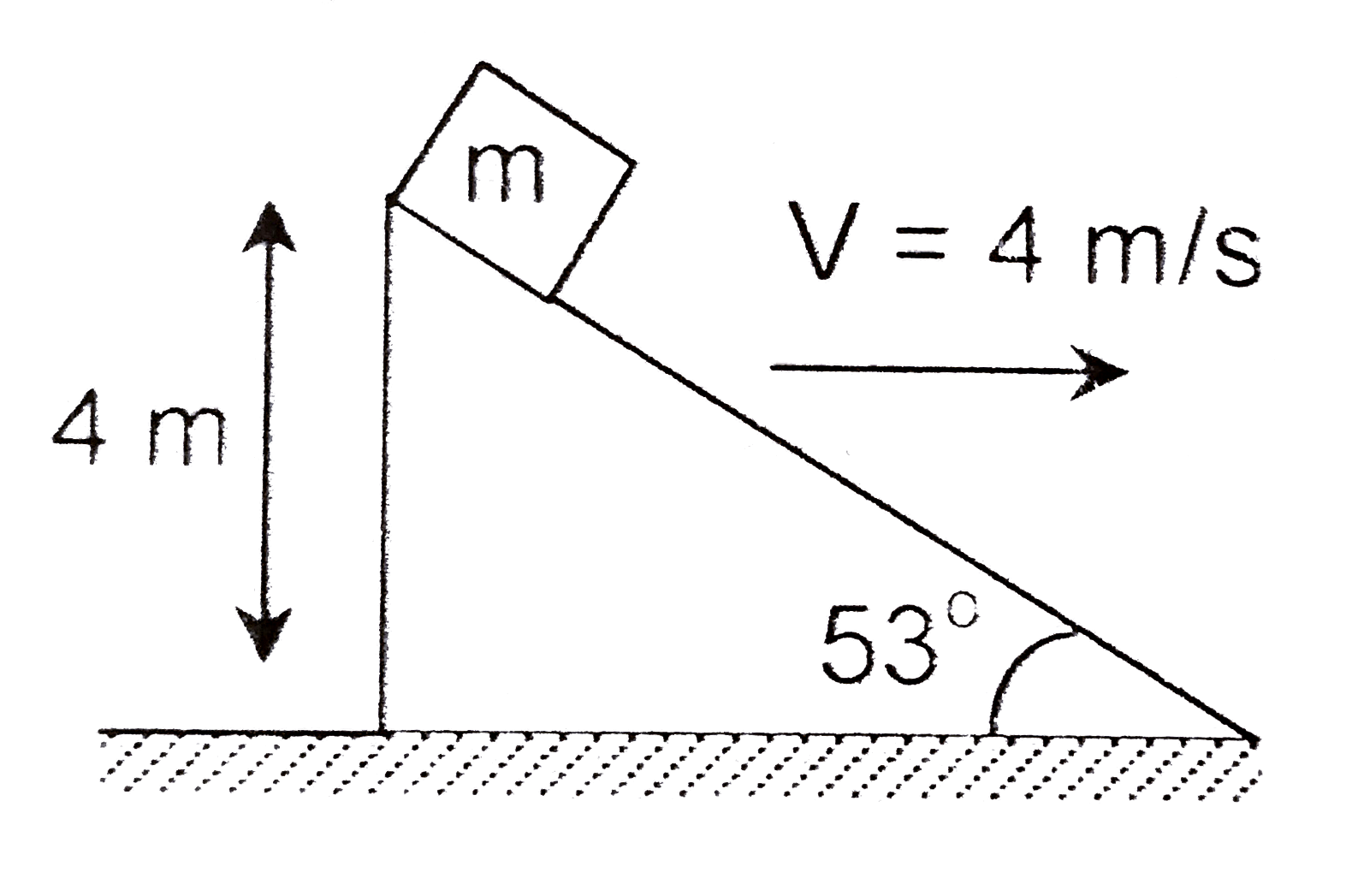 A4mB5mC1.6√5mD2√5m
A4mB5mC1.6√5mD2√5m
A rough inclined plane is placed on a cart moving with a constant velocity u on horizontal ground. A block of mass M rest on the incline. Is any work done by force of friction between the block and incline ? Is there then a dissipation of energy ?
View SolutionBlock of mass 10 kg is moving on inclined plane with constant velocity 10 m/s. The coedfficient of kinetic friction between incline plane and block is :-

View SolutionA block of mass 4kg is released from the top of a rough inclines plane of height 10m. If velocity of the block is10ms at the bottom of the inclined then work done by the friction force on the block will be
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA block of mass 10 kg is placed on an inclined plane when the angle of inclination is 30∘. The block just begins to slide down the plane. The force of static friction is
View Solution
