A body of mass 3kg moving with speed 5ms−1 , hits a wall at an angle of 60∘ and return at the same angle. The impact time was 0.2 sec. calculate the force exerted on the wall :
150√3N
50√3N
100N
75√3N
The correct Answer is:4
To solve the problem step by step, we will calculate the force exerted on the wall by the body after it collides with it.
Step 1: Identify the given data
- Mass of the body, m=3kg
- Initial speed of the body, v=5m/s
- Angle of incidence and reflection, θ=60∘
- Impact time, Δt=0.2s
Step 2: Resolve the initial velocity into components
The initial velocity can be resolved into horizontal (x-direction) and vertical (y-direction) components using trigonometric functions:
- vix=vcos(θ)=5cos(60∘)=5×12=2.5m/s
- viy=vsin(θ)=5sin(60∘)=5×√32=5√32m/s
Step 3: Determine the final velocity components after the collision
After hitting the wall, the body returns at the same angle but in the opposite direction:
- vfx=−vix=−2.5m/s (horizontal component changes direction)
- vfy=viy=5√32m/s (vertical component remains the same)
Step 4: Calculate the change in momentum
Momentum is calculated as p=mv. We will calculate the initial and final momentum in the x and y directions.
Initial momentum:
- pix=mvix=3×2.5=7.5kg m/s
- piy=mviy=3×5√32=15√32kg m/s
Final momentum:
- pfx=mvfx=3×(−2.5)=−7.5kg m/s
- pfy=mvfy=3×5√32=15√32kg m/s
Change in momentum:
- Δpx=pfx−pix=−7.5−7.5=−15kg m/s
- Δpy=pfy−piy=15√32−15√32=0
Step 5: Calculate the total change in momentum
Since the y-component cancels out, the total change in momentum is:
- Δp=Δpx=−15kg m/s
Step 6: Calculate the force exerted on the wall
Using the formula for force:
F=ΔpΔt
Substituting the values:
F=−150.2=−75N
Since we are interested in the magnitude of the force, we take the absolute value:
F=75N
Step 7: Final answer
The force exerted on the wall is:
F=75√3N
- Mass of the body, m=3kg
- Initial speed of the body, v=5m/s
- Angle of incidence and reflection, θ=60∘
- Impact time, Δt=0.2s
The initial velocity can be resolved into horizontal (x-direction) and vertical (y-direction) components using trigonometric functions:
- vix=vcos(θ)=5cos(60∘)=5×12=2.5m/s
- viy=vsin(θ)=5sin(60∘)=5×√32=5√32m/s
After hitting the wall, the body returns at the same angle but in the opposite direction:
- vfx=−vix=−2.5m/s (horizontal component changes direction)
- vfy=viy=5√32m/s (vertical component remains the same)
Momentum is calculated as p=mv. We will calculate the initial and final momentum in the x and y directions.
- pix=mvix=3×2.5=7.5kg m/s
- piy=mviy=3×5√32=15√32kg m/s
- pfx=mvfx=3×(−2.5)=−7.5kg m/s
- pfy=mvfy=3×5√32=15√32kg m/s
- Δpx=pfx−pix=−7.5−7.5=−15kg m/s
- Δpy=pfy−piy=15√32−15√32=0
Since the y-component cancels out, the total change in momentum is:
- Δp=Δpx=−15kg m/s
Using the formula for force:
F=ΔpΔt
Substituting the values:
F=−150.2=−75N
Since we are interested in the magnitude of the force, we take the absolute value:
F=75N
The force exerted on the wall is:
F=75√3N
Similar Questions
A body of mass 3 kg moving with velocity 10 m/s hits a wall at an angle of 60∘ and returns at the same angle. The impact time was 0.2 s. Calculate the force exerted on the wall.
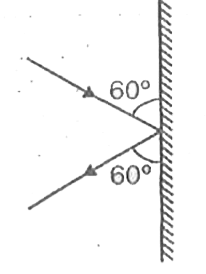
View SolutionA body of mass 3 kg hits a wall at an angle of 60∘ & returns at the same angle. The impact time was 0.2s. Calculate the force exerted on the wall:
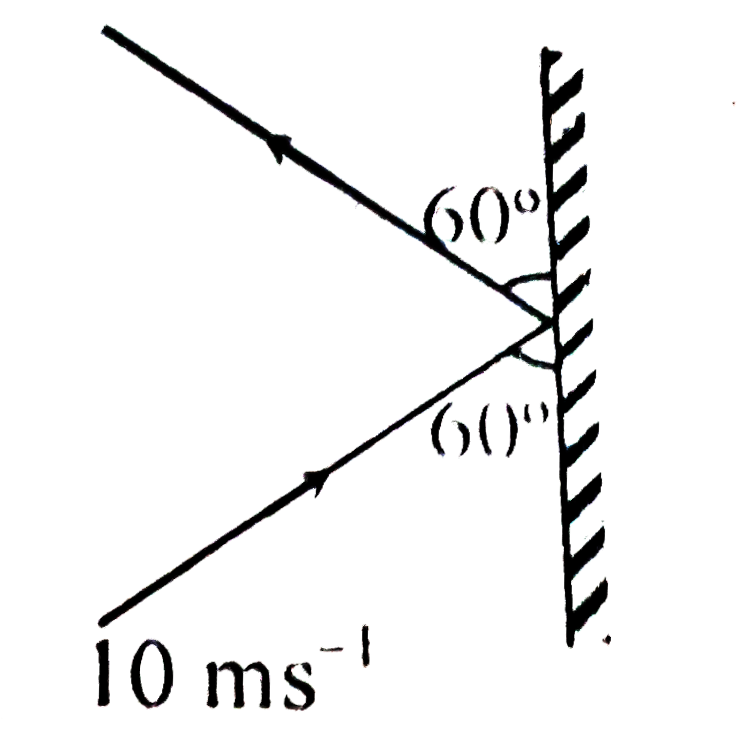
View Solution
Knowledge Check
A body of mass 3kg hits a wall at an angle of 60∘ and with speed of 10m/s and returns at the same angle and with the same velocity. The impact time is 0.2sec. Calculate force exterted on the wall
 A150√3NB100NC50√3ND75√3N
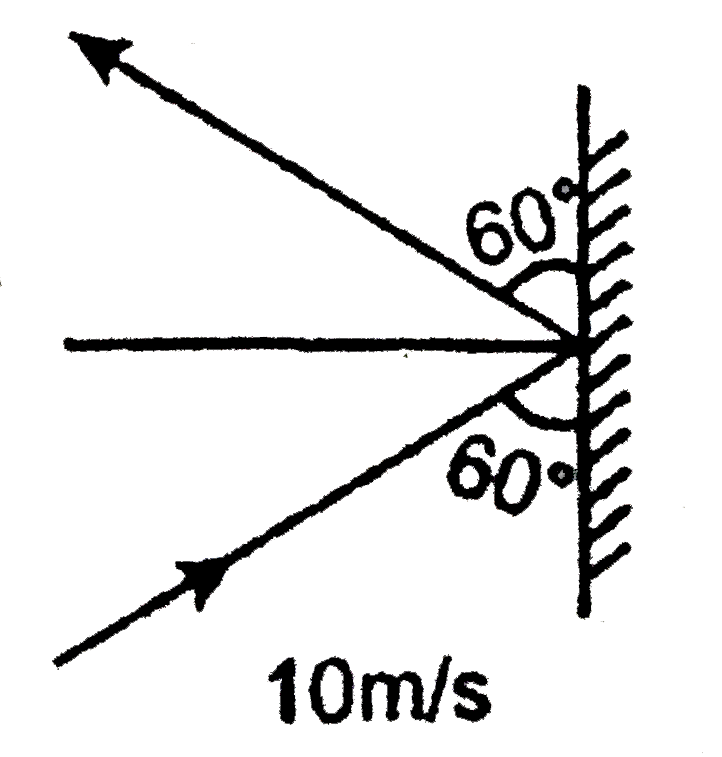
A150√3NB100NC50√3ND75√3NA ball of mass 3kg moving with a speed of 100 m/s, strikes a wall at an angle 60∘ (as shown in figure). The ball rebounds at the same speed and remains in contact with the wall for 0.2 s, the force exerted by the ball on the wall is
 A1500√3NB1500NC300√3ND300N
A1500√3NB1500NC300√3ND300NA 100g iron ball having velocity 10m/s collides with a wall at an angle 30∘ and rebounds with the same angle. If the period of contact between the ball and wall and wall is 0.1 second, then the force experienced by the wall is
A10NB100NC1.0ND0.1N
A ball moving with a momentum of 5kgms−1 strikes against a wall at an angle of 45∘ and is reflected at the same angle Calculate the impulse .
View SolutionA ball moving with a momentum of 5kgms−1 strikes against a wall at an angle of 45∘ and is reflected at the same angle. Calculate the change in momentum.
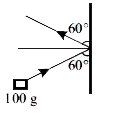
View SolutionA mass of 100 g strikes the wall with a speed of 5 m s−1 at an angle 60∘ as shown in figure and it rebounds with the same speed. If the contact time is 2×10−3s. what is the force applied by the wall?
View SolutionA bullet of mass 20 g moving with a speed of 120 m/s hits a thick muddy wall and penetrates into it. It takes 0.03 s to stop in the wall find the force exerted by the bullet on the wall,
View SolutionA ball of mass 250 g moving with 20 m/s strikes a vertical wall and rebounds along the same line with a velocity of 15 m/s. If the time of contact is 0.1 s, the force exerted by the wall on the ball is
View Solution
