Consider the functions f(x)=√x and g(x)x2−b. In the standard (x, y) coordinate plane, y=f(g(x)) passes through the point (3, 5). What is the value of b ?
16
4
−2
−16
The correct Answer is:D
To solve the problem, we need to find the value of b such that the function y=f(g(x)) passes through the point (3,5). We are given the functions:
- f(x)=√x
- g(x)=x2−b
Step 1: Substitute g(x) into f(x)
We start by substituting g(x) into f(x):
y=f(g(x))=f(x2−b)=√x2−b
Step 2: Use the given point (3,5)
We know that the function passes through the point (3,5). This means when x=3, y should equal 5. We can set up the equation:
5=√32−b
Step 3: Square both sides to eliminate the square root
To eliminate the square root, we square both sides of the equation:
52=32−b
This simplifies to:
25=9−b
Step 4: Solve for b
Now, we can solve for b by isolating it:
b=9−25
b=−16
Conclusion
The value of b is −16.
---
- g(x)=x2−b
b=−16
Topper's Solved these Questions
- INTERMEDIATE ALGEBRA/COORDINATE GEOMETRYBOOK - ENGLISH SATCHAPTER - INTERMEDIATE ALGEBRA/COORDINATE GEOMETRYEXERCISE - EXERCISE
12 Videos
- IMPROVING YOUR MATH SCOREBOOK - ENGLISH SATCHAPTER - IMPROVING YOUR MATH SCOREEXERCISE - VERY CHALLENGING PROBLEMS
12 Videos
- LINEAR FUNCTIONS BOOK - ENGLISH SATCHAPTER - LINEAR FUNCTIONS EXERCISE - EXERCISES
7 Videos
Similar Questions
Knowledge Check
What is the y-intercept of the line in the standard (x,y) coordinate plane that goes through the points (-3,6) and (3,2) ?
A0B2C4D6The graph of the function f(x)=x2−x−3x−1 is shown in the standard (x,y) coordinate plane below . Which of the following , if any is a list of each of the vertical asymptotes of f(x) ?
 AThis function has no vertical asymptote .By=−12x+1Cy=2x-1Dx=1
AThis function has no vertical asymptote .By=−12x+1Cy=2x-1Dx=1The function y=f(x) is graphed in the standard (x, y) coordinate plane below.
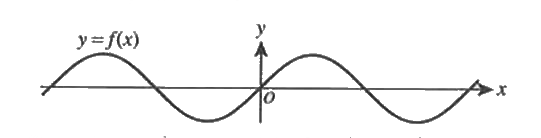
The points on the graph of the function y=3+f(x−1) can be obtained from the points on y=f(x) by a shift of:A1 unit to the right and 3 unit up.B1 unit to the right and 3 unit down.C3 unit to the right and 1 unit up.D3 unit to the right and 1 unit down.
If f(x)=3x−√xandg(x)=x2, what is g(f(4))?
View Solutionv) What type of function is y=f(x)g(x) ?
View SolutionLet f(x)=2x−sinx and g(x)=3√x . Then
View SolutionIf the function f(x)=x3+ex2 and g(x)=f−1(x), then the value of g'(1) is
View SolutionGiven functions f(x)=2x+1andg(x)=x2−4, what is the value of f(g(−3))?
View Solution