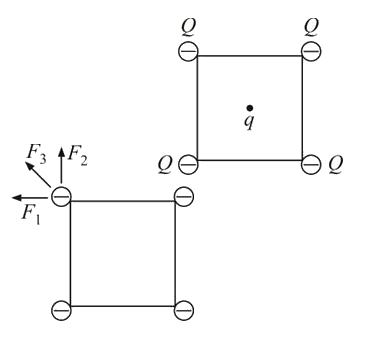
Four charges equal to −Q are placed at the four corners fo a square and a charge q is at its centre, If the system is in equilibrium the value of q si
−Q4(1+2√2)
Q4(1+2√2)
−Q2(1+2√2)
Q2(1+2√2)
The correct Answer is:B
The system is in equilibrium net force on any charge will be zero. Consider any corner’s charge
Resultant of all three forces is √2kQ2a2+kQ2(√2a)2
The must be balanced by attraction of charge at centre
kQq(12√2a)=kQqa2/2
On solving we get 2kQqa2=√2kQ2a2+kQ22a2
2q=√2Q+Q2⇒q=Q√2+Q4(1√2+14)=Q4(1+2√2)
Similar Questions
Four positive point charges Q are placed at the corners of a square of side a. Calculate the charge that should be placed at the centre so that system remains in equilibrium.
View SolutionThree charges, each of value q, are placed at the comers of an equilateral triangle. A fourth charge Q is placed at the centre of the triangle. If the value of Q for which charges remain stationary is (−q√n), then find n.
View Solution
Knowledge Check
Four charges equal to −Q are placed at the four corners of a square and a charge q is at its centre. If the system is in equilibrium the value of q is
A(a) −Q2(1+2√2)B(b) Q4(1+2√2)C(c) −Q4(1+2√2)D(d) Q2(1+2√2)Four charges equal to Q are placed at the four comers of a square and a charge q is at its centre . If the system is in equilibrium , the value of q is
A−Q4(1+2√2)BQ4(1+2√2)C−Q2(1+2√2)DQ2(1+2√2)Four charges +Q,−Q,+Q,−Q are placed at the corners of a square taken in order. At the centre of the square
AE=0,V=0BE=0,V≠0CE≠0,V=0DE=0,V≠0
Four identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .
.
View SolutionFour identical charges, Q each, are fixed at the vertices of a square. A free charge q is placed at the centre of the square. Investigate the nature of equilibrium of charge q if it is to be displaced slightly along any of the two diagonals of the square.
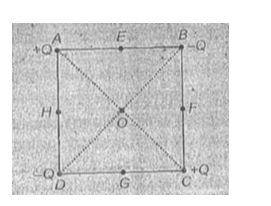
View SolutionFour charges Q-Q, Q-Q are placed at the corners of a square as shown . The potential is zero at points
View SolutionA charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
View SolutionFour positive charges (2√2−1)Q are arranged at the four corners of a square. Another charge q is placed at the center of the square. Resulting force acting on each corner charge is zero if q is
View Solution