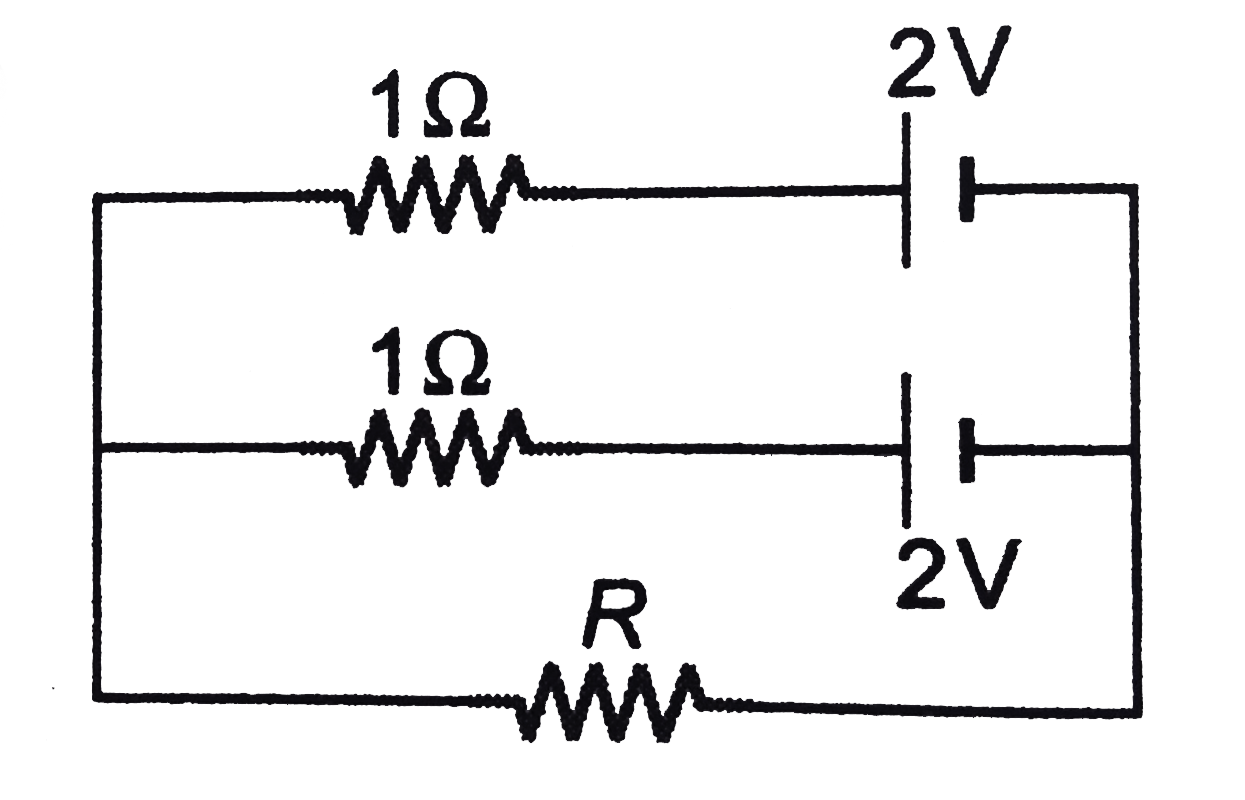
Two ideal batteries of emf and three resistances are connected as shown in the figure. The current in resistance would be zero if
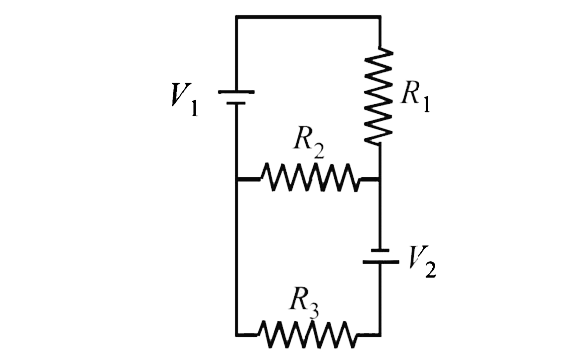
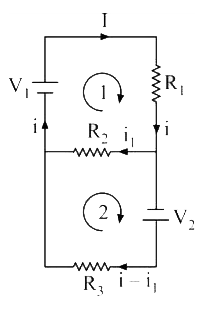
From loop 1
……(i)
From loop 2
………(ii)
Solving equation (i) and (ii)
We get ,
If is zero
It is possible in followin options
(a) (b)
Similar Questions
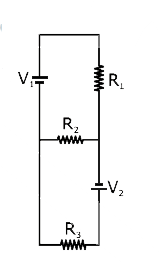
Two ideal batteries of emf V1andV2 and these resistances R1,R2andR3 are connected as shown in the figure . The current in resistance R2 would be zero if
 View Solution
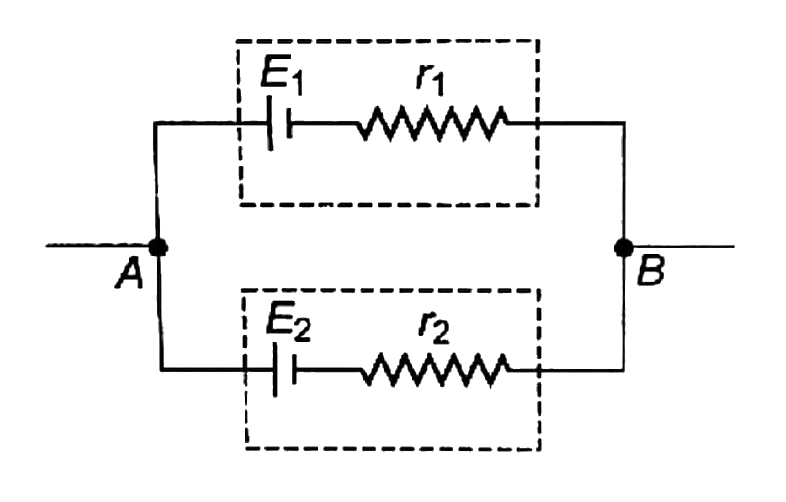
View SolutionTwo batteries of emf E1 and E2(E2>E1) and internal resistance r1 and r2 respectively are connected in parallel as shown in the figure. Then, which of the followings statements is correct ?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two ideal batteries of emfV1andV2 and three resistance R1R2andR3 are connected The current in resistance R2 would be zero if
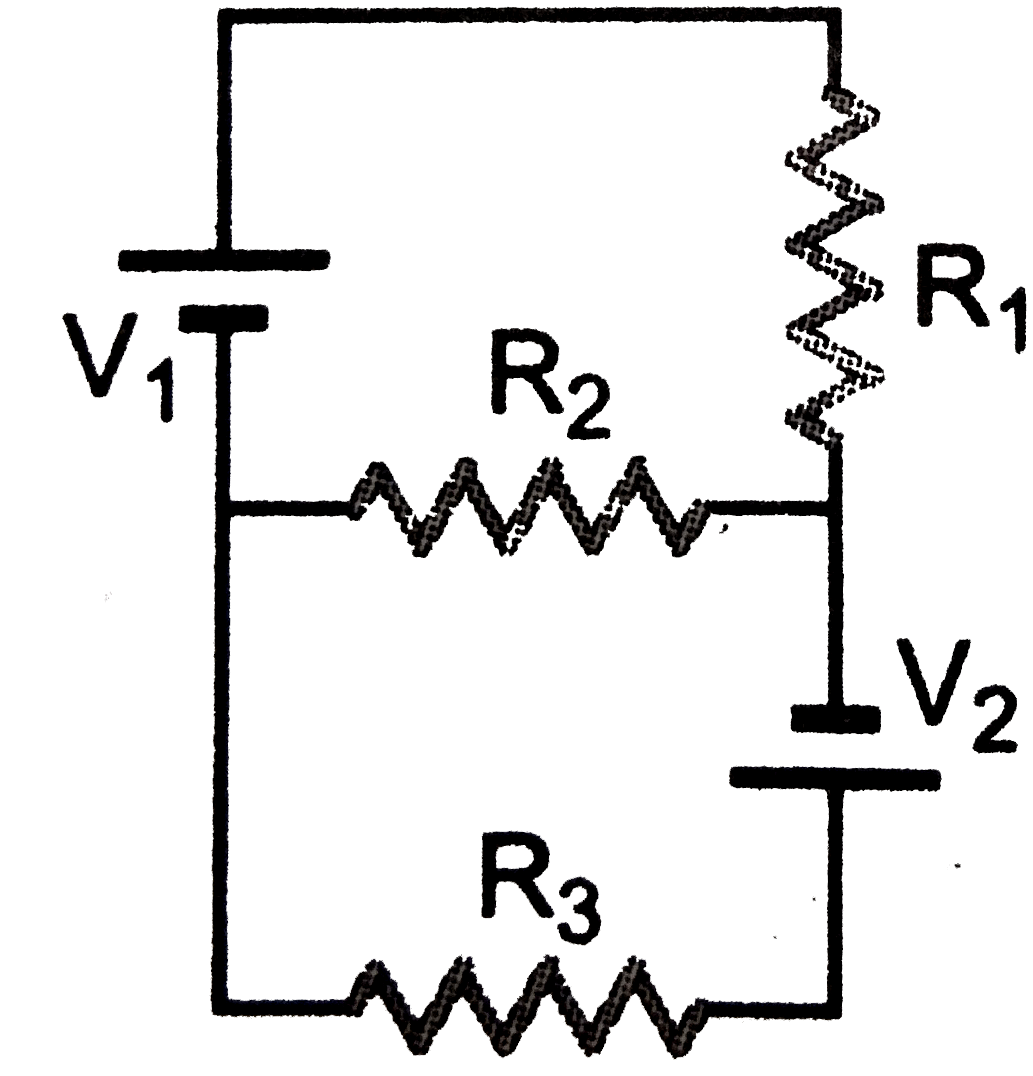 AV1=V2andR1=R2=R3BV1=V2andR1=2R2=R3CV1=2V2and2R1=2R2=R3D2V1=V2and2R1=R2=R3
AV1=V2andR1=R2=R3BV1=V2andR1=2R2=R3CV1=2V2and2R1=2R2=R3D2V1=V2and2R1=R2=R3 - Question 2 - Select One
Three batteries of emf E1,E2 and E3 and internal resistance r1,r2 and r3 are connected as shown. The current through the battery of emf E3 is zero if.
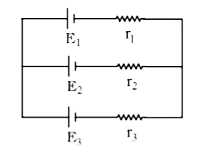 AE1r2+E2r1=E3(r1+r2)BE1r2−E2r1=E3(r1+r2)CE1r2+E2r1=E3(r1+r2)DE1r1+E2r2=E3(r1+r2)
AE1r2+E2r1=E3(r1+r2)BE1r2−E2r1=E3(r1+r2)CE1r2+E2r1=E3(r1+r2)DE1r1+E2r2=E3(r1+r2) - Question 3 - Select One
The given circuit has two ideal diodes connected as show in the figure. The current flowing through the resistance R1 will be
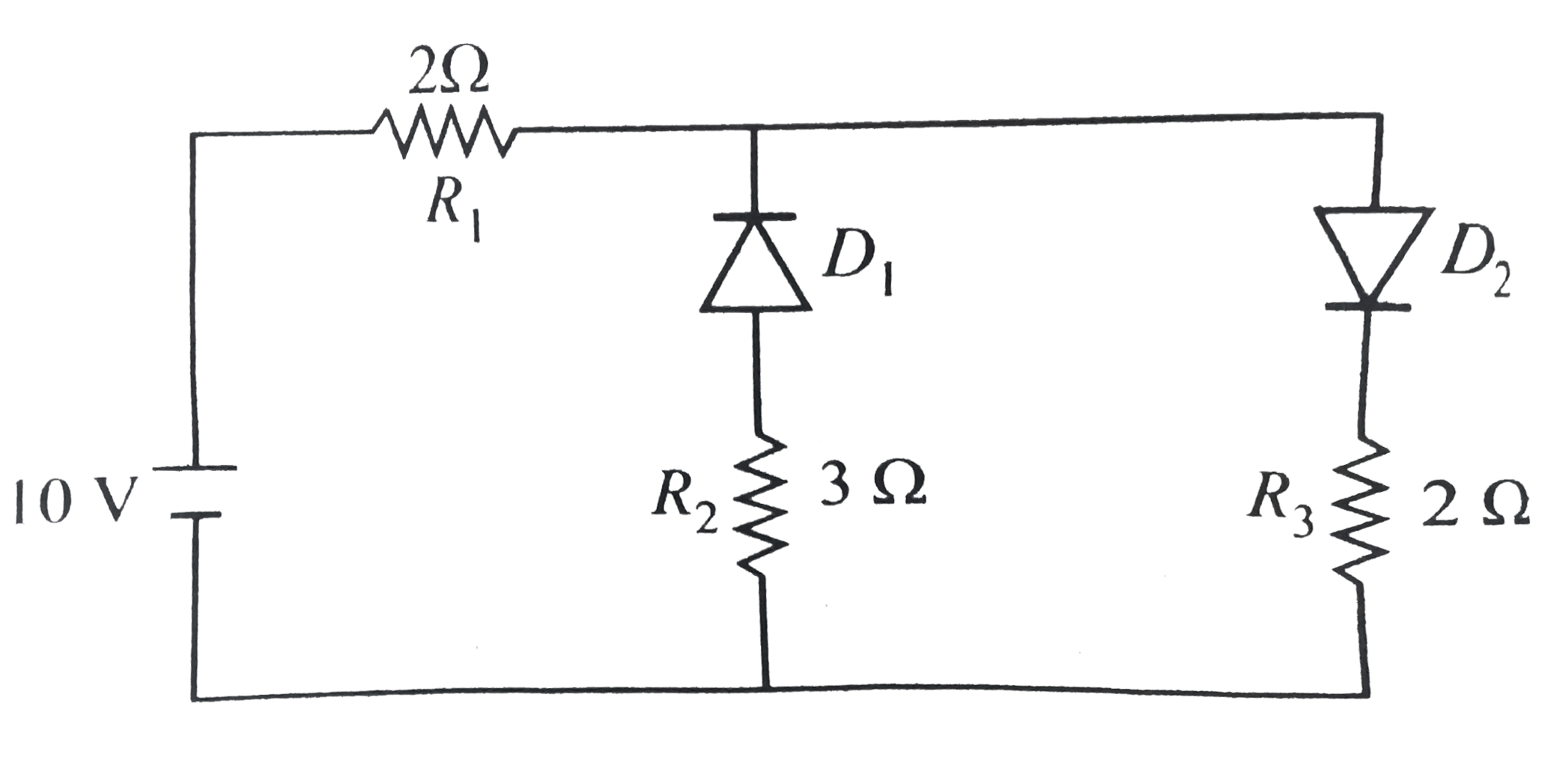 A2.5 AB10.0 AC1.43 AD3.13 A
A2.5 AB10.0 AC1.43 AD3.13 A
Two non-ideal batteries have e.m.f 2V and 8V respectively and internal resistance 1 Omega and 2 Omega respectively as shown in the figure. If the potential difference between a and b is zero then the resistance R has a value
_PHY_E01_014_Q01.png) View Solution
View SolutionThree resistors having resistance r1, r2, r3 are connected as shown. The ratio i3i1 of current in terms of resistance used in circuit is
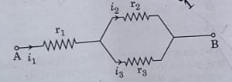 View Solution
View SolutionThe given circuit has two ideal diodes connected as show in the figure. The current flowing through the resistance R1 will be
 View Solution
View SolutionTwo batteries of emf ε1 and ε2(ε2>ε1) and internal resistances r_1 and r2 respectively are connected in parallel as shown in Fig. Then
 View Solution
View SolutionTwo identical batteries, each of emf 2V and internal resistance r=1Ω are connected as shown. The maximum power that can be developed across R using these batteries is
 View Solution
View Solution
