A rail track made of steel having length 10 m is clamped on a railway line at its two ends

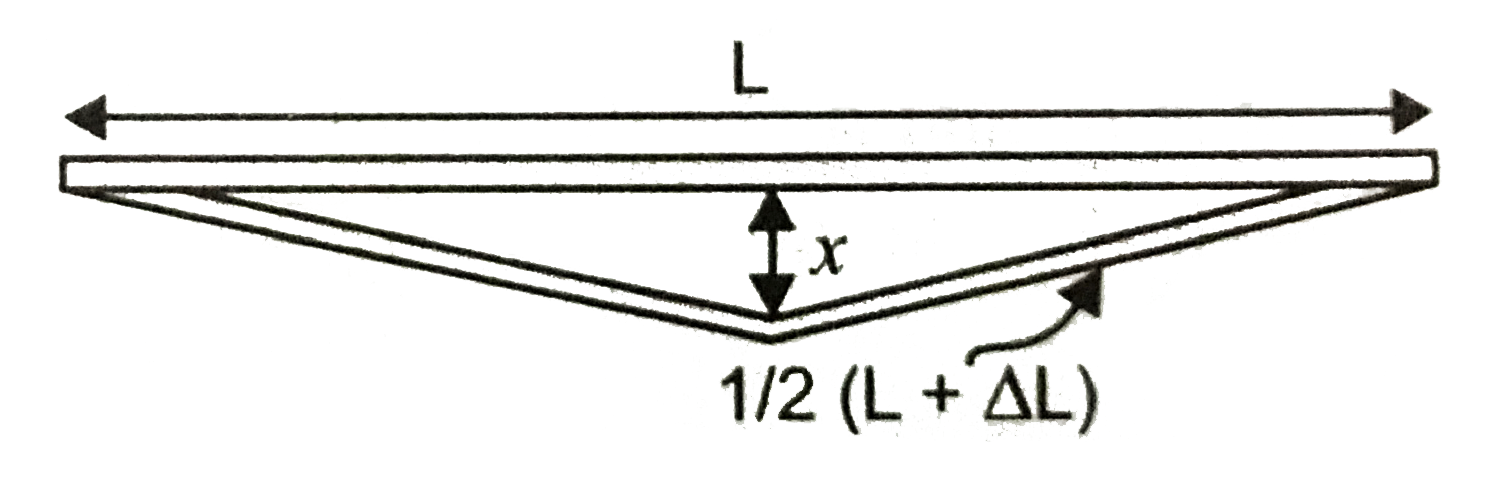
on a summer day due to rise in temperature by $20^{\circ} C,$ it is deformed as shown in fig. Find x (displacement of the centre) if $α_{s t e e l} = 1.2 \times 10^{- 5} /^{\circ} C .$

Video Solution

Text Solution

Verified by Experts

Consider the diagram,

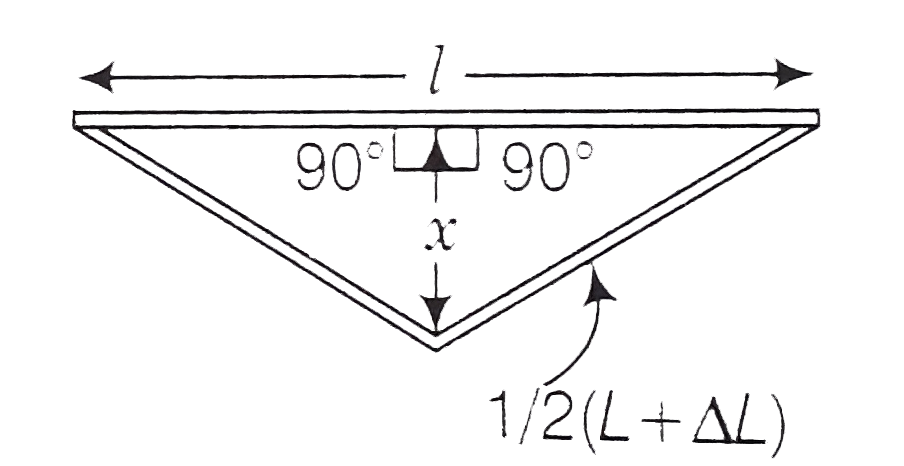
Applying Pythagorus theorem in right angled triangle in figure
${(\frac{L + Δ L}{2})}^{2} = {(\frac{L}{2})}^{2} + x^{2}$
$\Rightarrow x = \sqrt{{(\frac{L + Δ L}{2})}^{2} - {(\frac{L}{2})}^{2}}$
$= \frac{1}{2} \sqrt{{(L + Δ L)}^{2} - L^{2}}$
$= \frac{1}{2} \sqrt{(L^{2} - Δ L^{2} + 2 L Δ L) - L^{2}}$
$= \frac{1}{2} \sqrt{(Δ L^{2} + 2 L Δ L)}$
As increase in length $Δ L$ is very small, therefore, neglecting ${(Δ L)}^{2}$ , we get
$x = \frac{1}{2} \times \sqrt{2 L Δ L}$ ...(i)
But $Δ L = L α Δ t$ ...(ii)
Substituting value of $Δ L$ in Eq. (i) from Eq. (ii)
$x = \frac{1}{2} \sqrt{2 L \times L α Δ t} = \frac{1}{2} L \sqrt{2 α Δ t}$
$= \frac{10}{2} \times \sqrt{2 \times 1.2 \times 10^{- 5} \times 20}$
$= 5 \times \sqrt{4 \times 1.2 \times 10^{- 4}}$
$= 5 \times 2 \times 1.1 \times 10^{- 2} = 0.11 m = 11 c m$
Note Here we have assumed $Δ L$ to be very small so that it can neglected compared to L.

Updated on:21/07/2023

A rail track made of steel having length $10 m$ is clamped on a railway line at its two end (figure). On a summer day due to rise in temperatue by $20^{\circ} C$ . It is deformed as shown in figure. Find $x$ (displacement of the centre) if $α_{steel} = 1.2 \times 10^{- 5} /^{\circ} C$

A $15 \sqrt{30} \times 10^{- 3} c m$
B $10 \sqrt{30} \times 10^{- 3} c m$
C $25 \sqrt{30} \times 10^{- 3} c m$
D $20 \sqrt{30} \times 10^{- 3} c m$
A steel wire of length $1 m$ and mass $0.1 k g$ and having a uniform cross-sectional area of $10^{- 6} m^{2}$ is rigidly fixed at both ends. The temperature of the wire is lowered by $20^{\circ} C$ . If the wire is vibrating in fundamental mode, find the frequency (in Hz). $(Y_{s t e e l} = 2 \times 10^{11} N / m^{2}, α_{s t e e l} = 1.21 \times 10^{- 5} / .^{\circ} C)$ .
A11
B20
C15
D10
A rod has been laid by using alternate rails of steel and aluminium each $12 m$ long in a winter season when temperature is $. - 2^{\circ} C$ . The maximum temprature which can must be left be-tween advance rules of they are just to touch on a summer day
$(α_{steel} = 1.2 \times 10^{- 5}^(0) C^{- 1} α_{A l} = 2.4 \times 10^{- 5}^(0) C^{- 1}$
A $0.6 c m$
B $1.2 c m$
C $0.95 c m$
D $0.86 c m$