We would like to make a vessel whose volume does not change with temperature . We can use brass and iron at create a volume of 100 c c. How do you think you can achieve this.
In the previous problem the difference in the length was constant.
In this problem the difference in volume is constant.
The situation is shown in the diagram.
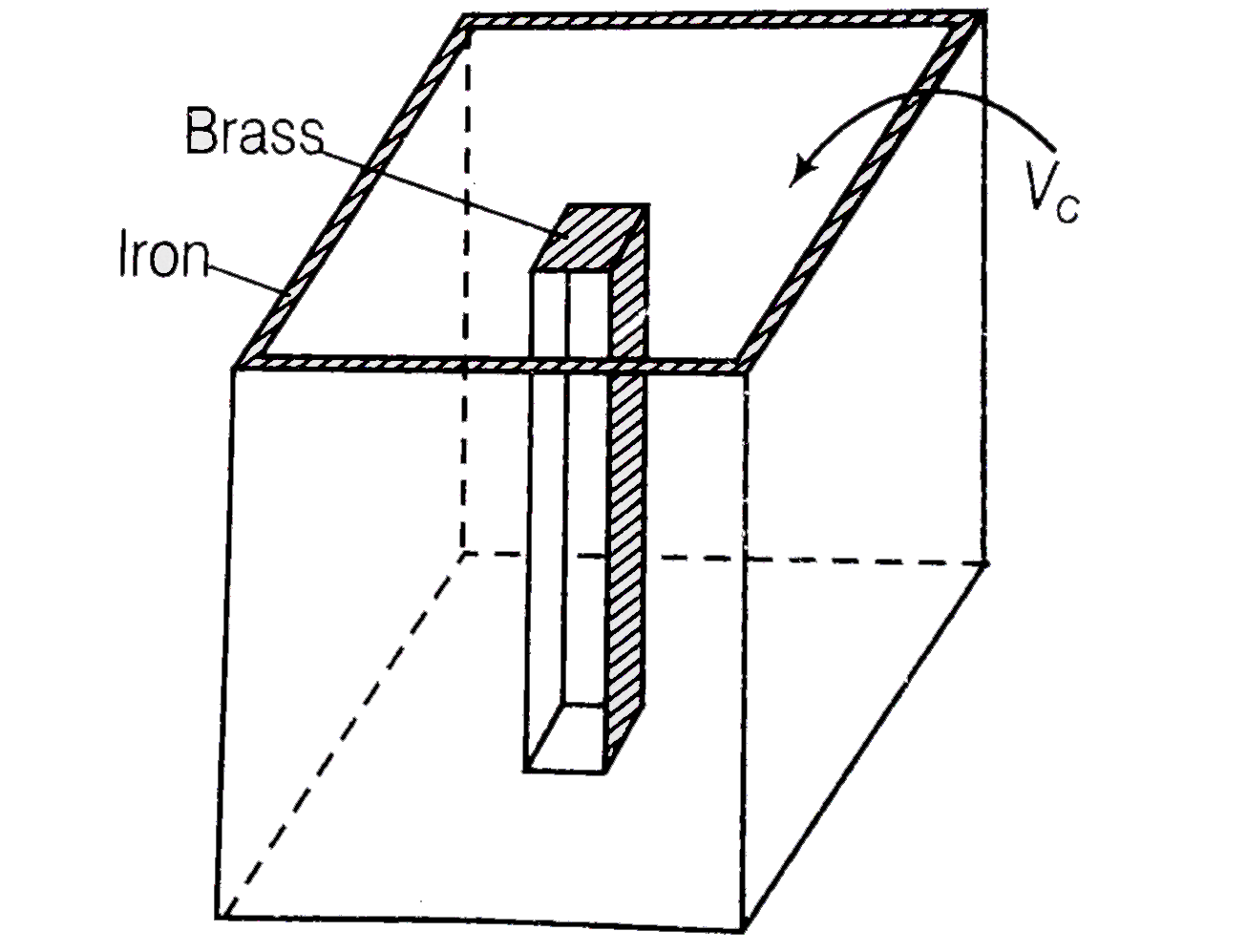
Let be the volume of iron and brass vessel at
be the volume of iron and brass vessel at ,
be the coefficient, of volume expansion of iron and brass.
As per question, ...(i)
Now,
Since, = constant.
So,
...(ii)
Solving Eqs. (i) and (ii), we get
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - NCERT EXEMPLARCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Very short Answer type Questions
15 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NCERT EXEMPLARCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - very short answer type questions
18 Videos
- THERMODYNAMICS BOOK - NCERT EXEMPLARCHAPTER - THERMODYNAMICS EXERCISE - LONG ANSWER TYPE QUESTIONS
5 Videos
Similar Questions
We would like to make a vessel whose volume does not change with temperature (take a hing from the problem aobve). We can use brass and iron (βbrass=6×10−5/Kandβiron=3.55×10−5/K) ot create a volume of 100 c c. How do you think you can achieve this.
View SolutionWe would like to perpare a scale whose length does not change with temperature. It is proposed to prepare a unit scale f this type whose length remains, say 10 cm. We can use a bimetallic strip made of brass and iron each of different length (both components) would change in such a way that differnece between theri lenght rermain constant. If αiron=1.2×10−5/Kandαbrass=1.8×10−5/K, what should we take as lenght of each strip ?
View Solution
Knowledge Check
The approximate number of air molecules in a 1 m3 volume at room temperature (300 K) and atmospheric pressure is (Use R=8.2×10−5m3 atm/mol K and NA=6.02×1023 mol^(-1))
A41B450C2.4×1025D2.7×1026How much should the temperature of a brass rod be increased so as to increase its length 3% (α for brass is 2×10−5/∘C−1)?
A1500∘CB150∘CC2000∘CD2500∘CConsider a brass rod and a steel rod (80 cm longer than brass rod) at 0∘C. It is observed that on increasing temperatures of the two rods by same amount difference in lengths of the two rods does not change. Given that the thermal coefficient of linear expansion for steel and brass are 11×10−6.∘C−1 and 19×10−6.∘C−1 respectively. The sum of lengths of the two rods at 0∘C is
A2 mB4 mC3 mD1.5 m
What is the percentage change in length of 1m iron rod it its temperature changes by 100∘C.α for iron is 2×10−5/∘C.
View SolutionWhat length of brass and iron at 00C must be used if the difference between their lengths is always 0.2m? The values of α for brass and iron and 18×10−6/0C and 12×10−6/0C respectively,
View SolutionEnds of two wires A and B, having resistivityρA=3×105Ωm and ρB=6×10−5ωm of same cross section ara are pointed together to form a single wire. If the resistance of the joined wire does not change with temperature, then find the ratio of their lengths given that temperatue coefficient of resistitivy of wires A and B are α=4×10−5/∘C and α=−4×106/∘C . Assume that mechanical dimensions do not change with temperature.
View SolutionA glass vessel is filled up to 3/5th of its volume by mercury. If the volume expansivities of glass and mercury be 9×10−6/∘C and 18×10−5/∘C respectively, then the coefficient of apparent expansion of mercury is
View SolutionAn iron rod and another of brass, both at 27∘C differ in length by 10−3m. The coefficient of linear expansion for iron is 1.1×10−5/∘C and for brass is 1.9×10−5/∘C. The temperature at which both these rods will have the same length is
View Solution
