Two capacitors of capacitance 2μFand5μF are charged to a potential difference 100V and 50 V respectively and connected such that the positive plate of one capacitor is connected to the negative plate of the other capacitor after the switch is closed, the initial current in the circuit is 50 mA. the total resistance of the connecting wires is (in Ohm):
100
300
1000
3000
The correct Answer is:D
To solve the problem step by step, we will analyze the situation involving the two capacitors and apply Kirchhoff's Voltage Law (KVL) to find the total resistance in the circuit.
Step 1: Understand the Capacitors and Their Voltages
We have two capacitors:
- Capacitor 1 (C1): 2 µF charged to 100 V
- Capacitor 2 (C2): 5 µF charged to 50 V
Step 2: Determine the Initial Voltage Across the Circuit
When the capacitors are connected such that the positive plate of C1 is connected to the negative plate of C2, the total voltage across the circuit will be the difference in their voltages:
- Voltage across C1 = 100 V (positive terminal)
- Voltage across C2 = 50 V (negative terminal)
The effective voltage (V_total) in the circuit after connecting the capacitors is:
Vtotal=VC1−VC2=100V−50V=50V
Step 3: Use Kirchhoff's Voltage Law (KVL)
According to KVL, the sum of the potential differences in a closed loop is equal to the sum of the voltage drops across the resistances. In this case, we have:
Vtotal=I⋅R
Where:
- Vtotal=50V
- I=50mA=50×10−3A
- R is the total resistance we need to find.
Step 4: Substitute the Known Values
Substituting the known values into the KVL equation:
50V=(50×10−3A)⋅R
Step 5: Solve for Resistance (R)
Rearranging the equation to solve for R:
R=50V50×10−3A
R=500.05
R=1000Ω
Step 6: Conclusion
The total resistance of the connecting wires is:
R=1000Ω
We have two capacitors:
- Capacitor 1 (C1): 2 µF charged to 100 V
- Capacitor 2 (C2): 5 µF charged to 50 V
When the capacitors are connected such that the positive plate of C1 is connected to the negative plate of C2, the total voltage across the circuit will be the difference in their voltages:
- Voltage across C1 = 100 V (positive terminal)
- Voltage across C2 = 50 V (negative terminal)
Vtotal=VC1−VC2=100V−50V=50V
According to KVL, the sum of the potential differences in a closed loop is equal to the sum of the voltage drops across the resistances. In this case, we have:
Vtotal=I⋅R
Where:
- Vtotal=50V
- I=50mA=50×10−3A
- R is the total resistance we need to find.
Substituting the known values into the KVL equation:
50V=(50×10−3A)⋅R
Rearranging the equation to solve for R:
R=50V50×10−3A
R=500.05
R=1000Ω
The total resistance of the connecting wires is:
R=1000Ω
Similar Questions
Two capacitors of capacitance 10 μF and 20 μF are charged to potential 20 V and 10 V respectively. If they are connected to share charges, then loss of energy is
View SolutionTwo capacitor of capacitance 2μFand3μF respectively are charged to potential difference 20 V each. Now the capacitors are connected such that the positively chargd plate of one capacitory is connected to the negatively charged plate of the other. after the current in the circuit has become negligible, the potential difference across the 2μF capacitor is ____V.
View Solution
Knowledge Check
Two capacitors of capacitances 3μF and 6μF are charged to a potential of 12V each. They are now connected to each other, with the positive plate of each joined to the negative plate of the other. The potential difference across each will be
A6 voltB4 voltC3 voltDZeroTwo capacitors of capacitance 2μF and 4μF respectively are charged to a potential of 12 V. they are now connected to each other, with the positive plate of each joined to the negative plate of the other. The potential difference across each capacitor will be
A2 VB3VC4VD6VTwo identical capacitors are joined in parallel, charged to a potential V and then separated and then connected in series i.e. the positive plate of one is connected to negative of the other
AThe charges on the free plates connected together are destroyedBThe charges on the free plates are enhancedCThe energy stored in the system increasesDThe potential difference in the free plates becomes 2V
Two capacitors of capacitance 2μF and 3μF respectively are charged to potential difference 20 V and 40 V respectively. Now the capacitors are connected in series with a resistance such that the positively charged plate of one capacitor is connected to the positively charged plate of the other. The initial current through the resistance is I0. The potential difference across the 2μF capacitor at the instant the current has reduced to I02 is_______ V.
View SolutionTwo capacitors of capacitances 3μFand6μF, are charged to potentials 2V and 5V respectively. These two charged capacitors are connected in series. Find the potential across each of the two capacitors now.
View SolutionThree capacitors of capacitances 1μF,2μF, and 2μF, are cahrged up to the potential difference 30V,10V, and 15V, respectivly. If terminal A is connected with DC is connected with E and F is connected eith B, the find the charge flow in the ciruit and the final charge on the capacitors.
 .
.
View SolutionT Two capacitors of capacitances 1μF and 2μF are charged to potential differnces 20V and 15V as shown in. If terminals B and C connected together and terminals A and D are connected with the positive and negative ends of the battery, respectively, then find the final charges on both the capacitors.
 .
.
View Solution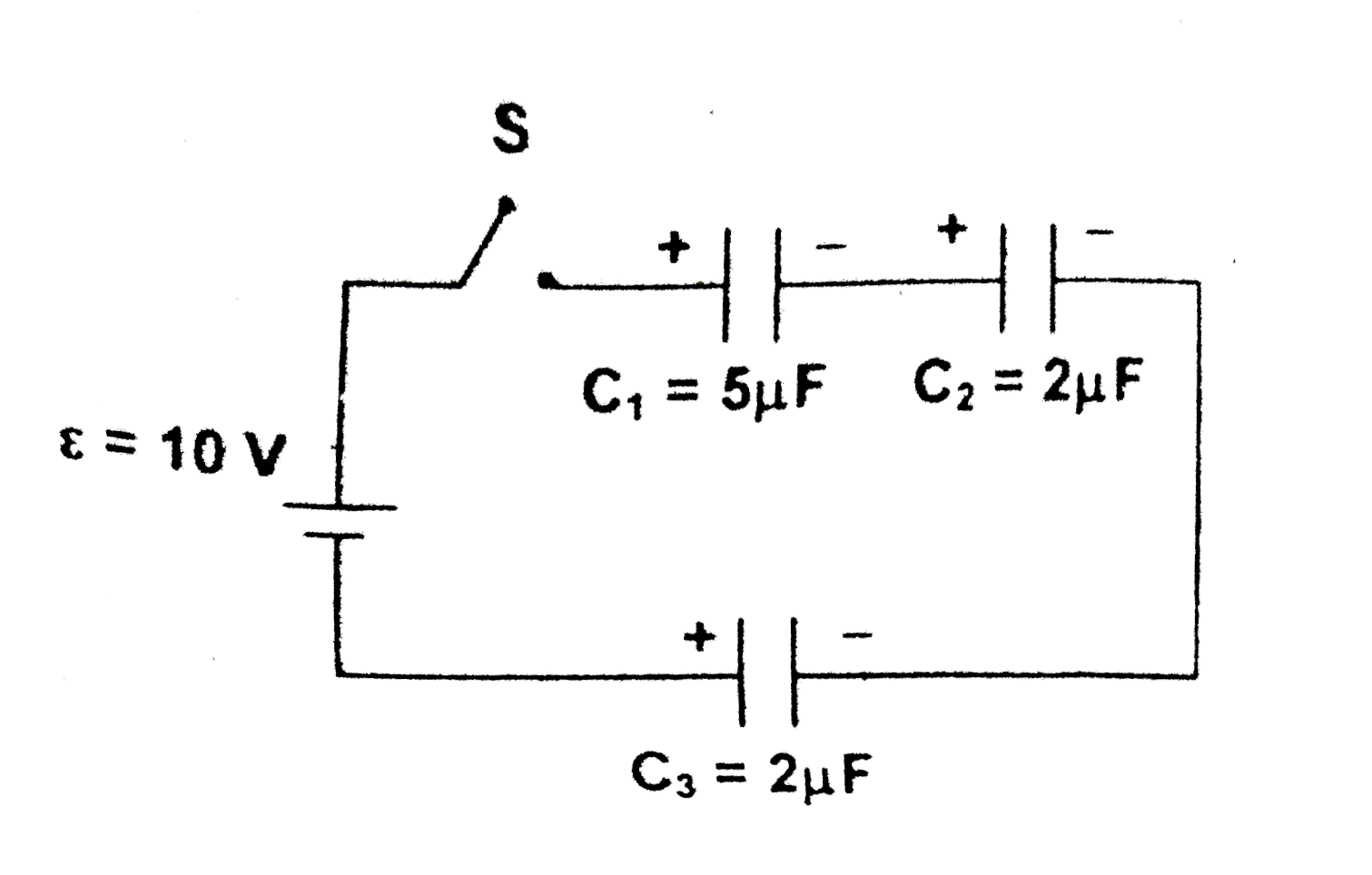
Three capacitors of capacitances 5μF,2μF and 2μF are charged to 20 V, 30 V and 10 V respectively and then connected in the circuit with polarities as shown. The magnitude of charge flown through the battery after closing the switch S is
View Solution