A container of large uniform cross sectional area A, resting on horizontal surface, holds two immiscible non viscous and incompressible liquids of density d and 2d, each of height H2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A tiny hole of area (s(s<<A) is punched on the vertical side of the container at a height h(h<H2). Determine
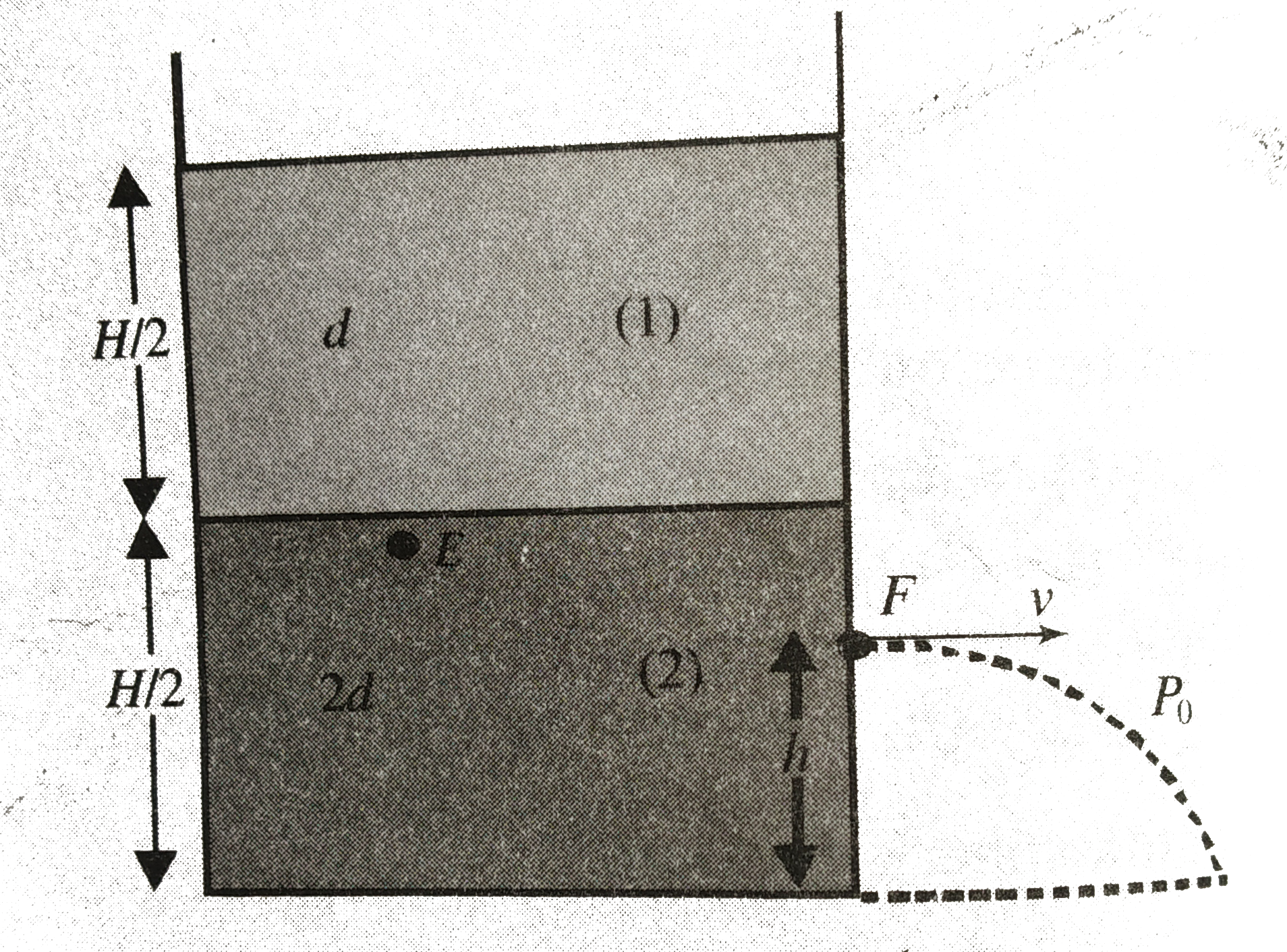
a.the initial speed of efflux of the liquid at the hole
b. the horizontal disance x travelled by the liquid initially
c. the height hm at which at the hole should be punched so that the liquid travels the maximum distance xm initially. also calculate xm (neglect air resistance in calculations).
The correct Answer is:(ii) (a) √(3H−4h)g2 (b) √h(3H−4h) (c ) hm=38H (d) xm=3H4
(ii) (a) √(3H−4h)g2 (b) √h(3H−4h) (c ) hm=38H (d) xm=3H4
(ii) (a) Applying Bernoulli’s theorem, p0+dg(H2)+2dg(H2−h)=p0+12(2d)v2
Here, v is velocity of efflux at 2. Solving this, we get v=√(3H−4h)g2
(b) Time taken to reach the liquid to the botton will be t=√2h/g
∴ Horizontal distance x travelled by the liquid is x=vt=√(3H−4h)g2(√2hg)
x=√h(3H−4h)
(c) For x to be maximum dxdh=0 or 12√h(2H−4h)(3H−8h)=0orh=3H8
Therefore, x will be maximum at h=3H8
The maximum value of x will be xm=√(3H8)[3H−4(3H8)],xm=34H
Similar Questions
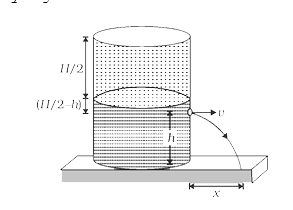
A container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscous and incompressible liquids of densities d and 2d each of height H//2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P_(0) . A homogeneous solid cylinder of length L(LltH//2) and cross-sectional area A//5 is immersed such that it floats with its axis vertical at the liquid-liquid interface with length L//4 in the denser liquid, The density D of the material of the floating cylinder is
03:51View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H//2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P_(0) . A homogeneous solid cylinder of length L(LltH//2) and cross-sectional area A//5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L//4 in the denser liquid, The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(sltltA) is punched on the vertical side of the container at a height h(hltH//2) . As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface. The initial speed of efflux without cylinder is
06:29View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H//2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P_(0) . A homogeneous solid cylinder of length L(LltH//2) and cross-sectional area A//5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L//4 in the denser liquid, The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(sltltA) is punched on the vertical side of the container at a height h(hltH//2) . As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface. The initial value of x is
08:07View SolutionA container of large uniform cross sectonal area A, resting on horizontal surface, holds two immiscible non viscous and incompresible liquids of density d and 2d, each of height H2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H2). Determine

a.the initial speed of efflux of the liquid at the hole
b. the horizontal disance x travelled by the liquid initially
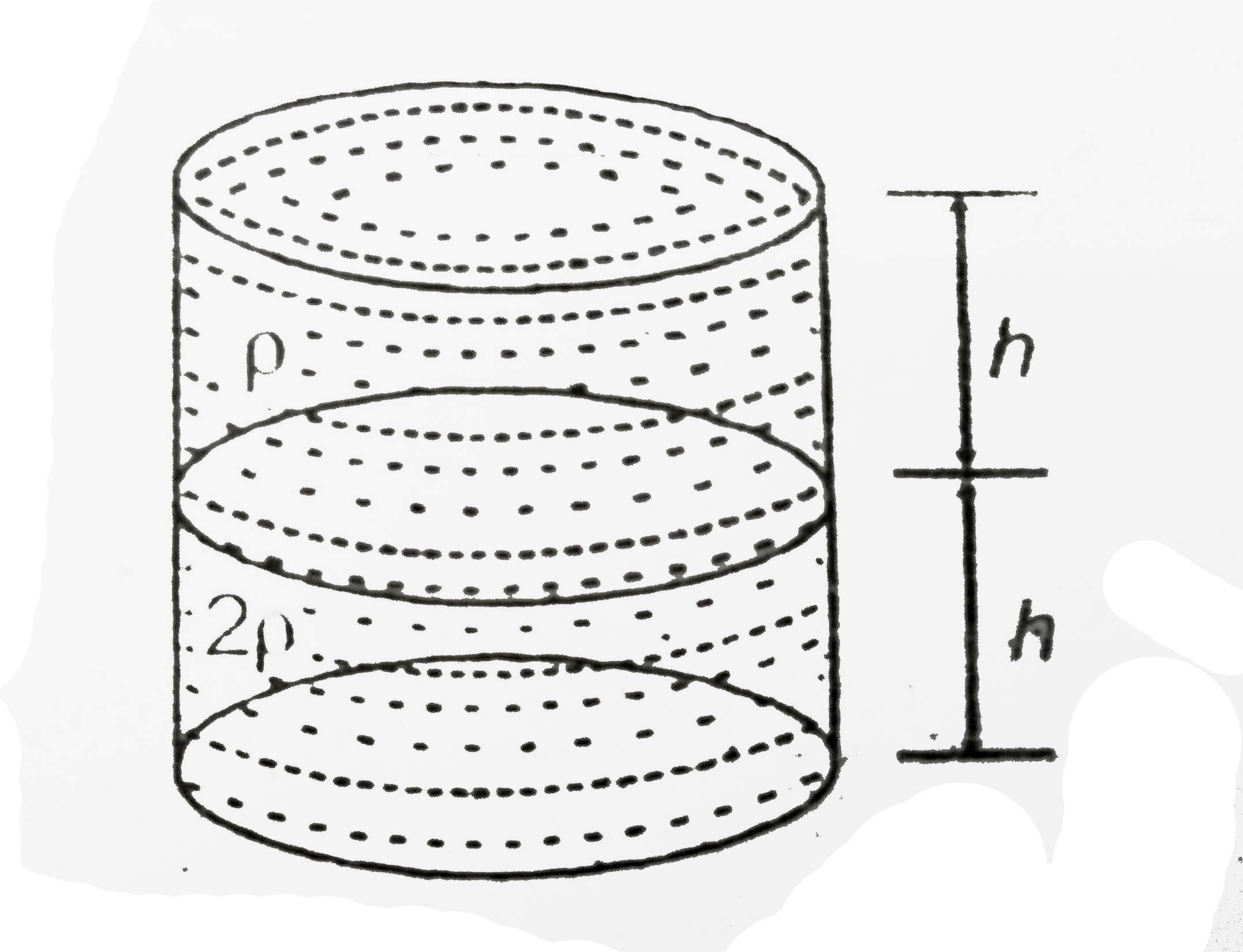
c. the height hm at which at the hole should be punched so that the liquid travels the maximum distance xm initially. also calculate xm (neglect air resistance inte calculations).05:43View SolutionA container of cross-section area A resting on a horizontal surface, holds two immiscible and incompressible liquid of densities ρand2ρ as shown in figure. The lower density liquid is open to the atmosphere having pressure P0. The pressure at the bottom of the container is
 02:02View Solution
02:02View SolutionA container of large uniform cross sectional area A , resting on horizontal surface, holds two immiscible non viscous and incompressible liquids of density d and 2d , each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P_(0) . A tiny hole of area (s(sltltA) is punched on the vertical side of the container at a height h(hltH/2) . Determine The horizontal distance traveled by the liquid, initially, is :
04:01View SolutionA container of a large uniform cross-sectional area A resting on a horizontal surface holds two immiscible, non viscous and incompressible liquids of densities d and 2d , each of height H//2 as shown in figure. The lower density liquid is open to atmosphere. A homogeneous solid cylinder of length L(L lt (H)/(2)) , cross-sectional area A//5 is immersed such that it floats with its axis vertical of the liquid-liquid interface with length L//4 denser liquid. Determine (a) density D of the solid and (b) the total pressure at the bottom of the container. (Atmospheric pressure = P_0 ). .
05:10View SolutionA container of large uniform cross sectional area A , resting on horizontal surface, holds two immiscible non viscous and incompressible liquids of density d and 2d , each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P_(0) . A tiny hole of area (s(sltltA) is punched on the vertical side of the container at a height h(hltH/2) . Determine the initial speed of efflux of the liquid at the hole
02:48View Solution
