A thin rod of length L is lying along the x-axis with its ends at x = 0 and x = L. Its linear density (mass/length) varies with x as k(xL)n where n can be zero or any positive number. If the position XCM of the centre of mass of the rod is plotted against n, which of the following graphs best approximates the dependence of XCM on n?
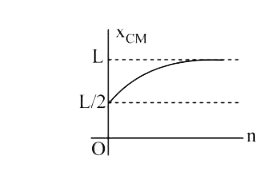
The correct Answer is:A
To find the position of the center of mass XCM of the thin rod with a varying linear density, we will follow these steps:
Step 1: Define the linear density
The linear density of the rod is given by:
λ(x)=k(xL)n
where k is a constant, L is the length of the rod, and n can be 0 or any positive number.
Step 2: Express the mass element
The mass element dm of a small segment of the rod of length dx at position x is given by:
dm=λ(x)dx=k(xL)ndx
Step 3: Calculate the total mass of the rod
The total mass M of the rod can be calculated by integrating dm from 0 to L:
M=∫L0dm=∫L0k(xL)ndx
This simplifies to:
M=k∫L0(xnLn)dx=kLn∫L0xndx
The integral ∫L0xndx evaluates to:
∫L0xndx=Ln+1n+1
Thus, the total mass becomes:
M=kLn⋅Ln+1n+1=kLn+1
Step 4: Calculate the center of mass XCM
The center of mass XCM is given by:
XCM=1M∫L0xdm=1M∫L0x⋅k(xL)ndx
This can be simplified to:
XCM=1M⋅k∫L0xn+1Lndx=1M⋅kLn⋅Ln+2n+2
Substituting M from the previous step:
XCM=1kLn+1⋅kLn+2(n+2)Ln=Ln+2L(n+1)(n+2)=L(n+1)(n+2)
Step 5: Analyze the behavior of XCM with respect to n
The expression for the center of mass is:
XCM=L(n+1)(n+2)
As n increases, the ratio n+1n+2 approaches 1, meaning XCM approaches L.
Step 6: Graphical representation
- For n=0: XCM=L(1)(2)=L2
- As n increases, XCM approaches L.
Thus, the graph of XCM against n will start at L2 when n=0 and will asymptotically approach L as n increases.
Final Answer
The graph that best represents the dependence of XCM on n will start at L2 and approach L as n increases.
The linear density of the rod is given by:
λ(x)=k(xL)n
where k is a constant, L is the length of the rod, and n can be 0 or any positive number.
The mass element dm of a small segment of the rod of length dx at position x is given by:
dm=λ(x)dx=k(xL)ndx
The total mass M of the rod can be calculated by integrating dm from 0 to L:
M=∫L0dm=∫L0k(xL)ndx
This simplifies to:
M=k∫L0(xnLn)dx=kLn∫L0xndx
The integral ∫L0xndx evaluates to:
∫L0xndx=Ln+1n+1
Thus, the total mass becomes:
M=kLn⋅Ln+1n+1=kLn+1
The center of mass XCM is given by:
XCM=1M∫L0xdm=1M∫L0x⋅k(xL)ndx
This can be simplified to:
XCM=1M⋅k∫L0xn+1Lndx=1M⋅kLn⋅Ln+2n+2
Substituting M from the previous step:
XCM=1kLn+1⋅kLn+2(n+2)Ln=Ln+2L(n+1)(n+2)=L(n+1)(n+2)
The expression for the center of mass is:
XCM=L(n+1)(n+2)
As n increases, the ratio n+1n+2 approaches 1, meaning XCM approaches L.
- For n=0: XCM=L(1)(2)=L2
- As n increases, XCM approaches L.
The graph that best represents the dependence of XCM on n will start at L2 and approach L as n increases.
Similar Questions
A thin rod of length 6m is lying along the x-axis with its ends at x=0 and x=6m. It linear density (mass/length) varies with x as kx4. Find the position of centre of mass of rod in meters.
View SolutionA rod of length L is placed along the x-axis between x = 0 and x = L. The linear density (mass/ length) λ of the rod varies with the distance x from the origin as λ=Rx. Here, R is a positive constant. Find the position of centre of mass of this rod.
View Solution
Knowledge Check
A thin rod of length L is lying along the x-axis with its ends at x = 0 and x = L. Its linear density (mass/length) varies with x as k(xL)n where n can be zero or any positive integer. If position Xcm of centre of mass of the rod is plotted against n, which of the following graphs best approximates the dependence of xCM on n?
A B
B C
C D
D
A thin rod of length 6 m is lying along the x-axis with its ends at x=0 and x=6m. Its linear density *mass/length ) varies with x as kx4. Find the position of centre of mass of rod in meters.
A5mB3mC2mD4m
A rod of length L is placed along the x-axis between x = 0 and x = L. The linear density (mass/length) λ of the rod varies with the distance x from the origin as λ = Rx. Here, R is a positive constant. Find the position of centre of mass of this rod.
View SolutionA rod of length L is placed along the x-axis between x=0 and x=L. The linear mass density (mass/length) ρ of the rod varies with the distance x from the origin as ρ=a+bx. Here, a and b are constants. Find the position of centre of mass of this rod.
View SolutionA rod of length L is placed along the x-axis between x=0 and x=L. The linear mass density is λ such that λ=a+bx. Find the mass of the rod.
View SolutionA rod of length L is placed along the X-axis between x=0andx=L. The linear density (mass/length) ρ of the rod varies with the distance x from the origin as ρj=a+bx. a. Find the SI units of a and b. b. Find the mass of the rod in terms of a,b, and L.
View SolutionThe mass per unit length of a non- uniform rod OP of length L varies as m=k(x)/(L) where k is a constant and x is the distance of any point on the rod from end 0 .The distance of the centre of mass of the rod from end 0 is
View SolutionIf linear density of a rod of length 3m varies as λ=2+x, them the position of the centre of gravity of the rod is
View Solution
