When is liquid is delived from a volumetric pipet a small amount is typically retained in the tip. How should a student proceeds in order to deliverthe volume of liquid stated on the pipet?
Leave the small amount in the tip
Use a pipet bulb to expel the remaining droplet
Shake the pipet to dispense the amount left in the tip
Draw the liquid above the line initially to compensate for the amount that remains in the tip
The correct Answer is:A
Step by step video, text & image solution for When is liquid is delived from a volumetric pipet a small amount is typically retained in the tip. How should a student proceeds in order to deliverthe volume of liquid stated on the pipet? by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SALT ANALYSISBOOK - GRB PUBLICATIONCHAPTER - SALT ANALYSISEXERCISE - B. Dry Tests/Colour/Solubility/Heating Effects
59 Videos
- SALT ANALYSISBOOK - GRB PUBLICATIONCHAPTER - SALT ANALYSISEXERCISE - C.Class- A Subgroup-I-Acidic Radicals
50 Videos
- S BLOCK ELEMENTSBOOK - GRB PUBLICATIONCHAPTER - S BLOCK ELEMENTSEXERCISE - SUBJECTIVE TYPE
9 Videos
- THERMODYNAMICSBOOK - GRB PUBLICATIONCHAPTER - THERMODYNAMICSEXERCISE - All Questions
704 Videos
Similar Questions
Raghvan is a student of class 9 in a Chennai school. His teacher, Mr. Murthy, had just finished a lecture on various types of changes which take place even in the same substance under different experimental conditions. Mr. Murthy gave a beaker full of a common liquid to Raghav. A drop of this liquid can turn anhydrous copper sulphate blue. Mr, Murthy than asked Raghav to perform two different experiements starting with the given liquid which lead to the formation of gas/gases under different experimental conditions. He also asked Raghavan to classify these changes under different types of changes. Raghvan first arranged a distillation apparatus. He took some of the given liquid in the distillation flask and started heating it with a burner. After some time, a gas started forming and going into the condenser. The condenser cooled the gas and re-converted it into the original liquid. Raghvan then arranged an apparatus to pass electric current into the given liquid. He took the given liquid in the appropriate apparatus, acidified it by adding a little of sulphuric acid, and then passed electric current from a battery for a considerable time. He collected two gases in the two water-filled test-tubes inverted over the two electrodes of the apparatus. When the mixed these two gases and ignited this mixture very,very carefully (with the help of his teacher), he heard a little explosion and saw the drops of original liquid being formed. Raghvan both the experiments and the conclusions obtained to his teacher. Mr. Murthy was very happly
(a) What do you think was the liquid give to Raghvan by Mr. Murthy ?
(b) Which gas is formed when heat energy is applied to the liquid in the distillation flask ? What type of change occurs in this case ?
(c) Which two gases are formed when electric energy is applied to the acidified liquid in the appropriate appartus ? What type of change occurs in this case ?
(d) How does the above change (which occurs on passing electric current through acidified liquid) differ from the change which takes place when a piece of paper is burnt ?
(e) What values are displayed by Raghvan in this episode ?
View Solution(Allocation problem) A cooperative society of farmers has 50 hectare of land to grow two crops X and Y. The profit from crops X and Y per hectare are estimated as Rs 10,500 and Rs 9,000 respectively. To control weeds, a liquid herbicide has to be used for crops X and Y at rates of 20 litres and 10 litres per hectare. Further, no more than 800 litres of herbicide should be used in order to protect fish and wild life using a pond which collects drainage from this land. How much land should be allocated to each crop so as to maximise the total profit of the society?
View Solution
Knowledge Check

When pressure is increases at constant temp volume of gas decreases AB→gases,BC→vpour+liquCD→liqu
critical point: At this point all the physical propeties of liquid phase will be same as the physical properties in vapour such as, density of liquid = density of vapour
Tc or critical temp: Temperature abive which a gas can not be liquified
Pc or critical pressure: minimum pressure which must be applied at critical temp to convert the gas into liqid.
Vc or critical volume: volume occupied by one mole of gas at Tc&Pc
CRITICAL CONSTANT USING VANDERWAAL EQUATIONS:
(P+aV2m)(Vm−gb)=RT==⇒(PV2m+a)(Vm−b)=RTV2mPV3m+aVm−PbV2m−ab−RTV2m=0==⇒V3m+V2m(b+RTP)+aPVm−abP=0
since equation is cubic in Vm hence there will be three roots of equation of any temperature and pressure. At critical point all three roots will coincide and will given single value of Vm=Vc at critical point. Vander Waal equation will be
V3m−V2m(b+RTCPC)+aPCVm−abPC=0...(i)
But at critical point all three roots of the equation should be equal, hence equation should be:
Vm=Vc
(Vm−Vc)3=0
V3m−3V2mVc+3VmV2c−V3c=0..(2)
comparing with equation (1)
b+RTcPc=3Vc ...(i) aPc=3V2c ...(ii) abPc=V3c ..(iii)
From (ii) and (iii), Vc=3b
From (ii) Pc=a3V2c substituting Pc=a3(3b)2=a27b2
From (i) RTcPc=3Vc−b=9b−b=8b⇒Tc=8a27Rb
At critical point, the slope of PV curve (slope of isotherm) will be zero at all other point slope will be negative zero is the maximum value of slope.
(∂P∂Vm)TC=0..(i)∂∂Vm(∂P∂Vm)TC=0...(ii)
{Mathematically such points an known as point of inflection (where first two derivatives becimes zero)}
using the two TcPc and Vc can be calculated by

If the critical constants for a hypotentical gas are Vc=150cm3mol−1.Pc=50atm and Tc=300K. then the radius of the molecule is: [Take R=112Ltratmmol−1K−1]A(752πNA)1/3B(758πNA)1/3C(3πNA)1/3D(3256πNA)1/3
When pressure is increases at constant temp volume of gas decreases AB→gases,BC→vpour+liquCD→liqu
critical point: At this point all the physical propeties of liquid phase will be same as the physical properties in vapour such as, density of liquid = density of vapour
Tc or critical temp: Temperature abive which a gas can not be liquified
Pc or critical pressure: minimum pressure which must be applied at critical temp to convert the gas into liqid.
Vc or critical volume: volume occupied by one mole of gas at Tc&Pc
CRITICAL CONSTANT USING VANDERWAAL EQUATIONS:
(P+aV2m)(Vm−gb)=RT==⇒(PV2m+a)(Vm−b)=RTV2mPV3m+aVm−PbV2m−ab−RTV2m=0==⇒V3m+V2m(b+RTP)+aPVm−abP=0
since equation is cubic in Vm hence there will be three roots of equation of any temperature and pressure. At critical point all three roots will coincide and will given single value of Vm=Vc at critical point. Vander Waal equation will be
V3m−V2m(b+RTCPC)+aPCVm−abPC=0...(i)
But at critical point all three roots of the equation should be equal, hence equation should be:
Vm=Vc
(Vm−Vc)3=0
V3m−3V2mVc+3VmV2c−V3c=0..(2)
comparing with equation (1)
b+RTcPc=3Vc ...(i) aPc=3V2c ...(ii) abPc=V3c ..(iii)
From (ii) and (iii), Vc=3b
From (ii) Pc=a3V2c substituting Pc=a3(3b)2=a27b2
From (i) RTcPc=3Vc−b=9b−b=8b⇒Tc=8a27Rb
At critical point, the slope of PV curve (slope of isotherm) will be zero at all other point slope will be negative zero is the maximum value of slope.
(∂P∂Vm)TC=0..(i)∂∂Vm(∂P∂Vm)TC=0...(ii)
{Mathematically such points an known as point of inflection (where first two derivatives becimes zero)}
using the two TcPc and Vc can be calculated by

Identify the wrong statement related to the above graph:
 Abetween 50K and 150K temperature and pressure ranging from 10 atm to 20 atm matter may have liquid state.Bzero is the maximum value of the slope of P−V curveCIf vander waal equation of state is applicable above critical temperature then cubic equation of Vm will have one real and two imaginary roots.DAt 100K and pressure below 20 atm it has liquid state only
Abetween 50K and 150K temperature and pressure ranging from 10 atm to 20 atm matter may have liquid state.Bzero is the maximum value of the slope of P−V curveCIf vander waal equation of state is applicable above critical temperature then cubic equation of Vm will have one real and two imaginary roots.DAt 100K and pressure below 20 atm it has liquid state only
When pressure is increases at constant temp volume of gas decreases AB→gases,BC→vpour+liquCD→liqu
critical point: At this point all the physical propeties of liquid phase will be same as the physical properties in vapour such as, density of liquid = density of vapour
Tc or critical temp: Temperature abive which a gas can not be liquified
Pc or critical pressure: minimum pressure which must be applied at critical temp to convert the gas into liqid.
Vc or critical volume: volume occupied by one mole of gas at Tc&Pc
CRITICAL CONSTANT USING VANDERWAAL EQUATIONS:
(P+aV2m)(Vm−gb)=RT==⇒(PV2m+a)(Vm−b)=RTV2mPV3m+aVm−PbV2m−ab−RTV2m=0==⇒V3m+V2m(b+RTP)+aPVm−abP=0
since equation is cubic in Vm hence there will be three roots of equation of any temperature and pressure. At critical point all three roots will coincide and will given single value of Vm=Vc at critical point. Vander Waal equation will be
V3m−V2m(b+RTCPC)+aPCVm−abPC=0...(i)
But at critical point all three roots of the equation should be equal, hence equation should be:
Vm=Vc
(Vm−Vc)3=0
V3m−3V2mVc+3VmV2c−V3c=0..(2)
comparing with equation (1)
b+RTcPc=3Vc ...(i) aPc=3V2c ...(ii) abPc=V3c ..(iii)
From (ii) and (iii), Vc=3b
From (ii) Pc=a3V2c substituting Pc=a3(3b)2=a27b2
From (i) RTcPc=3Vc−b=9b−b=8b⇒Tc=8a27Rb
At critical point, the slope of PV curve (slope of isotherm) will be zero at all other point slope will be negative zero is the maximum value of slope.
(∂P∂Vm)TC=0..(i)∂∂Vm(∂P∂Vm)TC=0...(ii)
{Mathematically such points an known as point of inflection (where first two derivatives becimes zero)}
using the two TcPc and Vc can be calculated by

A scientist proposed the following equation of state P=RTVm−BV2m+CV3m. If this equation leads to teh critical behaviour then critical temperature is:A8B27RCBB8RCCB23RCDNone of these
When Manu came from school, he saw his mother talking to a person who claimed that he would polish her tarnished silver/gold bangles charging only a nominal amount of money. Out of curiosity, Manu asked the person concerned as to how would he polish the bangles ? The man showed him a slivery liquid. Using his knowledge of chemistry, Manu immediately saw through the trick and asked his mother not the get the bangles polished and requested the man to please go away. Read this passage and answer the following questions :
(i) Name the silvery liquid with with the bangles were to be rubbed.
(ii) Why did Manu ask his mother not to get the bangles polished ?
(iii) What benefit does the man derive from rubbing the silver /gold bangles with silvery liquid ?
(iv) As a student of chemistry, what initiative would you take to save the innocent women in the streets and mohallas from being cheated by quacks ?
View Solution(a) In Example 3.1, the electron drift speed is estimated to be only a few mm s−1 for currents in the range of a few amperes? How then is current established almost the instant a circuit is closed?
(b) The electron drift arises due to the force experienced by electrons in the electric field inside the conductor. But force should cause acceleration. Why then do the electrons acquire a steady average drift speed?
(c) If the electron drift speed is so small, and the electron’s charge is small, how can we still obtain large amounts of current in a conductor?
(d) When electrons drift in a metal from lower to higher potential, does it mean that all the ‘free’ electrons of the metal are moving in the same direction?
(e) Are the paths of electrons straight lines between successive collisions (with the positive ions of the metal) in the (i) absence of electric field, (ii) presence of electric field?
View SolutionClarify your elementary notions about current in a metallic conductor by answering the following queries :
(a) The electron drift speed is estimated to be only a few mms−1 for currents established almost the instant a circuit is closed?
(b ) The electron drift arises due to the force experienced by electons in the electric field inside the conductor. But force should cause acceleation. Why then do the electrons acquire a steady drift speed?
( c )If the electron drift speed is so small, and electron's charge is small, how can we still obtain large amounts of current in a conductor?
(d ) When electrons drift in a metal from lower to higher potential, does it mean that all the ''free'' electrons of the metal are moving in the same direction ?
(e ) Are the paths of electons straight lines between successive collisions (with the positive ions of the metal ) in the (i) absence of electic filed, (ii) presence of electic field.
View SolutionA system of greater disorder of molecules is more probable. The disorder of molecules is reflected by the entropy of the system.A liquid vapourises to form a more disordered gas.When a solute is present, there is additional contribution to the entropy of the liquid due to increased randomness.As the entropy of solution is higher than that of pure liquid, there is weaker tendency to form the gas.Thus, a solute (non-volatile) lowers the vapour pressure of a liquid, and hence a higher boiling point of the solution.
Similarly, the greater randomness of the solution opposes the tendency to freeze.In consequence, a lower the temperature must be reached for achieving the equilibrium between the solid (frozen solvent) and the solution.Elevation of B.Pt.(ΔTb) and depression of F.Pt.(ΔTf) of a solution are the colligative properties which depend only on the concentration of particles of the solute, not their identify.For dilute solutions. ΔTbandΔTf are proportional to the molality of the solute in the solution.
ΔTb=Kbm,Kb=Ebullioscopic constant=RT∘2bM1000ΔHvap
And ΔTf=Kfm,Kf=Cryoscopic constant=RT∘2fM1000ΔHfus (M=molecular mass of the sovent)
The values of KbandKf do depend on the properties of the solvent.For liquids, ΔHvapT∘b is almost constant.
[Troutan's Rule, this constant for most of the unassociated liquids (not having any strong bonding like Hydrogen bonding in the liquid state ) is equal to 90J/mol.]
For solutes undergoing changes of molecular state is solution (ionization or association), the observed ΔT values differ from the calculated ones using the above relations In such situations, the relationships are modified as ΔTb=iKbm,ΔTf=iKfm
where i=Van't Hoff factor, greater than unity for ionization and smaller than unity for association of the solute molecules.
To aqueous solution of Nal increasing amounts of solid HgI2 is added.The vapour pressure of the solution
View SolutionA cylinder is filled with a liquid of refractive index μ. The radius of the cylinder is decreasing at a constant rate K. The volume of the liquid inside the container remains constant at V. The observer and the object O are in a state of rest at a distance L from each other. When radius of cylinder is r the apparent velocity of the object as seen by the observer is
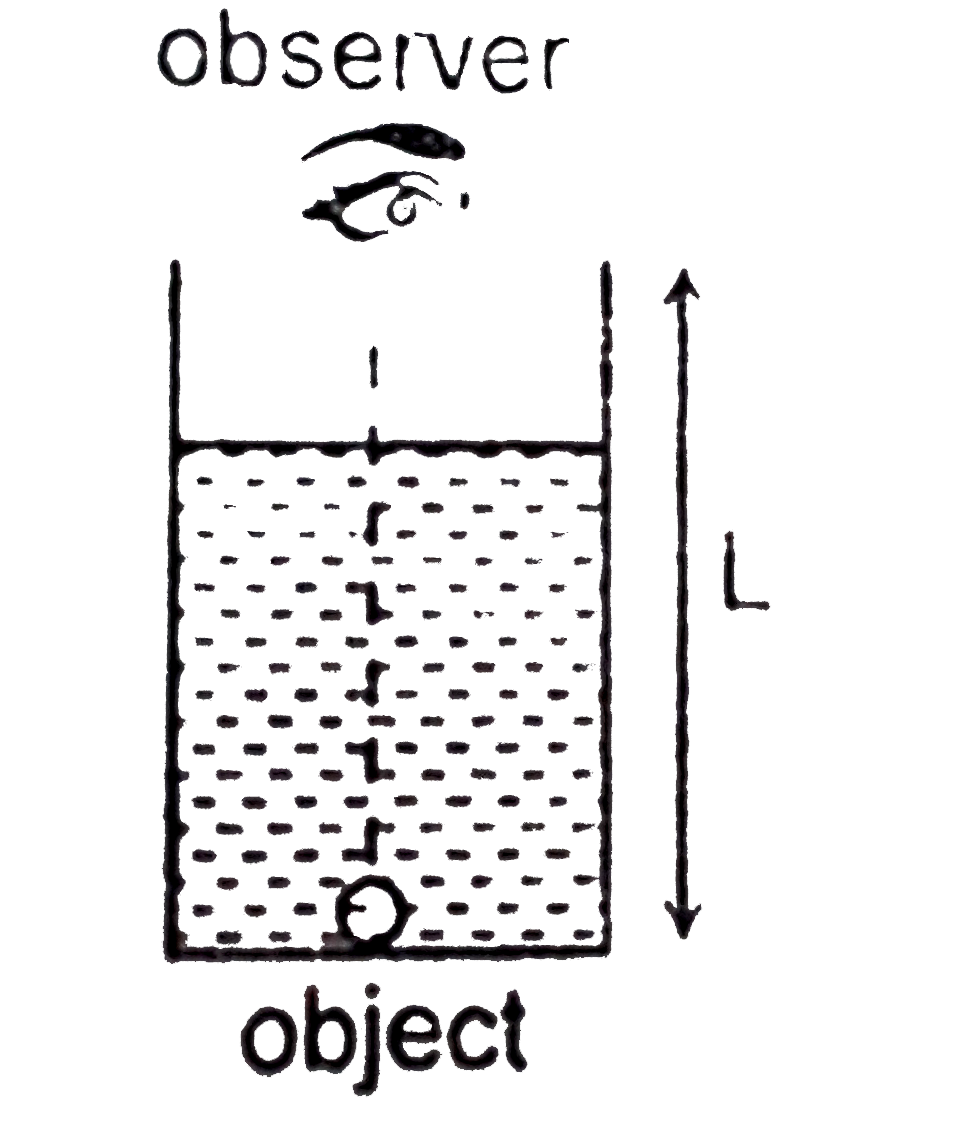
View Solution