A body mass of 1kg. Intially at rest explodes and breaks into three parts. The masses of the parts are in the ratio 1:1:3. The two pieces of equal mass fly off perpendicular to each other with a speed of 30 m/s each. The velocity of the heavier part in m/s isSolution in Telugu
10√2
6
3
6√2
Intial momentum before explosion is zero. Final momentum after explosion.
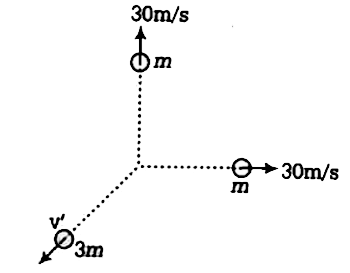
√2m4×30−3m4v'
From conservation of momentum Intial momentum = Final momentum
⇒√2m4×30−3m4v'=0
⇒ v'=10√2m/s
Topper's Solved these Questions
Similar Questions
Choose the incorrect statement from the following
View SolutionElectron microscope is based on the principle of
View SolutionA body of mass explodes at rest break up into three parts.If two parts having equal masses fly off perpendicularly to each other with a velocity of 18 m//s , then calculate the velocilty of the third part which has a mass 3 times the mass of each part.
View SolutionA body of mass 1kg initially at rest, explodes and breaks into three fragments of masses in the ratio 1:1:3. The two pieces of equal mass fly off perpendicular to each other with a speed of 15ms−1 each. What is the velocity of the heavier fragment?
View SolutionA body of mass 1 kg at rest explodes into three fragments of masses in the ratio 1 : 1 : 3 . The two pieces of equal mass fly in mutually perpendicular directions with a speed of 30 m//s each . What is the velocity of the heavier fragment ?
View SolutionA body of mass 1kg initially at rest explodes and breaks into threee fragments of masses in the ration breaks into three fragments of masses in the ration 1:1:3. The two pieces of equal masses fly off perpendicular to each other with a speed of 30m/s each What is the velocity of heavier fragments ? .
View SolutionA body of mass 1kg initially at rest explodes and breaks into three parts of masses in the ration 1:2:3. If the two pieces of equal masses fly off perpendicular to each other with a speed of 30m/s The speed of third piece will be .
View SolutionA bomb of mass 5 m initially at rest explodes and breaks into three pieces of masses in the ratio 1 : 1 : 3 . The two pieces of equal mass fly off perpendicular to each other with a speed of v_(0) . What is the velocity of the heavier piece? Also , calculate the energy released in explosion.
View SolutionA body of mass 1 kg initially at rest explodes and breaks into three fragments of masses in the ration 1 : 1 : 3. The two pieces of equal mass fly off perpendicular to each other with a speed of 30 m/s each. What is the velocity of the heavier fragment?
View SolutionA body of mass 1 kg initially at rest explodes and breaks into three fragments of masses in the ration 1 : 1 : 3. The two pieces of equal mass fly off perpendicular to each other with a speed of 30 m/s each. What is the velocity of the heavier fragment?
View SolutionA body of mass 1 kg initially at rest explodes and breaks into three fragments of masses in the ratio 1: 1: 3. The two pieces of equal mass fly off perpendicular to each other with a speed of 30ms−1 each. What is velocity of the heavier fragment?
View SolutionA body of mass explodes at rest break up into three parts. If two parts having equal masses fly off perpendicularly to each other with a velocity of 18m/s, then calculate the velocity of the third part which has a mass 3 times the mass of each part.
View SolutionA body of mass 1 kg , initially at rest, explodes and breaks into three fragments of masses in the ratio 1:1:3 . The two pieces of equal mass fly off perpendicular to each other with a speed of 30 m//s each. What is the velocity of the heavier fragment?
View SolutionA body of mass 1 kg initially at rest explodes and breaks into three fragment of masses in ratio 1 : 1 : 3. The Pieces of equal masses fly of perpendicular to each other with a speed of 30 m/s each. What is the velocity of the heavier segment.
View SolutionA body of mass 1 kg initially at rest explodes and breaks into three segments of masses in the ratio 1:1:3 . The two pieces of equal mass fly off perpendicular to each other, with a speed of 15 m/s each. The speed of the heavier fragment is
View Solution
