Range of a projectile with time of flight 10 s and maximum height 100 m is : (g=−10m/s2)
200 m
125 m
100 m
Data incoorect
The correct Answer is:D
T=10 s, H=100m
Must satisfy H=18gT2,100≠18×10×102⇒ Data is incorrect
Similar Questions
A projectile is projected from ground with an initial velocity (5ˆi+10ˆj)m/s If the range of projectile is R m, time of flight is Ts and maximum height attained above ground is Hm, find the numerical value of R×HT. [Take g=10m/s2]
View SolutionAssertion In projectile motion, if time of flight is 4s, then maximum height will be 20 m. (Take, g=10ms−2)
Reason Maximum height =gT2.View SolutionThe time of flight of a projectile is 10 s and range is 500m. Maximum height attained by it is [g=10m/s2]
View SolutionIntroduction || Time OF flight, Range, Maximum Height
View SolutionProjectile motion|| Time OF flight|| Max height|| Range
View Solution" For a projectile the ratio of the square of the time of flight to its maximum height is "
View SolutionFor a projectile if the time of flight is T, the maximum height is H and the horizontal range is R. Find the (a) the maximum height in terms of T and (b) the maximum horizontal range in terms of R and H.
View SolutionA particle is thrown vertically upward. Its velocity at half of the height is 10 m/s. Then the maximum height attained by it : -
(g=10m/s2)View SolutionFind time of flight and range of the projectile along the inclined plane as shown in figure. (g=10m/s2)
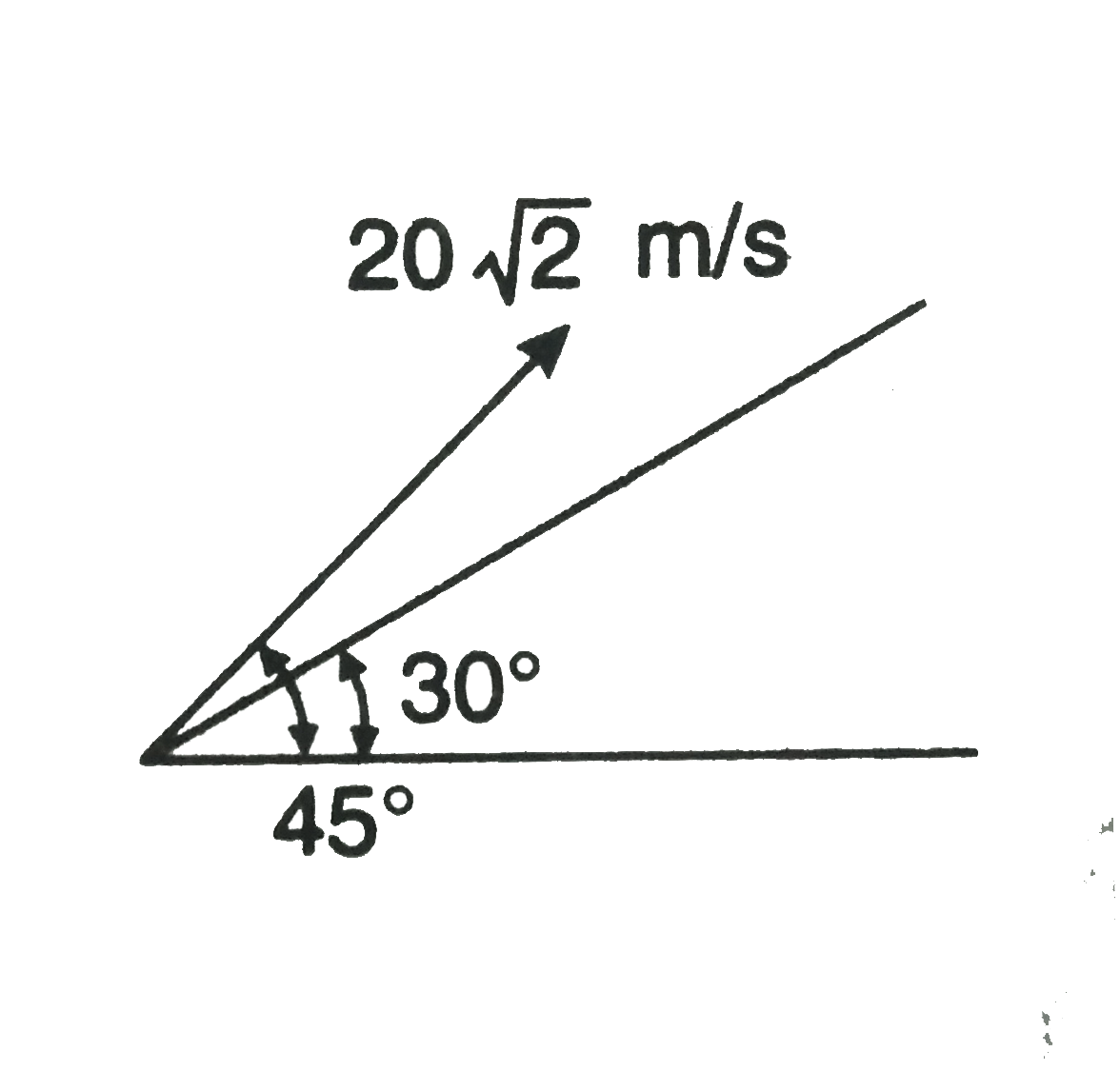 View Solution
View SolutionFind time of flight and range of the projectile along the inclined plane as shown in figure. (g=10m/s2)
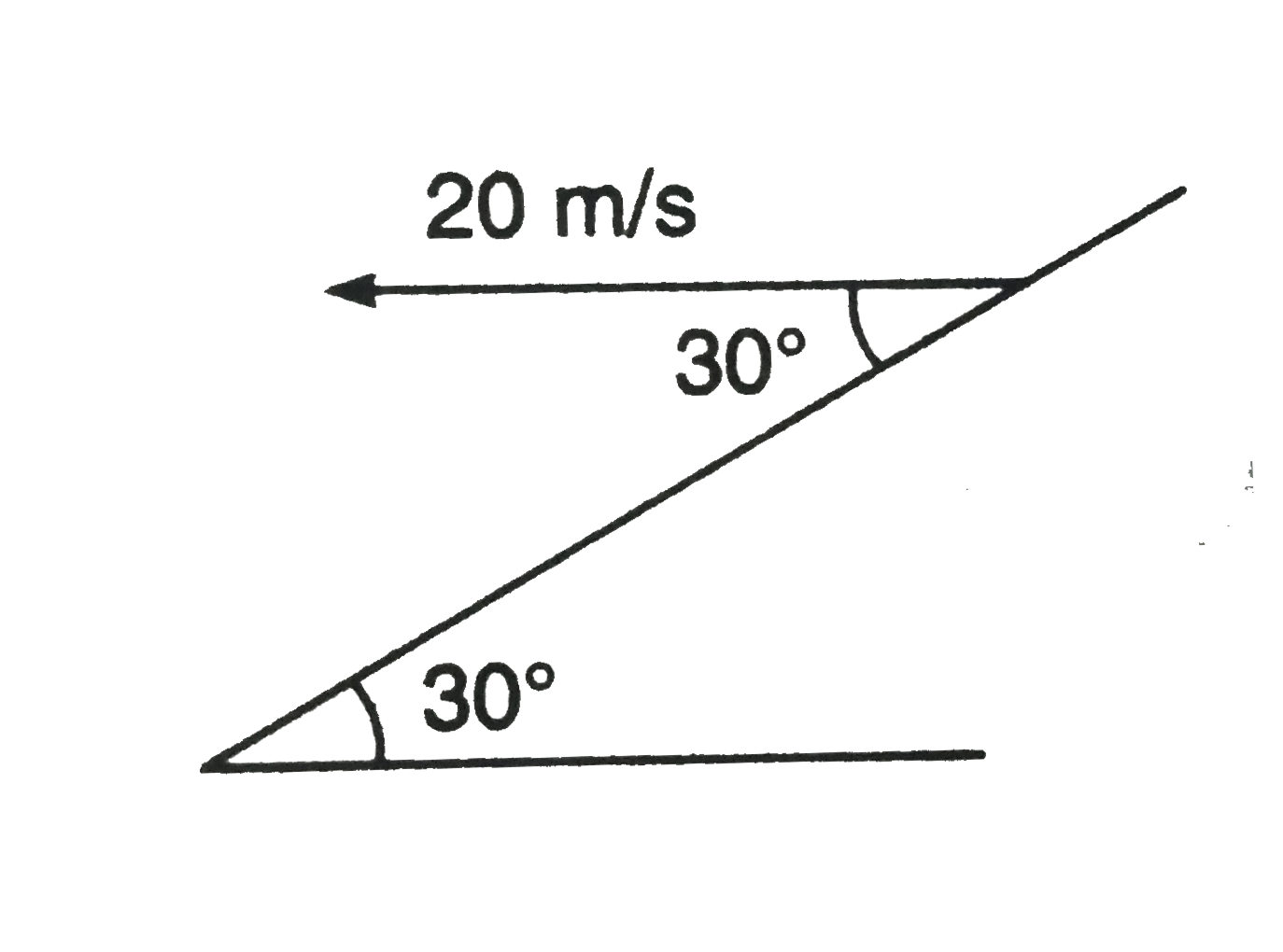 View Solution
View Solution


