The correct curve between and ) is -
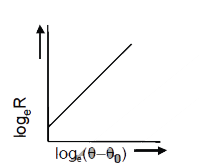
The correct Answer is:A
To solve the problem, we need to establish the relationship between the logarithm of the rate of cooling (log_e R) and the logarithm of the difference between the temperature of the body (theta) and the surrounding temperature (theta_o).
1. Understanding the Variables:
- Let R be the rate of cooling, defined as R=−dTdt (the negative sign indicates cooling).
- Let θ be the absolute temperature of the body.
- Let θo be the temperature of the surroundings.
2. Applying Newton's Law of Cooling:
- According to Newton's Law of Cooling, the rate of cooling can be expressed as:
R=k(θ−θo)
where k is a constant of proportionality.
3. Taking the Natural Logarithm:
- We take the natural logarithm (log_e) of both sides:
logeR=loge(k(θ−θo))
- Using the properties of logarithms (specifically the product rule), we can expand the right side:
logeR=logek+loge(θ−θo)
4. Rearranging the Equation:
- This can be rearranged to:
logeR=loge(θ−θo)+logek
- This is in the form of a linear equation y=mx+c, where:
- y=logeR
- x=loge(θ−θo)
- m=1 (the slope)
- c=logek (the y-intercept)
5. Analyzing the Graph:
- Since k is a positive constant, logek is also positive.
- The slope m=1 indicates that the graph is a straight line with a positive slope.
- The y-intercept is positive, confirming that the line will cross the y-axis above the origin.
6. Conclusion:
- The relationship between logeR and loge(θ−θo) is linear, with a positive slope and a positive intercept. Therefore, the correct curve is a straight line that rises from left to right.
Final Answer:
The correct curve between logeR and loge(θ−θo) is a straight line with a positive slope and a positive y-intercept.
- Let R be the rate of cooling, defined as R=−dTdt (the negative sign indicates cooling).
- Let θ be the absolute temperature of the body.
- Let θo be the temperature of the surroundings.
- According to Newton's Law of Cooling, the rate of cooling can be expressed as:
R=k(θ−θo)
where k is a constant of proportionality.
- We take the natural logarithm (log_e) of both sides:
logeR=loge(k(θ−θo))
- Using the properties of logarithms (specifically the product rule), we can expand the right side:
logeR=logek+loge(θ−θo)
- This can be rearranged to:
logeR=loge(θ−θo)+logek
- This is in the form of a linear equation y=mx+c, where:
- y=logeR
- x=loge(θ−θo)
- m=1 (the slope)
- c=logek (the y-intercept)
- Since k is a positive constant, logek is also positive.
- The slope m=1 indicates that the graph is a straight line with a positive slope.
- The y-intercept is positive, confirming that the line will cross the y-axis above the origin.
- The relationship between logeR and loge(θ−θo) is linear, with a positive slope and a positive intercept. Therefore, the correct curve is a straight line that rises from left to right.
The correct curve between logeR and loge(θ−θo) is a straight line with a positive slope and a positive y-intercept.
Topper's Solved these Questions
- HEAT TRANSFER & THERMAL EXPANSION Book:MOTIONChapter:HEAT TRANSFER & THERMAL EXPANSION Exercise:Exercise - 3 Section-A
Explore 21 Videos
- HEAT TRANSFER & THERMAL EXPANSION Book:MOTIONChapter:HEAT TRANSFER & THERMAL EXPANSION Exercise:Exercise - 3 Section-B
Explore 19 Videos
- HEAT TRANSFER & THERMAL EXPANSION Book:MOTIONChapter:HEAT TRANSFER & THERMAL EXPANSION Exercise:EXERCISE - 1
Explore 60 Videos
- HEAT - 1Book:MOTIONChapter:HEAT - 1Exercise:EXERCISE -4 (Level - II) Previous Year | JEE Advanced
Explore 22 Videos
- HEAT-2 Book:MOTIONChapter:HEAT-2 Exercise:EXERCISE-4 (LEVEL-II)
Explore 30 Videos
Similar Questions
Knowledge Check
The current curve between logejT2 and lT
A B
B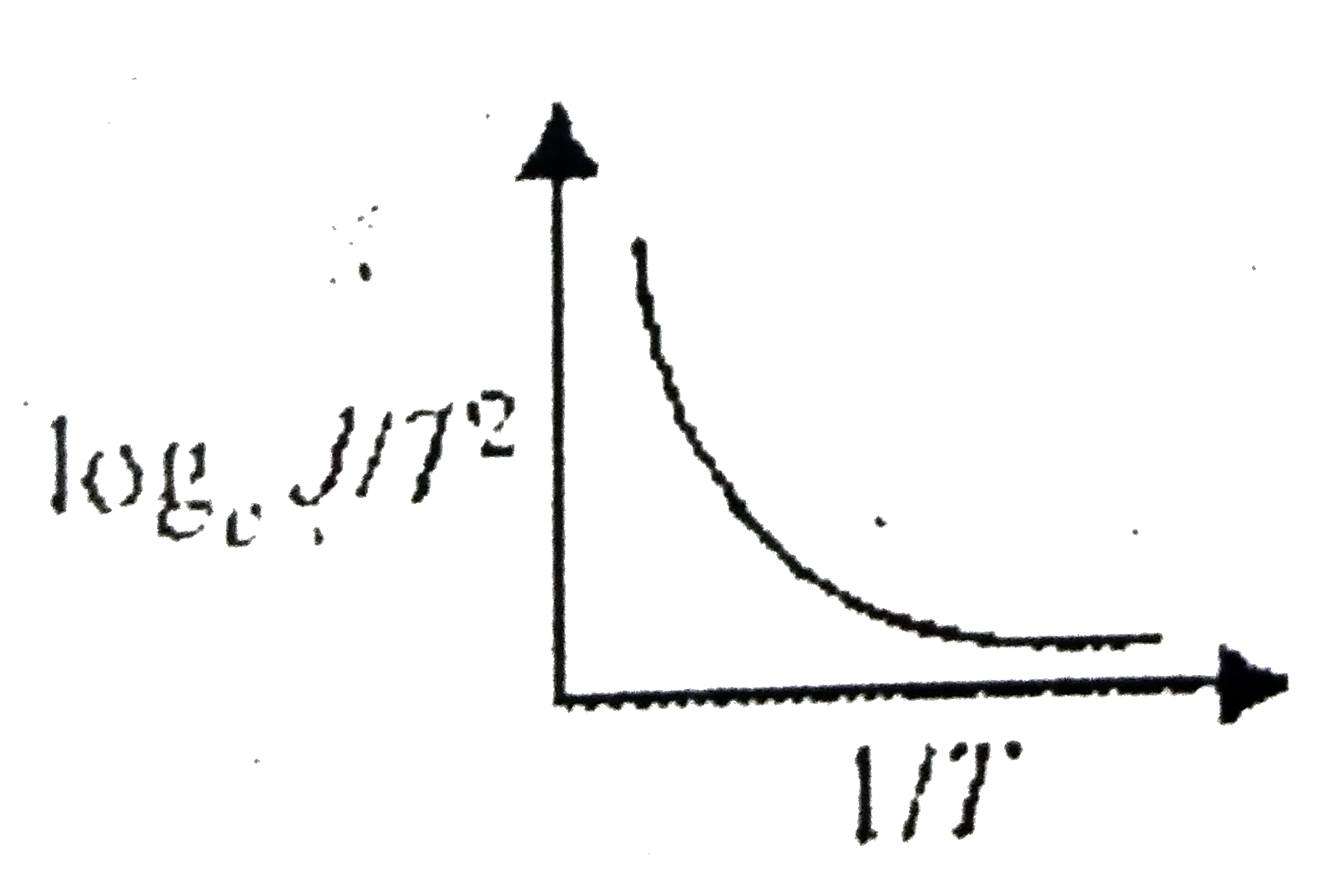 C
C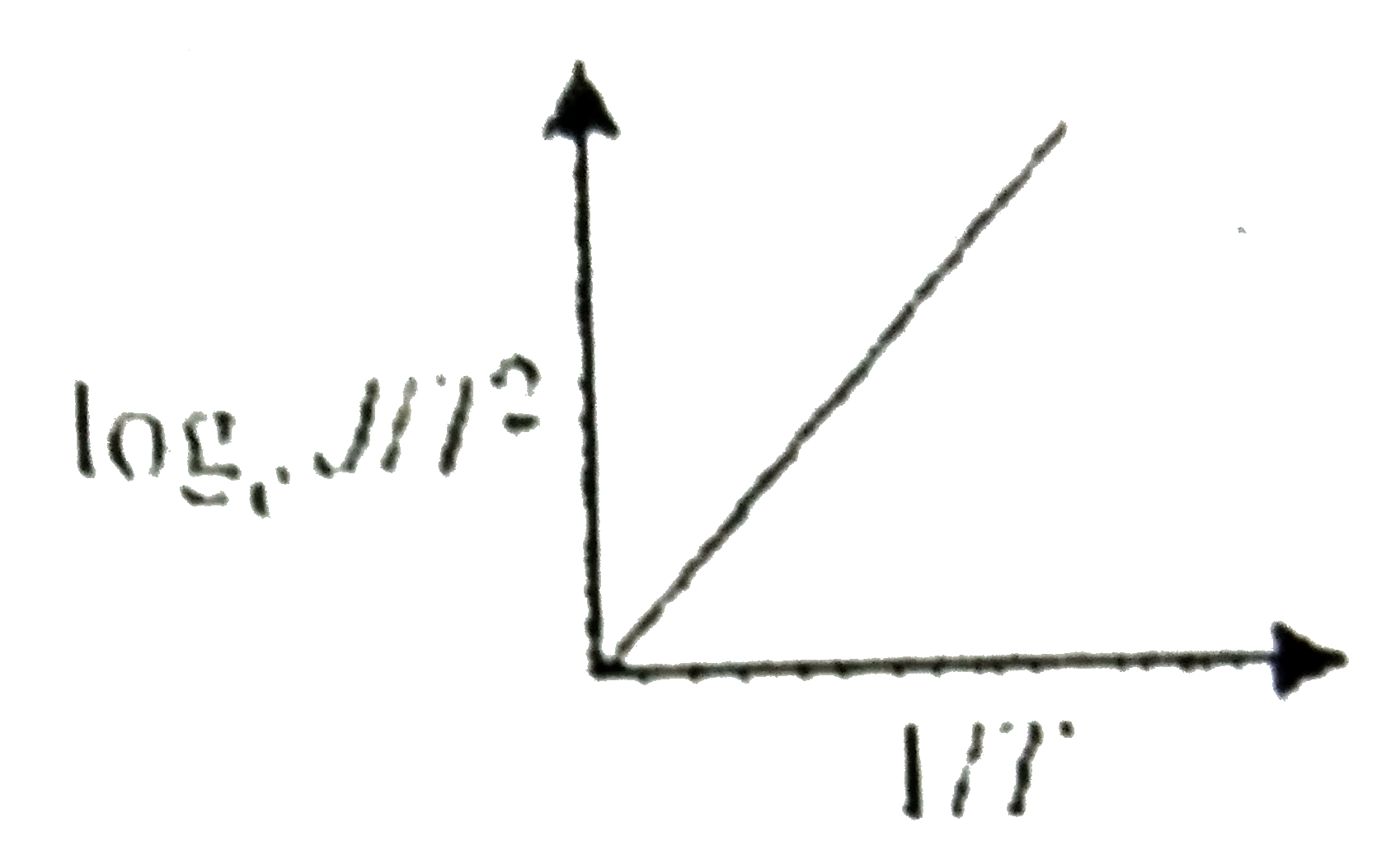 D
D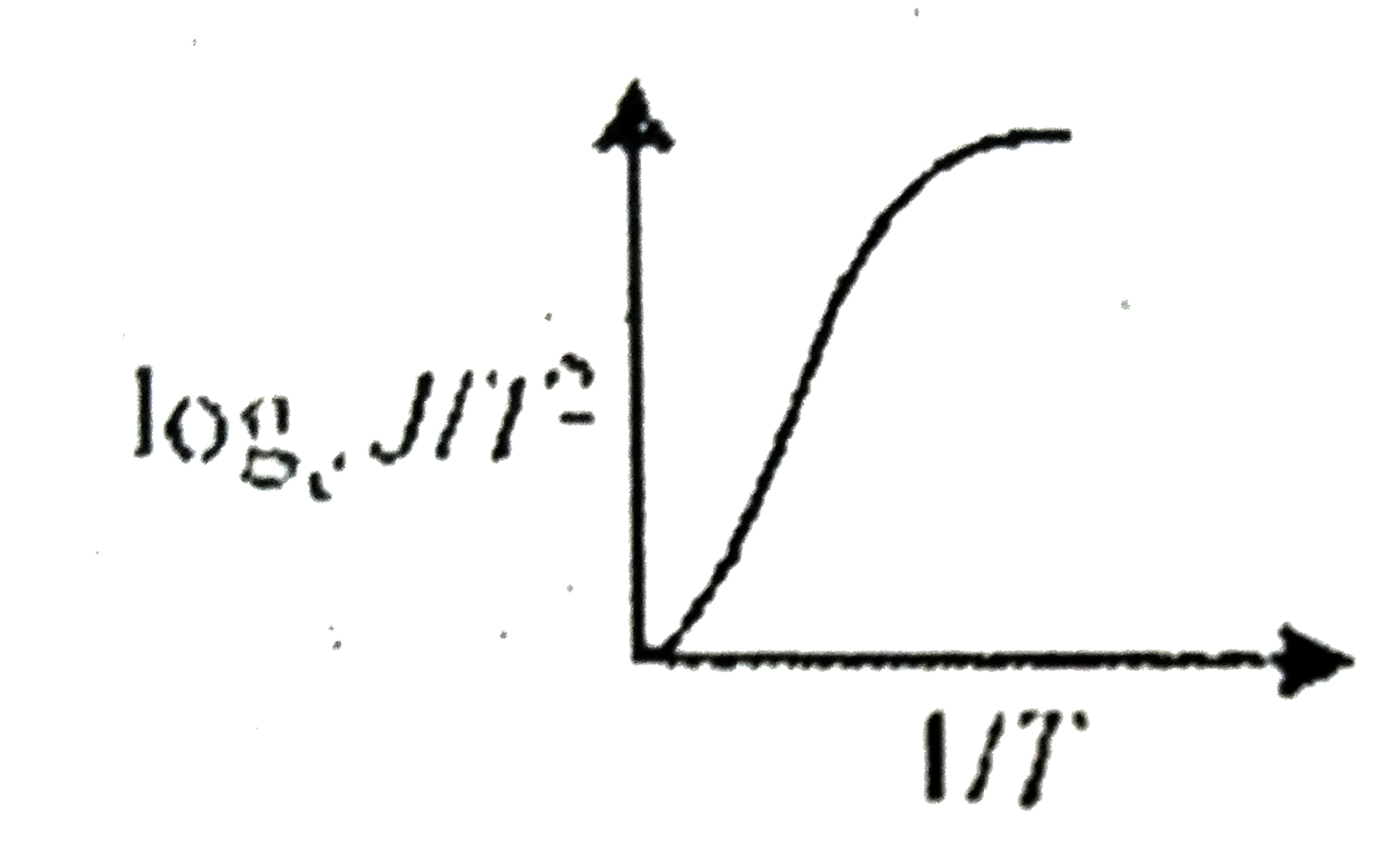
The curve between loge L and loge P is (L is the angular momentum and P is the linear momentum).
A B
B C
C D
D
A liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
A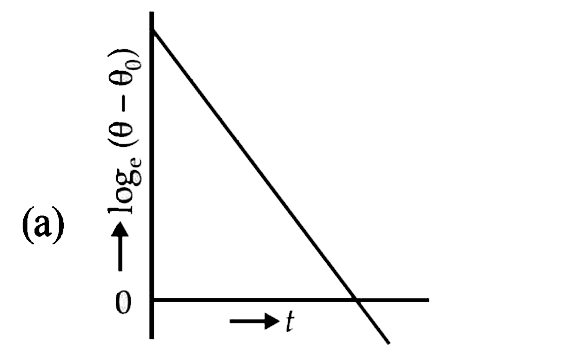 B
B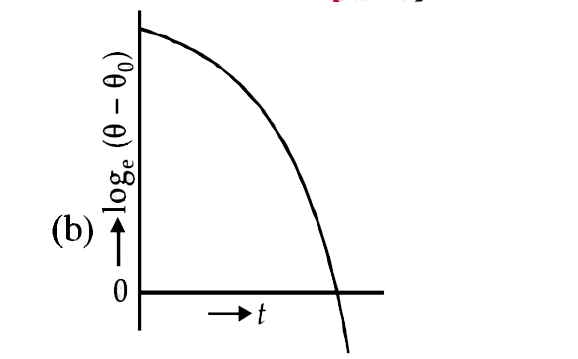 C
C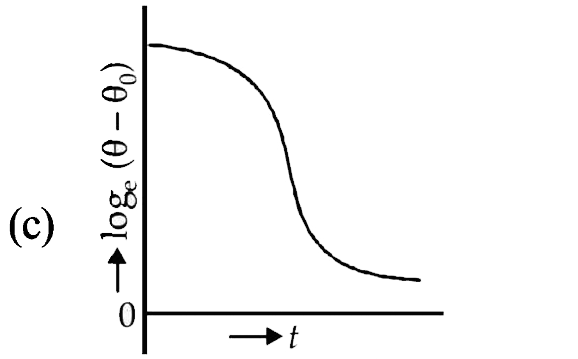 D
D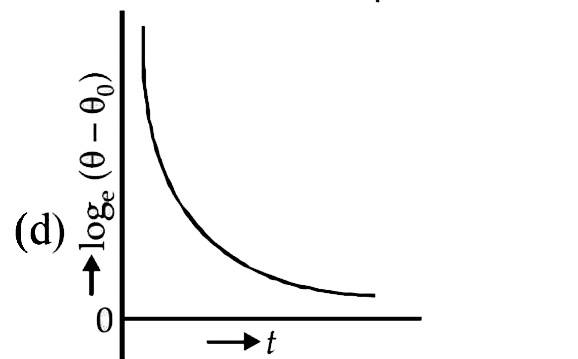
Plot the curve y=(log)e(−x).
View SolutionSketch the region bounded by the curves y=logexandy=(logex)2. Also find the area of the region.
View SolutionThe larger of cos(logθ)andlog(cosθ) if π2<θ<π2, is
View SolutionIn the above question, the curve between log I and log R will be :
View SolutionThe value of logsecθ(1−sin2θ) is ______
View Solution