A particle having mass m is projected with a velocity v0 from a point P on a horizontal ground making an angle θ with horizontal. Find out the torque about the point of projection acting on the particle when
it is at its maximum height ?
Particle is at maximum height then τ about point
P is τp=t⊥F
F = mg , r⊥=R2
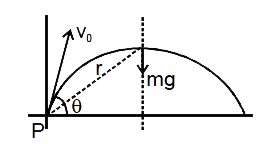
⇒τp=R2mg=mg×v20sin2θ2g
τp=mv20sin2θ2g
τp=mv20sin2θ2
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 1
90 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 2
40 Videos
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Exercise-4
38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBOOK - MOTIONCHAPTER - SEMI CONDUCTOR AND LOGIC GATESEXERCISE - EXERCISE 3
34 Videos
Similar Questions
A particle having mass m is projected with a velocity v0 from a point P on a horizontal ground making an angle θ with horizontal. Find out the torque about the point of projection acting on the particle when
It is just about to hit the ground back ?
View SolutionA particle of mass m is projected with a speed v0 at an angle of projection θ with horizontal. Find the torque of the weight of the particle about the point of projection after time v0sinθg
View Solution
Knowledge Check
A particle of mass 'm' is projected with a velocity υ making an angle of 30∘ with the horizontal. The magnitude of angular moment of the projectile about the point of projection when the particle is at its maximum height 'h' is
A√32mυ2gBzeroCmυ3√2gD√32mυ2gA particle of mass m is projected with a velocity v making an angle of 45° with the horizontal. The magnitude of the angular momentum of the projectile about the point of projection, when the particle is at its maximum height h, is
AzeroBmv34√2gCmv3√2gDm√2gh3A particle of mass m is projected with a velocity v making an angle of 45∘ with the horizontal. The magnitude of the angular momentum of the projectile abut the point of projection when the particle is at its maximum height h is.
AzeroB(mv)34√2gC(mv)3√2gDm√2gh3
A particle of mass m is projected with velocity v at an angle θ with the horizontal. Find its angular momentum about the point of projection when it is at the highest point of its trajectory.
View SolutionA body of mass m is projected with a velocity u at an angle θ with the horizontal. The angular momentum of the body, about the point of projection, when it at highest point on its trajectory is
View SolutionA particle of mass m is projecte dwilth speed u at an angle θ with the horizontal. Find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View SolutionA particle projected with velocity u at angle θ with horizontal at t=0. What is the magnitude of change in the velocity of the particle when it is at maximum height?
View SolutionA particle of mass m is projected with an initial velocity U at an angle θ to the horizontal. The torque of gravity on projectile at maximum height about the point of projection is
View Solution