The probability distribution of a random variable x is given as under
P(X=x)=⎧⎪⎨⎪⎩kx2x=1,2,32kxx=4,5,60,otherwise
where, k is a constant. Calculate
(i) E(X) (ii) E(3X2) (iii) P(X≥4)
The correct Answer is:(i) 4.32 (ii) 20.64 (iii) 15/22
Step by step video & text solution for The probability distribution of a random variable x is given as under P(X=x)={{:(kx^(2),x="1,2,3"),(2kx,x="4,5,6"),("0,","otherwise"):} where, k is a constant. Calculate (i) E(X) (ii) E(3X^(2)) (iii) P(Xge4) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
The probability distribution of a random variable X is given as follows:
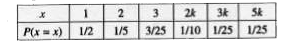
If E(X)=2.3 , then value of k isA1/2B5/4C3D2The probability distribution of a random variable (X) is P(X)={x12:X=1, 2, 3, 4, 5, 60:otherwise
Then, the conditional probability
P⎛⎝32<X<72X>2⎞⎠ isA56B518C16D712The p.m.f. of a r.v. X is P(x)={kx2,x=1,2,3,40, otherwise, then E (X) =
A103B53C1003D503
Let X be a discrete random variable whoose probability distribution is defined as follows.
P(X=x)=⎧⎪⎨⎪⎩k(x+1),for x=1,2,3,42kx, for x=5,6,70, otherwise
where,k is a constant. Calculate (i) the value of k. (ii) E (X).
(ii) standard deviation of X.
View SolutionIf the probability distribution of a random variable X is given by X=xi: 1 2 3 4 P(X=xi): 2k 4k 3k k Write the value of k .
View SolutionThe probability distribution of a random variable X is given by.
X=x:01234P(X=x):0.40.30.10.10.1
The variance of X, is
View SolutionThe p.m.f. of a r.v. X is P(x){kx2,x=1,2,3,40, otherwise, then Var (X) =
View SolutionThe p.m.f. of a r.v. X is P(x)={2kx,x=1,2,30, otherwise, then k =
View Solution

