If the probability mass function of a discrete random variable X is
P(x)=Cx3,x=1,2,3
=0, otherwise
Then E(X)=
343297
294251
297294
251294
The correct Answer is:B
To solve the problem, we need to find the expected value E(X) of the discrete random variable X given its probability mass function (PMF):
P(X=x)=Cx3,x=1,2,3
and P(X=x)=0 otherwise.
Step 1: Find the value of C
To find the value of C, we use the property that the total probability must equal 1:
P(1)+P(2)+P(3)=1
Substituting the PMF into this equation:
C13+C23+C33=1
This simplifies to:
C(1+18+127)=1
Step 2: Calculate the sum inside the parentheses
To calculate 1+18+127, we find a common denominator. The least common multiple of 1, 8, and 27 is 216.
Calculating each term:
- 1=216216
- 18=27216
- 127=8216
Now, adding these fractions:
1+18+127=216+27+8216=251216
Step 3: Solve for C
Now substituting back into the equation for C:
C⋅251216=1
Thus,
C=216251
Step 4: Calculate the expected value E(X)
The expected value E(X) is calculated using the formula:
E(X)=3∑x=1x⋅P(X=x)
Substituting the PMF:
E(X)=1⋅P(1)+2⋅P(2)+3⋅P(3)
This becomes:
E(X)=1⋅C13+2⋅C23+3⋅C33
Substituting C=216251:
E(X)=1⋅216251+2⋅216251⋅8+3⋅216251⋅27
This simplifies to:
E(X)=216251(1+28+327)
Step 5: Calculate the sum inside the parentheses
Calculating 1+28+327:
- 1=216216
- 28=54216
- 327=24216
Adding these fractions:
1+28+327=216+54+24216=294216
Step 6: Final calculation of E(X)
Now substituting back into the expected value:
E(X)=216251⋅294216
The 216 cancels out:
E(X)=294251
Thus, the expected value E(X) is:
294251
and P(X=x)=0 otherwise.
- 18=27216
- 127=8216
- 28=54216
- 327=24216
Topper's Solved these Questions
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Competitive Thinking
9 Videos
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Evaluation test
5 Videos
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Evaluation test
5 Videos
- PLANEBOOK - TARGET PUBLICATIONCHAPTER - PLANEEXERCISE - EVALUATION TEST
14 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - TARGET PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - Evaluation Test
9 Videos
Similar Questions
Knowledge Check
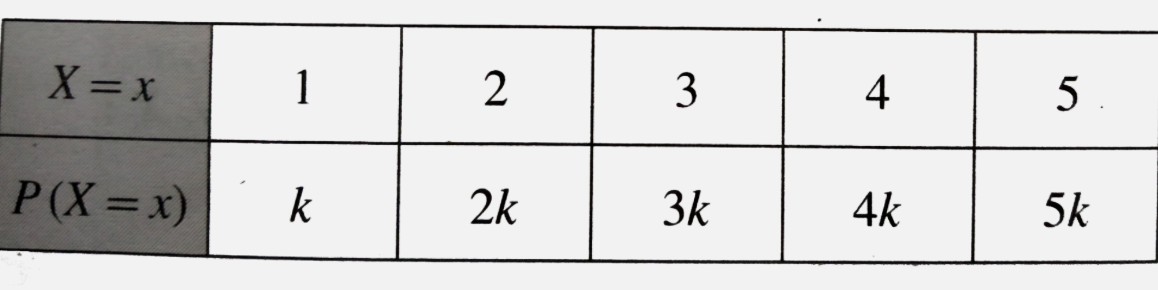
The probability distribution of a discrete random variable X is given below
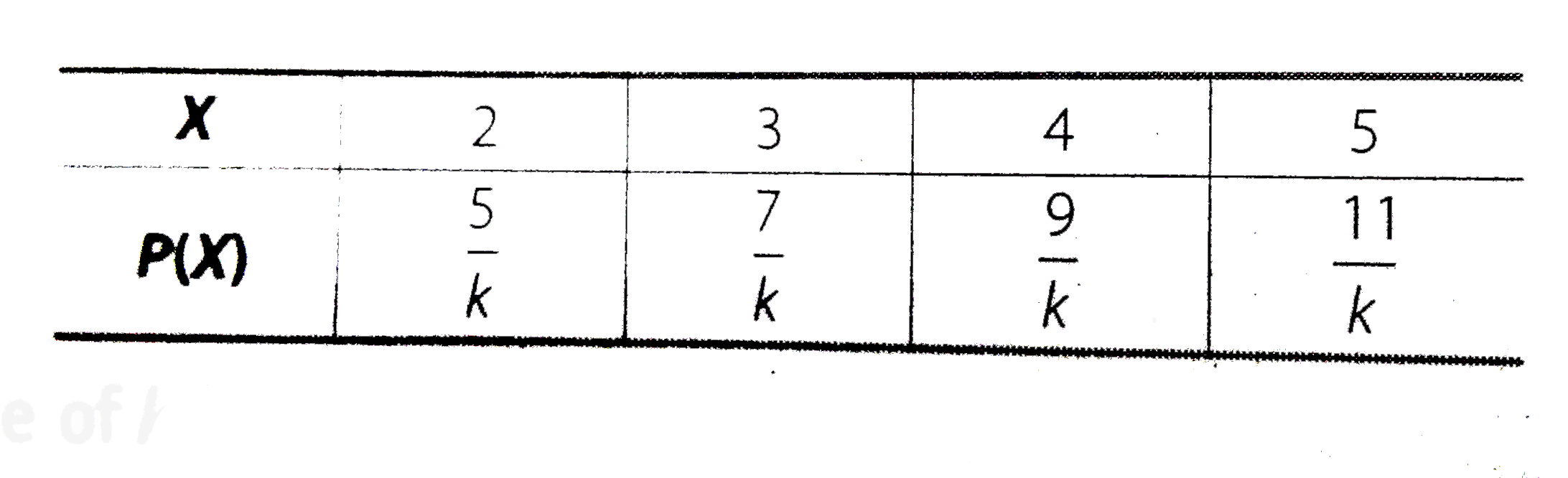
The value of k isA8B16C32D48If the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5 then the probability that X takes a prime value is
A1320B2360C1120D1960If the probability density function of a random variable X is f(x)=x2 in 0≤x≤2, then P(X>1.5∣X>1) is equal to
A716B34C712D2164
The following is the p.d.f (Probability Density Function ) of a continous random variable X :
f(x)=x32,0<x<8
= 0 , otherwise
Find the expression for c.d.f (Cumulative Distribution Function ) of X
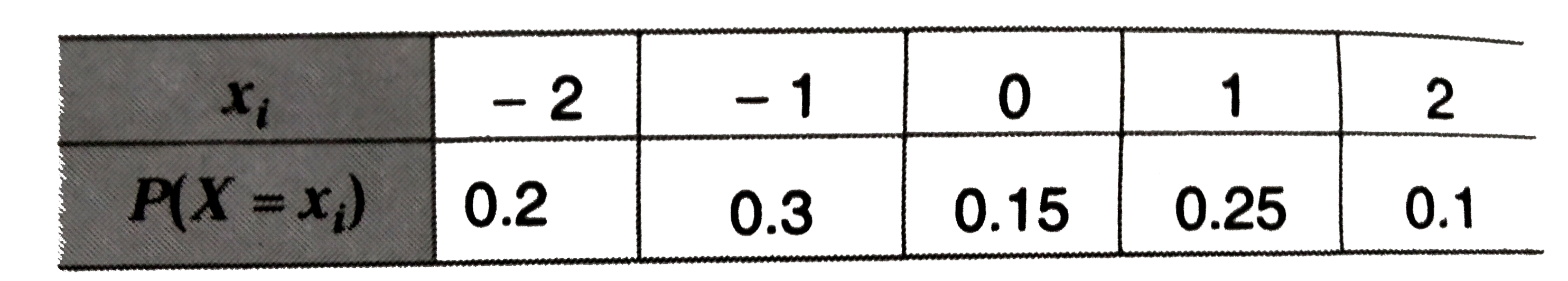
View SolutionIf the probability density function of a random variable X is given as
then F(0) is equal to
View SolutionThe probability distribution of a random variable (X) is P(X)={x12:X=1, 2, 3, 4, 5, 60:otherwise
Then, the conditional probability
P⎛⎝32<X<72X>2⎞⎠ is
View SolutionIf the probability density function of a random variable X is f(x)=π2 in 0≤x≤2, then P(X>1.5X>1) is equal to
View SolutionLet the p.m.f. of a random variable X be -
P(x)=3−x10 for x=−1,0,1,2
= 0 otherwise
Then E(X) is ………… .
View Solution