Shows two long metal rails placed horizontally and parallel to each other at a separation i. A uniform magnetic field b exists in the vertically downward direction. A wire of mass m can slide on the rails. The rails are connected to a constant current source which drives a current I in the circuirt. The friction coefficient between the rails and the wire is μ. (a) What should be the minimum value of μ which can prevent the wire from sliding on the rails? (b) Describe the motion of the wire if the value of μ is half the value found in the previous part.

The force on the wire due to the magnetic field is
→F=i→l×→B
or F=ilB
It acts towards right tin the given figure. If the wire does not slide on the rails, the force of friction by the rails should be equal to F. If μ0 be the minimum coefficient of fricition which can prevent sliding , this force is also equal to μ0 mg . Thus,
μ0mg=ilB
or , μ0=ilBmg
(b) If the friction coefficient is μ=μ02=ilB2mg,the wire will slide towards right . The frictional force by the rails is
f=μmg=ilB2 towards left.
The resultant force is ilB−ilB2=ilB2 towards
right . The acceleration will be a =ilB2m. The wire will slide towards right with this acceleration.
Topper's Solved these Questions
- MAGNETISM -1Book:MOTIONChapter:MAGNETISM -1Exercise:QUESTIONS FOR PRACTICE
Explore 34 Videos
- MAGNETISM -1Book:MOTIONChapter:MAGNETISM -1Exercise:Exercise - 1 SECTION -A :- Magnetif field due to straight wire
Explore 10 Videos
- MAGNETISM Book:MOTIONChapter:MAGNETISM Exercise:EXERCISE-4 (LEVEL-II)
Explore 40 Videos
- MATTER WAVEBook:MOTIONChapter:MATTER WAVEExercise:EXERCISE 3 (SECTION B)
Explore 7 Videos
Similar Questions
Magnetic field is produced by the flow of current in a straight wire. This phenomenon is based on-
View SolutionAlong the direction of current carrying wire, the value of magnetic field is ?
View SolutionIn a moving coil galvanometer, torque on the coil can be experessed as τ=ki, where i is current through the wire and k is constant . The rectangular coil of the galvanometer having number of turns N , area A and moment of interia I is placed in magnetic field B. Find
(a) k in terms of given parameters N,I,AandB
(b) the torsion constant of the spring , if a current i0 produces a deflection of (π)/(2) in the coil .
(c) the maximum angle through which the coil is deflected, if charge Q is passed through the coil almost instaneously. ( ignore the daming in mechinal oscillations).
View SolutionFormation of covalent bonds in compound exhibits
View SolutionShows two long metal rails placed horizontally and parallel to each other at a separation i. A uniform magnetic field b exists in the vertically downward direction. A wire of mass m can slide on the rails. The rails are connected to a constant current source which drives a current I in the circuirt. The friction coefficient between the rails and the wire is mu . (a) What should be the minimum value of mu which can prevent the wire from sliding on the rails? (b) Describe the motion of the wire if the value of mu is half the value found in the previous part.
View SolutionA straight wire of length l can slide on two parallel plastic rails kept in a horizontal plane with a separation d. The coefficient of friction between the wire and the rails is μ. If the wire carries a current I, what minimum magnetic field should exist in the space in order to slide the wire on the rails.
View SolutionA metal wire PQ of mass 10g lies at rest on two horizontal metal rails separated by 5cm as shown in Fig. A vertically downward magnetic field of magnitude 0.80T exist in the space. The resistance of the circuit is slowly decreased and it is found that when the resistance goes below 20.0Omega the wire PQ starts sliding on the rails. The coefficient of friction between wires and rails is found. The coefficient of friction between wires and rails is found to be n//25 . find n
View SolutionA metal wire of mass m slides without friction on two horizontal rails spaced distance d-apart as shown in figure. The rails are situated in a uniform magnetic field B, directed vertically upwards, and a battery is sending a current I through them. Find the velocity of the wire as a function of time, assuming it to be at rest initially.
View SolutionFigure shows a wire sliding on two parallel, conducting rails placed at a separation L . A magnetic field B exists in a direction perpendicular to the plane of the rails. What force is necessary to keep the wire moving at a constant velocity V ?
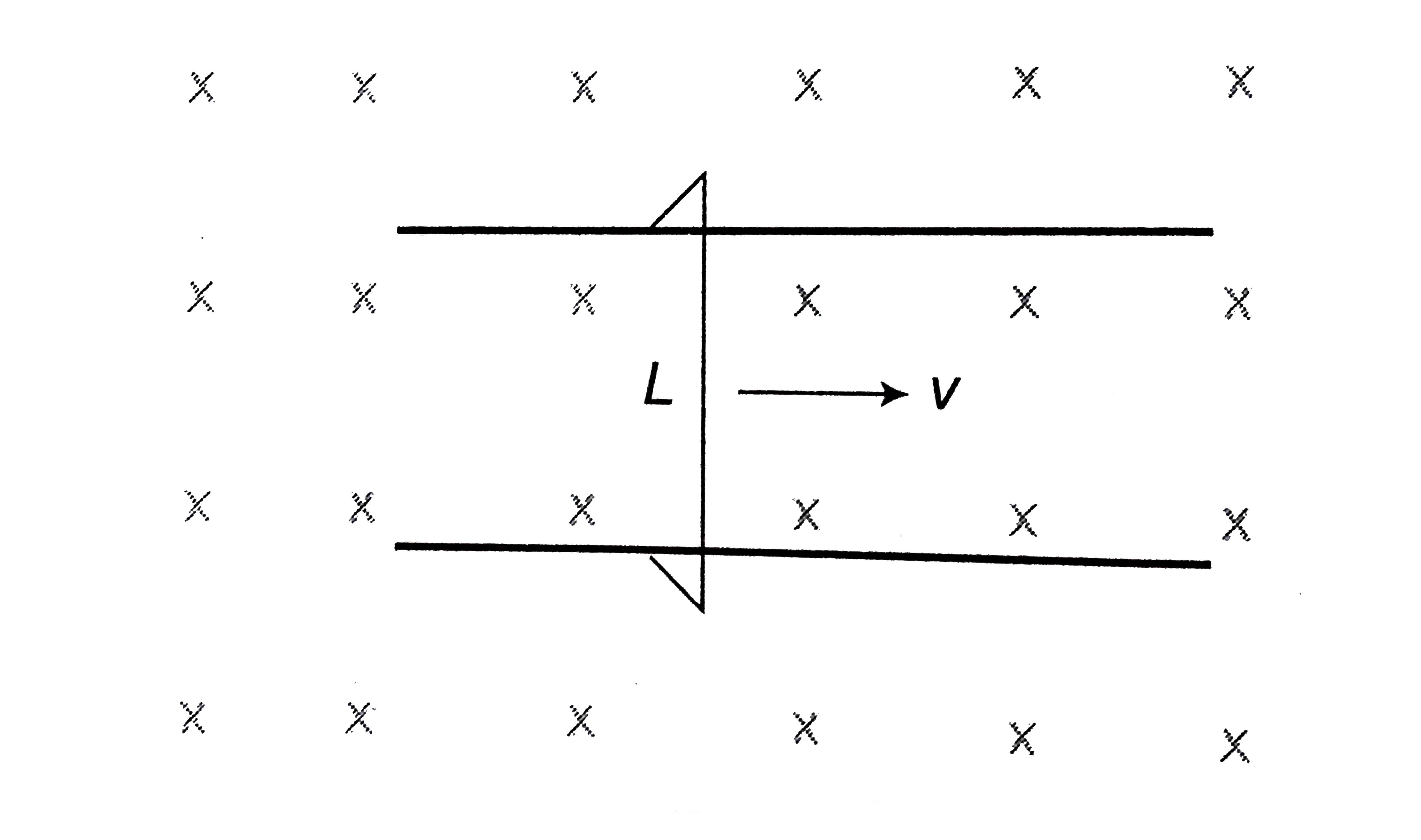
View SolutionIn a metal wire of mass m=24.1 mg can slide with negligible friction on two horizontal parallel rails separated by distance d=2.56 cm . The track lies in a vertical uniform magnetic field of magnitude 56.3 m. At time t=0 , device G is connected to the rails . producing a constant current i=9.13 mA in the wire and rails (even as the wire moves). At t=61.1 ms , what are the wire's (a) speed and (b) direction of motion (left or right)?
View SolutionA wire having mass m and length 1 can freely slide on a pair or parallel smooth horizontal rails placed in vertical magnetic field B. The rails are connected by a capacitor of capacitance C . The electric resistance of the rails and the wire is zero .If a constant force F acts on the Wire as shown in the figure . Then ,the acceleration of the wire can be given as
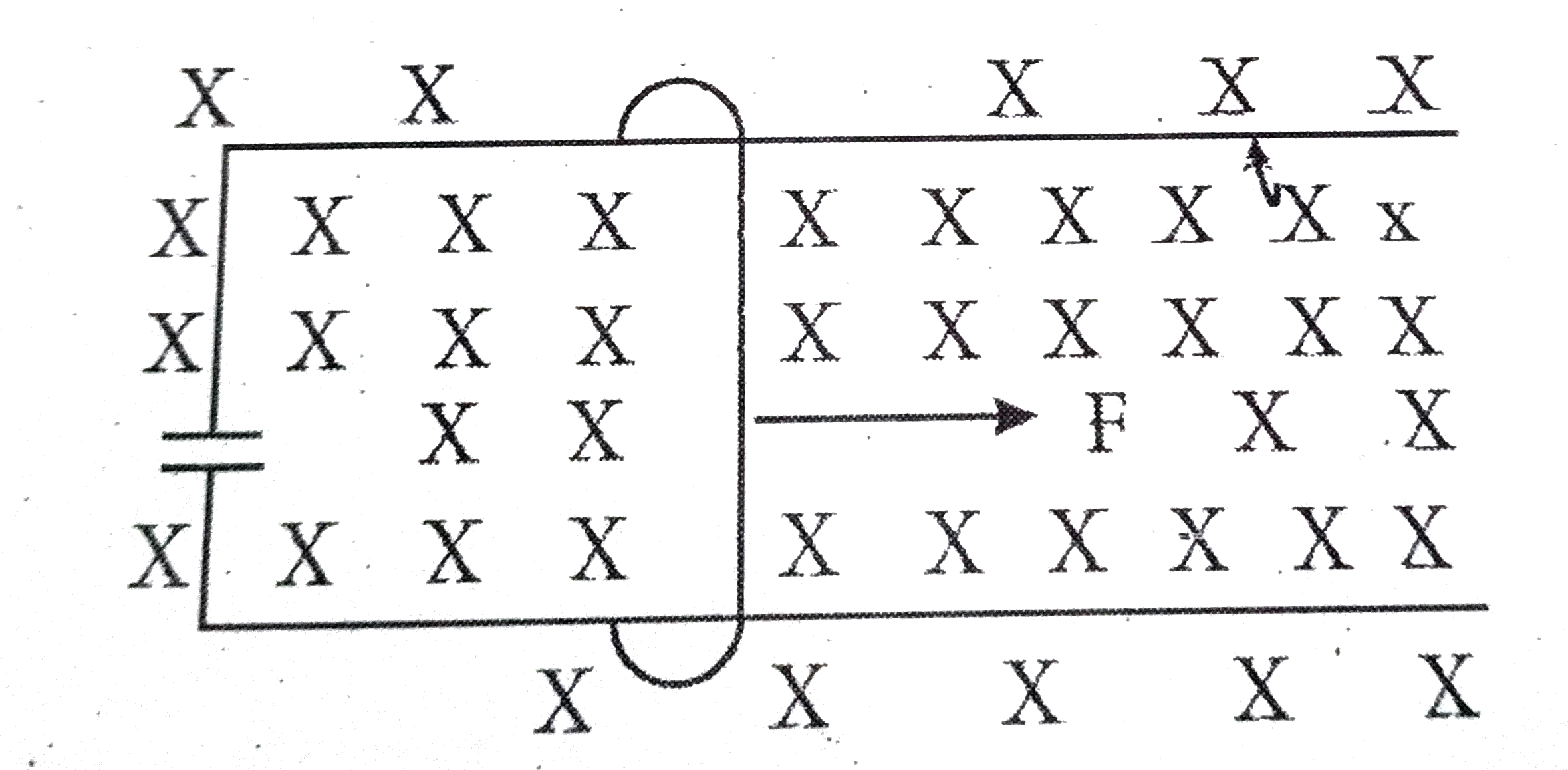
View SolutionShows two long metal rails placed horizontally and parallel to each other at a separation i. A uniform magnetic field b exists in the vertically downward direction. A wire of mass m can slide on the rails. The rails are connected to a constant current source which drives a current I in the circuirt. The friction coefficient between the rails and the wire is μ. (a) What should be the minimum value of μ which can prevent the wire from sliding on the rails? (b) Describe the motion of the wire if the value of μ is half the value found in the previous part.

View SolutionA straight wire of length l can slide on two parallel plastic rails kept in a horizontal plane with a separation d. The coefficient of friction between the wire and the rails is μ. If the wire carries a current I, what minimum magnetic field should exist in the space in order to slide the wire on the rails.
View SolutionA wire of mass m and length I can freely slide on a pair of parallel, smooth, horizontal rails placed in a vertical magnetic field B . The rails are connected by a capacitor of capacitance C. The electric resistance of the rails and the wire is zero. If a constant force F acts on the wire as shown in the figure, find the acceleration of the wire.
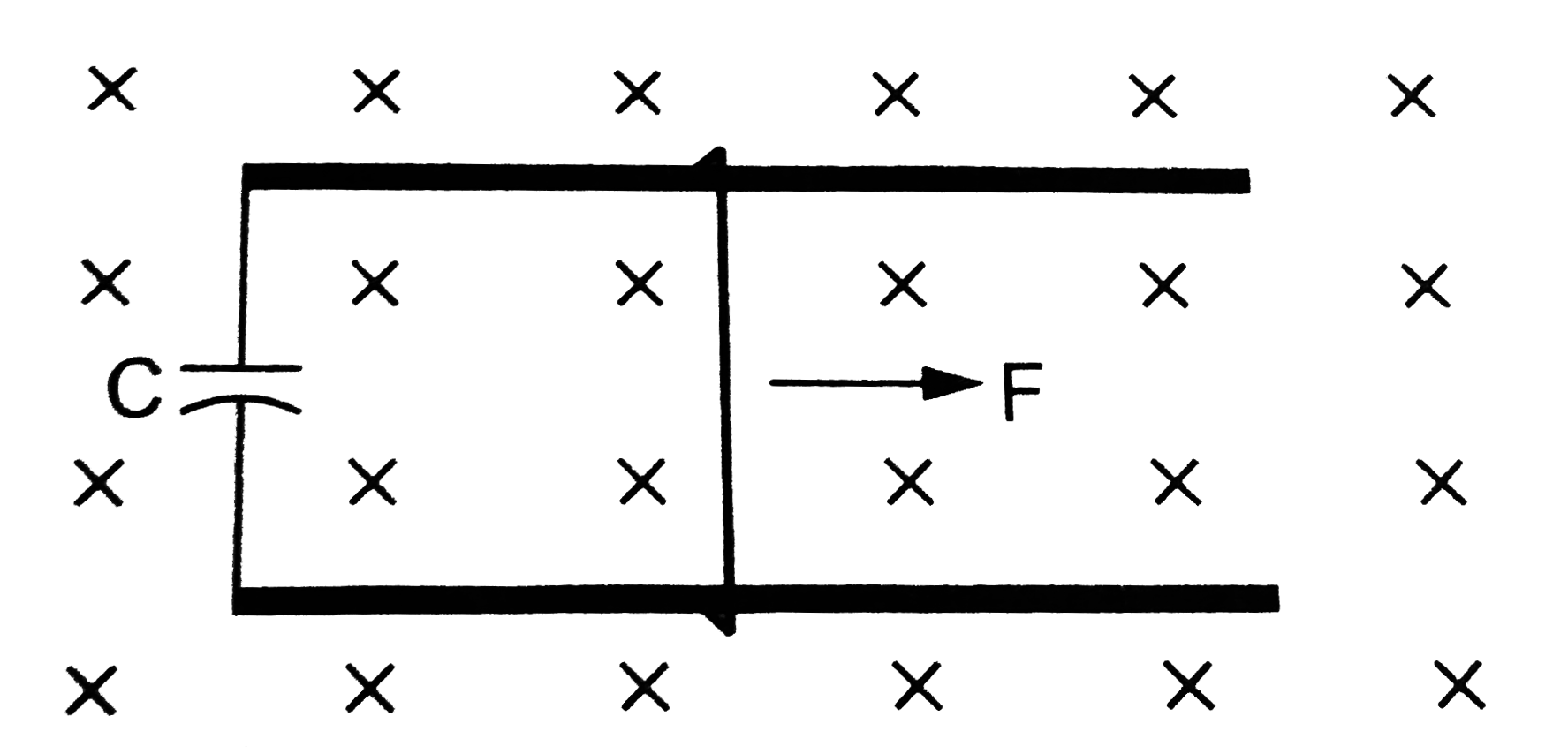
View SolutionFigure shows a wire sliding on two parallel, conducting rails placed at a separation L . A magnetic field B exists in a direction perpendicular to the plane of the rails. What force is necessary to keep the wire moving at a constant velocity V ?

View SolutionA metallic wire whose mass is m slips of friction on two rails placed at a distance l from each other. A uniform magnetic field perpendicular to the track B is formed. A fixed current in the wire flows along the rail and intercepts the other rail. The wire has an acceleration
View Solution