A block of ice starts sliding down from the top of the inclined roof of a house (angle of inclination of roof= $30^{\circ}$ with the horizontal) along aline of maximum slope. The highest and lowest points of the roof are at heights of 8.1m and 5.6 m respectively from the ground. At what horizontal distance from the starting point will the block hit the ground? Neglect friction.

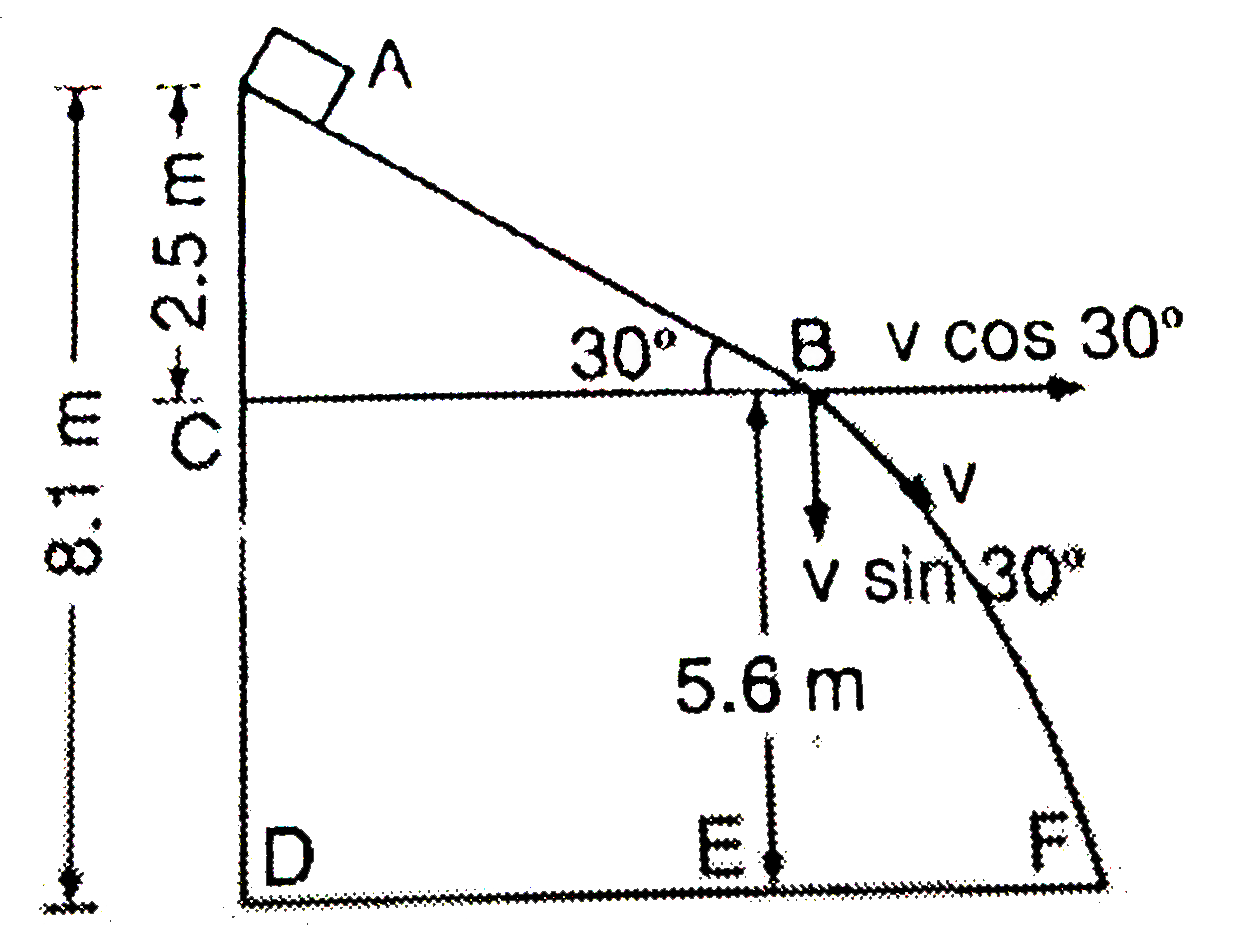
4.33m

14.33m

8.998m

0.668m

Video Solution

Text Solution

Verified by Experts

The ice block sliding on roof AB travels a vertical distance
AC $= (8.1 - 5.6) m = 2.5 m$
If v is the velocity of block at B then we have the relation
$v^{2} = u^{2} + 2 a s$
$u = 0 s = h_{1} = A C = 2.5 m$
and a $= g = 9.8 m / s$
$∴ V^{2} = 0 + 2 \times 9.8 \times 2.5$
$\Rightarrow v = 7 m / s a l o n g B G$
The horizontal and vertical (downwards) components of velocity are given by

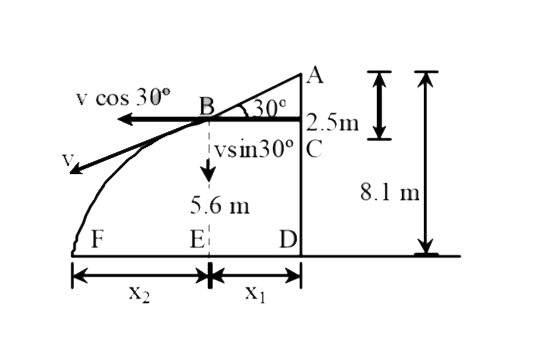
$v_{z} = v {cos 30}^{\circ} = 7 \times \frac{\sqrt{3}}{2} = 3.5 \sqrt{3} m / s$
$v - (z) v {sin 30}^{\circ} = 7 \times 1 / 2 = 3.5 m / s$
The time taken by the block to reach the ground is determined by vertical motion by the relation
$S = U T + (1 / 2) a t^{2}$
Here S $= h_{2} = 5.6, u = v_{y} = 3.5 m / s$
$a = g = 9.8 m / s$
$: > 5.6 = 3.5 t + (1 / 2) 9.8 t^{2}$
$\Rightarrow t = 0.77 s$
Horizontal distance
EF $= x_{2} = v_{20 t = 3.5 \sqrt{3} \times 0.77 = 4.668 m}$
Also $x_{1} = E D = B C = A C {cot 30}^{\circ} = 2.5 \sqrt{3} = 4.33$
$∴$ Total distance
$x = x_{1} + x_{2}$
$= 4.33 + 4.668$
$8.998 m$

Updated on:21/07/2023

A ball is thrown from a point in level with velocity $u$ and at a horizontal distance $r$ from the top of a tower of height $h$ .
At what horizontal distance $x$ from the foot of the tower does the ball hit the ground ?
A $\frac{u cos θ}{g} {{(u^{2} {sin}^{2} θ + g h)}^{1 / 2} - u sin θ}$
B $\frac{u cos θ}{g} {{(u^{2} {cos}^{2} θ + 2 g h)}^{1 / 2} - u cos θ}$
C $\frac{u cos θ}{g} {{(u^{2} {cos}^{2} θ + g h)}^{1 / 2} - u cos θ}$
D $\frac{u cos θ}{g} {{(u^{2} {cos}^{2} θ + g h)}^{1 / 2} - u cos θ}$
A boy playing on the roof of a $10 m$ high building throws a ball with a speed of $10 m / s$ at an angle of $30 (\circ)$ with the horizontal. How far from the throwing point will the ball be at the height of $10 m$ from the ground ?
$[g = 10 m / s^{2}, {sin 30}^{\circ} = \frac{1}{2}, {cos 30}^{\circ} = \frac{\sqrt{3}}{2}]$
A $5.2 m$
B $4.33 m$
C $2.66 m$
D $8.66 m$
A block is projected upwards with a velocity of 5 m/s from the bottom of a rough inclined plane of inclination 30° with the horizontal. It stops after 0.5 s. What is the coefficient of friction ? (g $= 10 m / s^{2}$ )
A $\sqrt{3}$
B $\frac{1}{\sqrt{3}}$
C $\sqrt{2}$
D $\sqrt{5}$