In the xy - plane, the point (0, 2) is the center of a circle that has radius 2. Which of the following is NOT a point on the circle?
(0,4)
(−2,4)
(−2,2)
(0,0)
The correct Answer is:B
To determine which point is NOT on the circle with center (0, 2) and radius 2, we will follow these steps:
Step 1: Write the equation of the circle
The standard equation of a circle with center (a, b) and radius r is given by:
(x−a)2+(y−b)2=r2
In this case, the center is (0, 2) and the radius is 2. Thus, we can substitute these values into the equation:
(x−0)2+(y−2)2=22
This simplifies to:
x2+(y−2)2=4
Step 2: Identify the points to test
Let's assume we have several points to test whether they lie on the circle or not. For example, let's consider the following points:
1. A: (-2, 2)
2. B: (-2, 4)
3. C: (2, 2)
4. D: (0, 0)
Step 3: Substitute each point into the equation
We will substitute each point into the equation x2+(y−2)2=4 to see if it satisfies the equation.
Testing Point A: (-2, 2)
(−2)2+(2−2)2=4+0=4
This point satisfies the equation.
Testing Point B: (-2, 4)
(−2)2+(4−2)2=4+22=4+4=8
This point does NOT satisfy the equation.
Testing Point C: (2, 2)
(2)2+(2−2)2=4+0=4
This point satisfies the equation.
Testing Point D: (0, 0)
(0)2+(0−2)2=0+4=4
This point satisfies the equation.
Conclusion
The point that does NOT lie on the circle is (-2, 4).
The standard equation of a circle with center (a, b) and radius r is given by:
Let's assume we have several points to test whether they lie on the circle or not. For example, let's consider the following points:
1. A: (-2, 2)
2. B: (-2, 4)
3. C: (2, 2)
4. D: (0, 0)
We will substitute each point into the equation x2+(y−2)2=4 to see if it satisfies the equation.
(−2)2+(2−2)2=4+0=4
This point satisfies the equation.
(−2)2+(4−2)2=4+22=4+4=8
This point does NOT satisfy the equation.
(2)2+(2−2)2=4+0=4
This point satisfies the equation.
(0)2+(0−2)2=0+4=4
This point satisfies the equation.
The point that does NOT lie on the circle is (-2, 4).
Similar Questions
The line 2x−y+1=0 is tangent to the circle at the point (2, 5) and the center of the circle lies on x−2y=4 . Then find the radius of the circle.
View SolutionThe line 2x−y+1=0 is tangent to the circle at the point (2, 5) and the centre of the circle lies on x−2y=4. The square of the radius of the circle is
View Solution
Knowledge Check
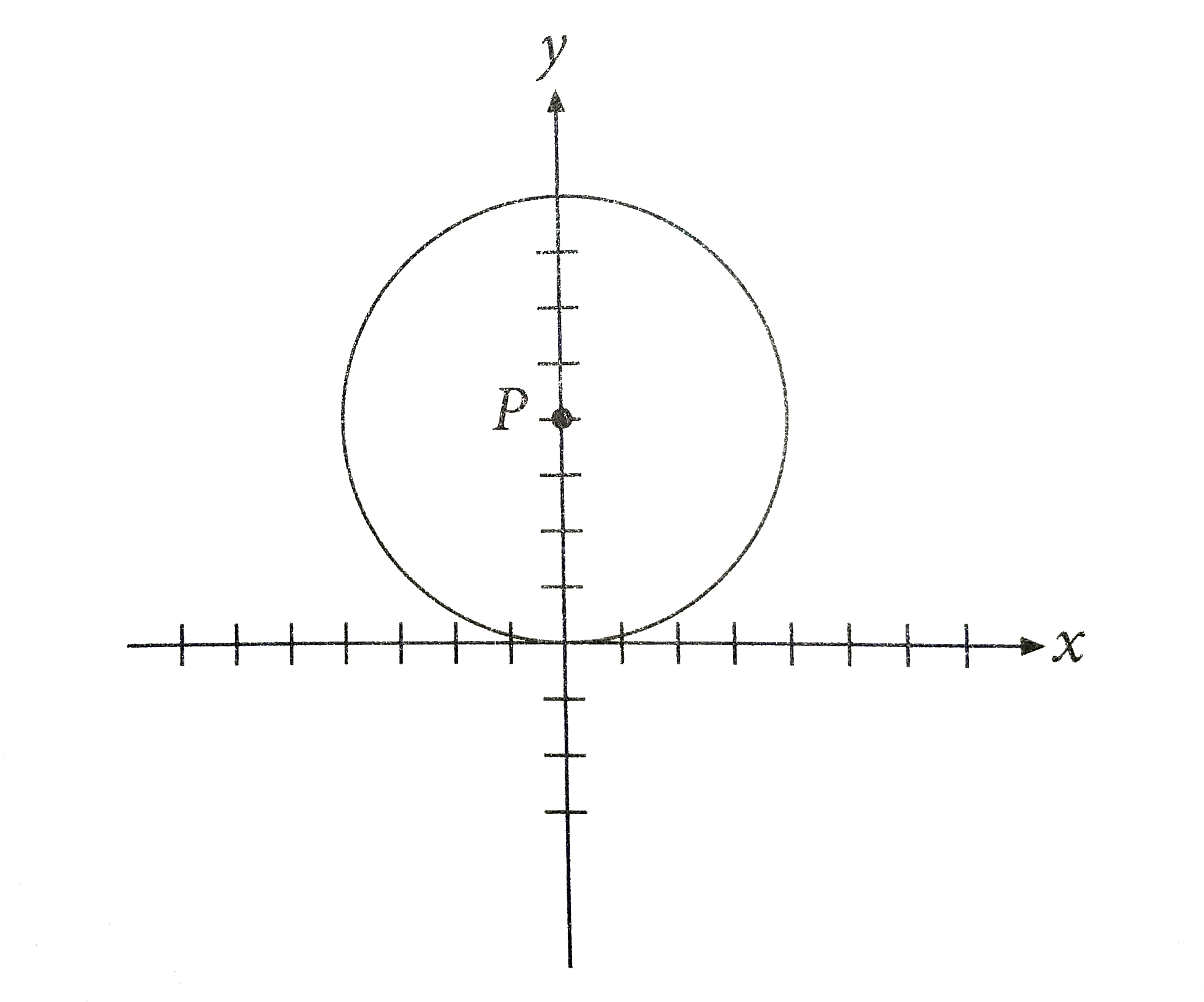
Point P is the certer of the circle shown above, which has a radius of 4. Which of the following points lies on circle P?A(4,0)B(0,4)C(−4,4)D(4,3)A circle center (3, 8) contains the point (2, -1). Which of the following is also a point on the circle ?
A(1, -10)B(4, 17)C(5, -9)D(7, 15)A circle in the xy -plane has center ( 5, 7) and radius 2. Which of the following is an equation of the circle?
A(x−5)2+(y−7)2=4B(x+5)2+(y+7)2=4C(x−5)2+(y−7)2=2D(x+5)2+(y+7)2=2
The center of circle O (not shown) falls on the point where the line y=43x+4 intersects the x-axis on the coordinate plane. The point (3, 8) lies on the circumference of the circle. Which of the following could be the equation for circle O?
View SolutionA circle in the xy-plane is tangent to the x-axis at -10 and the y-axis at 10. Which of the following is an equation of the circle ?
View SolutionIn the xy-plane, a circle with center O passes through the point (2, 0) and has a radius of 4. Which of the following could be the equation of circle O?
View SolutionIn the xy-plane, the graph of 2x2−6x+2y2=45 is a circle . What is the radius of the circle ?
View SolutionA circle in the xy‑plane has equation (x+3)2+(y−1)2=25. Which of the following points does NOT lie in the interior of the circle?
View Solution
