In the xy-plane, a circle with center O passes through the point (2, 0) and has a radius of 4. Which of the following could be the equation of circle O?
(x−2)2+(y+4)2=4
(x−2)2+(y+4)2=16
(x−4)2+(y+2)2=16
(x+2)2+(y−2)2=16
The correct Answer is:B
To find the equation of the circle with center O that passes through the point (2, 0) and has a radius of 4, we can follow these steps:
Step 1: Understand the standard form of a circle's equation
The standard form of a circle's equation is given by:
(x−h)2+(y−k)2=r2
where (h,k) is the center of the circle and r is the radius.
Step 2: Identify the center and radius
From the problem, we know:
- The radius r=4
- The circle passes through the point (2, 0).
However, we need to determine the center O. Since the center is not explicitly given, we can denote it as (h,k).
Step 3: Substitute the known values into the equation
Using the point (2, 0) which lies on the circle, we can substitute into the equation:
(2−h)2+(0−k)2=42
This simplifies to:
(2−h)2+k2=16
Step 4: Check the options provided
Now, we need to check which of the given options could represent this equation. We will evaluate each option by substituting the point (2, 0) into the equations.
Option A:
(x−2)2+(y+4)2=4
Substituting (2, 0):
(2−2)2+(0+4)2=4⟹0+16=4(False)
Option B:
(x−2)2+(y+4)2=16
Substituting (2, 0):
(2−2)2+(0+4)2=16⟹0+16=16(True)
Option C:
(x−4)2+(y+2)2=16
Substituting (2, 0):
(2−4)2+(0+2)2=16⟹4+4=8(False)
Option D:
(x+2)2+(y−2)2=16
Substituting (2, 0):
(2+2)2+(0−2)2=16⟹16+4=20(False)
Step 5: Conclusion
The only option that satisfies the condition is Option B:
(x−2)2+(y+4)2=16
Final Answer:
The equation of circle O is given by Option B.
---
The standard form of a circle's equation is given by:
(x−h)2+(y−k)2=r2
where (h,k) is the center of the circle and r is the radius.
From the problem, we know:
- The radius r=4
- The circle passes through the point (2, 0).
Using the point (2, 0) which lies on the circle, we can substitute into the equation:
(2−h)2+(0−k)2=42
This simplifies to:
(2−h)2+k2=16
Now, we need to check which of the given options could represent this equation. We will evaluate each option by substituting the point (2, 0) into the equations.
(x−2)2+(y+4)2=4
Substituting (2, 0):
(2−2)2+(0+4)2=4⟹0+16=4(False)
(x−2)2+(y+4)2=16
Substituting (2, 0):
(2−2)2+(0+4)2=16⟹0+16=16(True)
(x−4)2+(y+2)2=16
Substituting (2, 0):
(2−4)2+(0+2)2=16⟹4+4=8(False)
(x+2)2+(y−2)2=16
Substituting (2, 0):
(2+2)2+(0−2)2=16⟹16+4=20(False)
The only option that satisfies the condition is Option B:
(x−2)2+(y+4)2=16
The equation of circle O is given by Option B.
Topper's Solved these Questions
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #4
3 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #5
3 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #2
3 Videos
- ADVANCED ARITHMETICBOOK - PRINCETONCHAPTER - ADVANCED ARITHMETICEXERCISE - Examples
25 Videos
Similar Questions
Knowledge Check
The center of circle O (not shown) falls on the point where the line y=43x+4 intersects the x-axis on the coordinate plane. The point (3, 8) lies on the circumference of the circle. Which of the following could be the equation for circle O?
Ax2+y2=25B(x+3)2+y2=25C(x+3)2+y2=100D(x+3)2+(y−8)2=100In the xy-plane , a circle with center (6,0) is tangent to the line y=x. what is the radius of the circle ?
A2√6B2√3C3√2D36 In the xy-plane, the line determined by the points (2,k) and (32,k) passes through the origin. Which of the following could be the value of k ?
A0B4C8D16
Find the equation of the circle which passes through the points (0,0), (0,1) and (2,3)
View SolutionA circle in the xy -plane has center ( 5, 7) and radius 2. Which of the following is an equation of the circle?
View SolutionA circle in the xy-plane is tangent to the x-axis at -10 and the y-axis at 10. Which of the following is an equation of the circle ?
View SolutionA circle is tangent to the lines with the equations x = 5 and y = 7. Which of the following could be the coordinates of the center of the circle ?
View Solution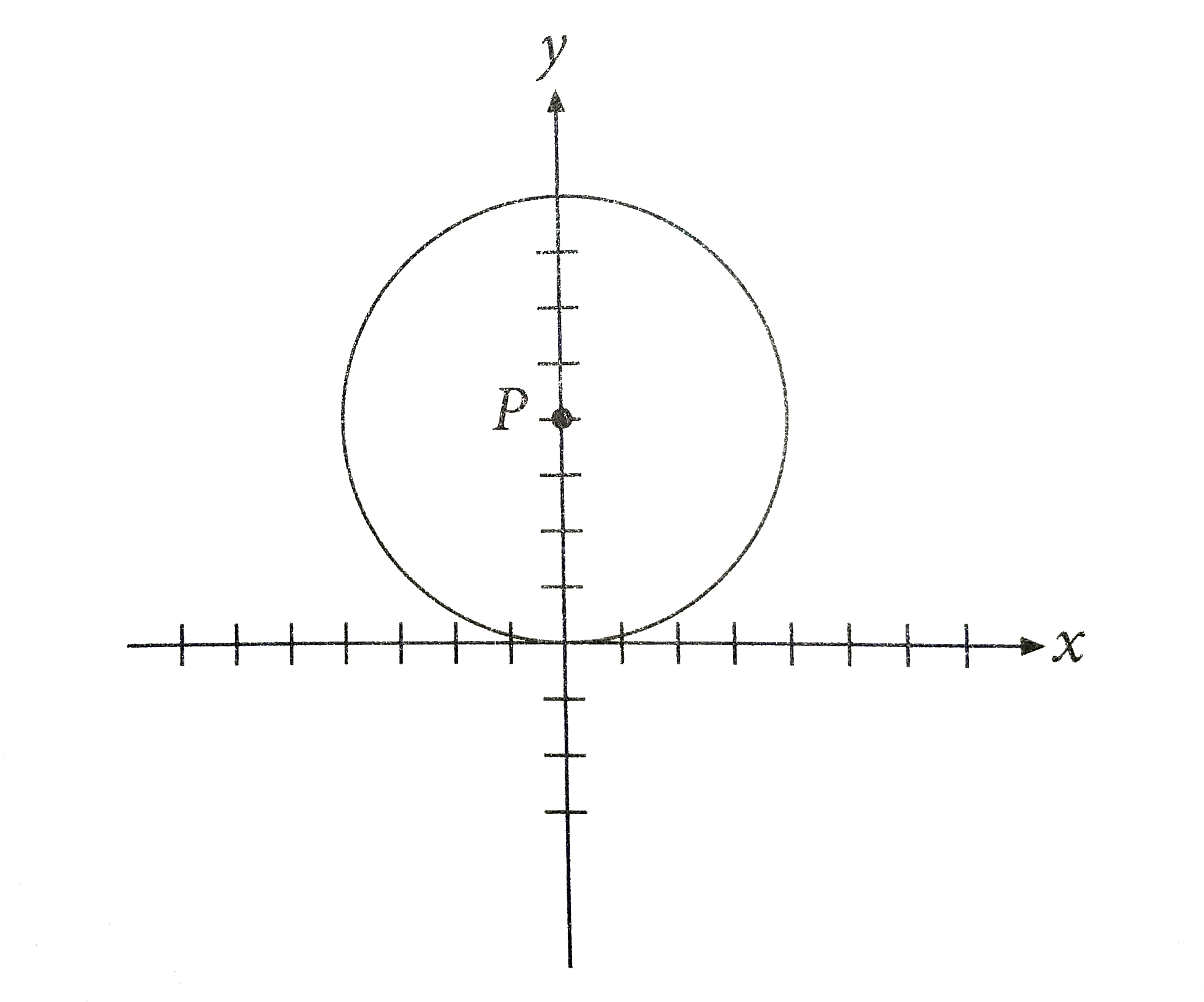
Point P is the certer of the circle shown above, which has a radius of 4. Which of the following points lies on circle P?
View Solution
