A particle describes a horizontal circle on the smooth inner surface of a conical funnel as shown in Fig. If the height of the plane of the circle above the vertex is 9.8cm, find the speed of the particle.
The correct Answer is:0.98m/s
The forces acting on the particle are reaction R and weight mg as shown in Fig. 7.119 . So , for verticle equilibrium of the particle in horizontal plane
R cos θ=mg ….(i)
And for circular motion of the particle in horizontal plane
R sin θ=(mv2/r).....(ii)
Dividing Eqn. (ii) by (i)
tan θ=v2rg
i.e., v=√rg tanθ
or v=√rg×(h/r)=√gh [ as from figure tan θ=h/r]
∴ v=√9.8×9.8×10−2=0.98m/s.

Topper's Solved these Questions
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - OBJECTIVE QUESTIONS ( only one choice is correct )
80 Videos
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - More than one correct
18 Videos
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - Comprehension type
11 Videos
- FORCE AND NEWTONS LAWS OF MOTIONBOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - Comprehension
12 Videos
- MOTION IN A STRAIGHT LINEBOOK - GRB PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Comprehension type Queston
14 Videos
Similar Questions
A particle describes a horizontal circule on the smooth inner surface of a coincal funnel as shown in Fig. If the height of the plane of the circle above the vertex is 9.8cm, find the speed of the particle.
View SolutionA particle describes a horizontal circle on the smooth inner surface of a conical funnel as shown in figure - 3.44. If the height of the plane of the circle above the vertex is 9.8 cm, find the speed of the particle.
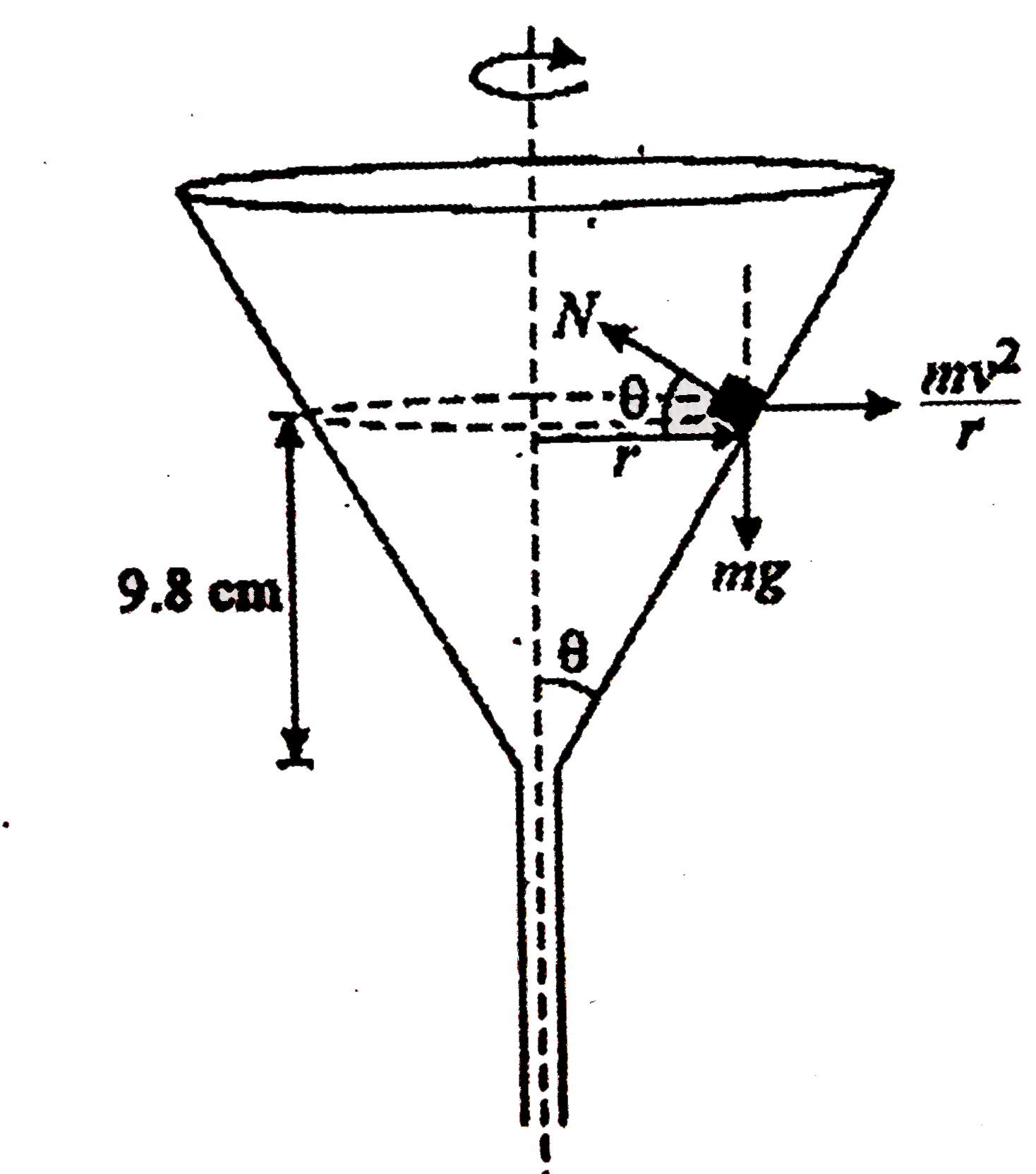
View Solution
Knowledge Check
A small ball descibes a horozontal circle on the smooth inner surface of a conical funnel. If the height of the plane of the circle above the vertex be 10 cm what is the speed of the particle?
A2 m/sB4 m/sC16 m/sD1 m/sA small ball describes a horizontal circle on the smooth inner surface of a conical funnel. If the height of the plane of the circle above vertex be 10 cm, what is the speed of the particle ?
A2 m/sB4 m/sC16 m/sD1 m/sA small ball describes a horizontal circle on the smooth inner surface of a conical funnel. If the height of the plane of the circle above vertex be 10 cm, what is the speed of the particle ?
A2 m/sB4 m/sC16 m/sD1 m/s
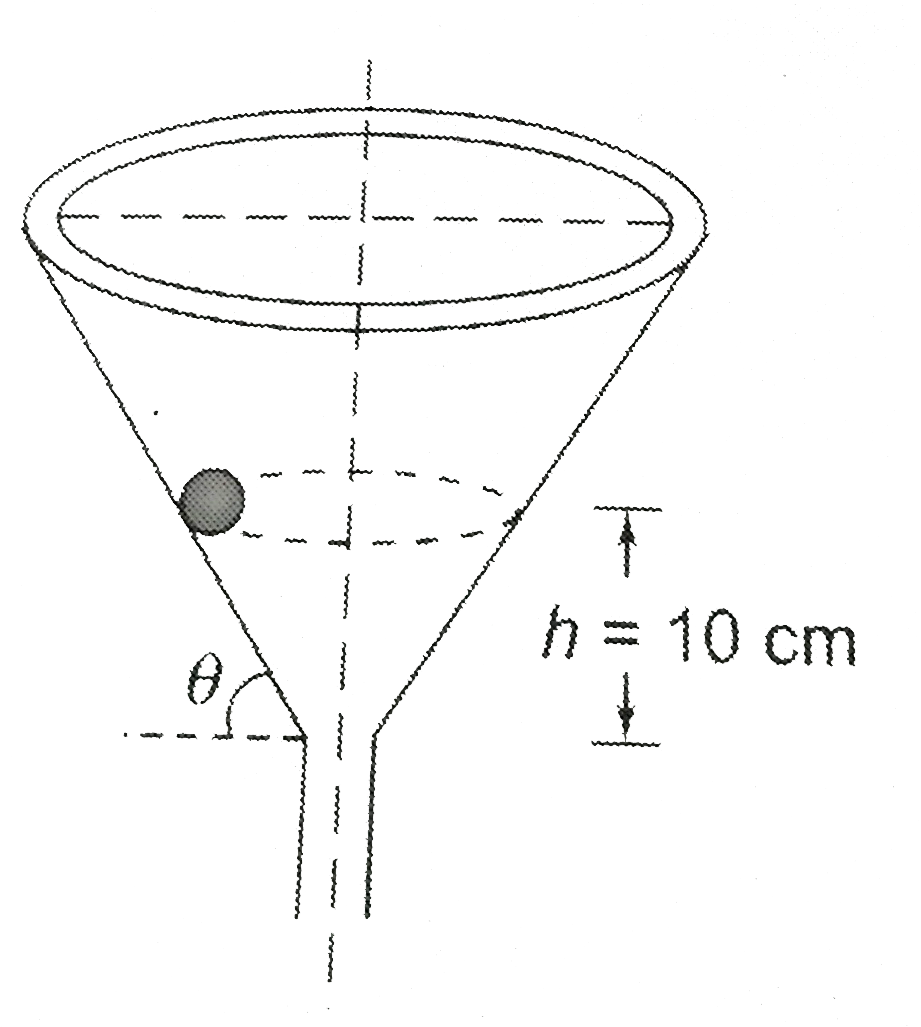
A particle describes a horizontal circle on smooth inner surface of a conical funnel as shown. If the height of the plane of the circle. Above the vertax is 10cm, find the speed of the paricle.
View SolutionA particle describes a horizontal circle on the smooth surface of an inverted cone. The height of the plane of the circle aove the vertex is 9.8 cm. Find the speed of the particle. Take g=9.8ms−2.
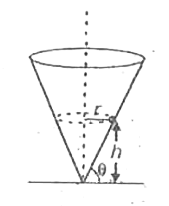
View SolutionA particle describes a horizontal circle of radius r on the smooth surface of an inverted cone as shown. The height of plane of circle above vertex is h. The speed of particle should be
View SolutionA particle describes a horizontal circle in a conical funnel whose inner surface is smooth with speed of 0.5m/s . What is the height of the plane of circle from vertex the funnel?
View SolutionA particle is moving in a horizontal circle with constant speed. It has constant
View Solution