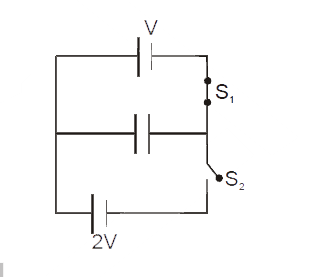
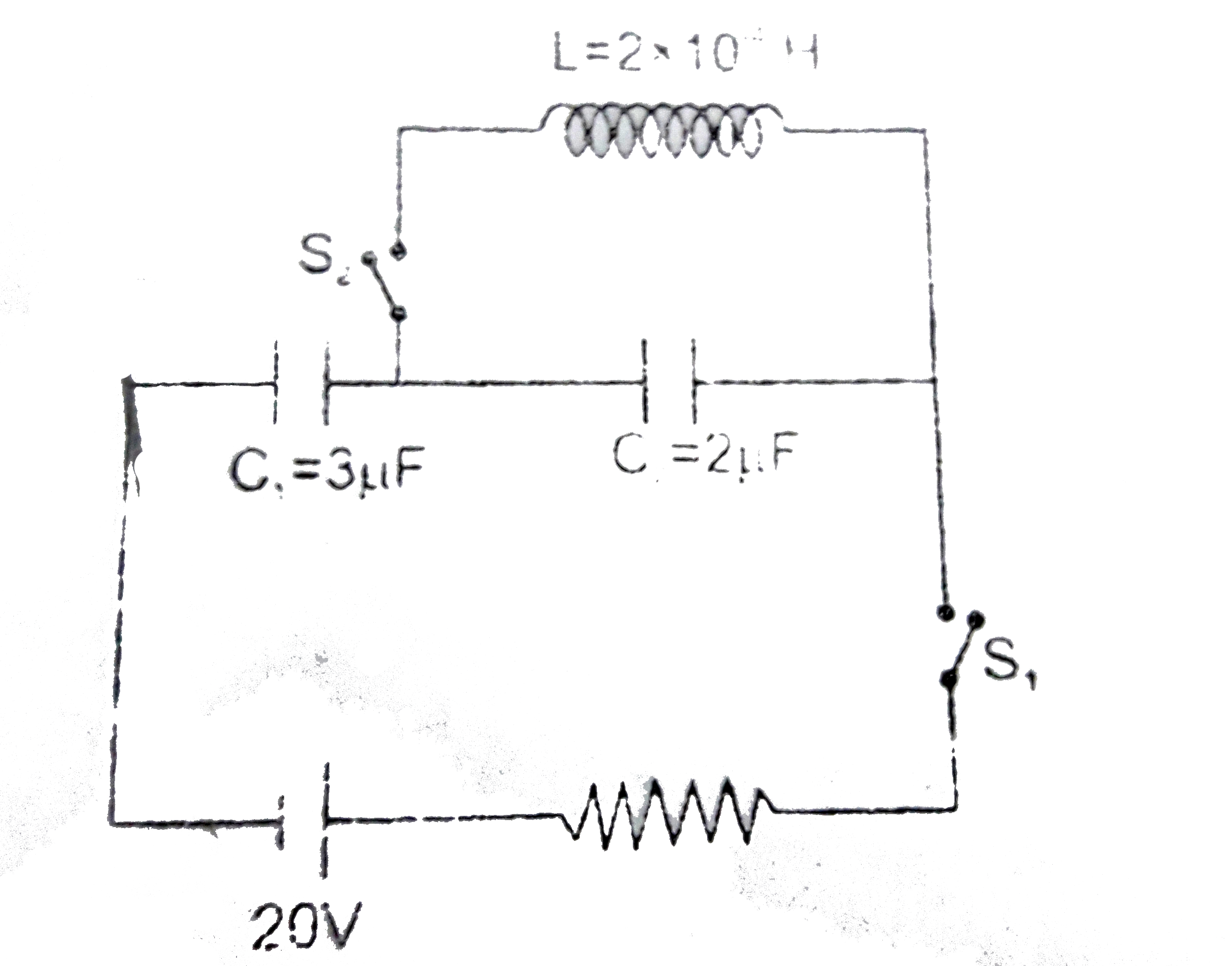
At any time S1 switch is opened and S2 is closed then find out heat generated in circuit.
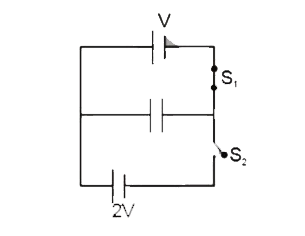
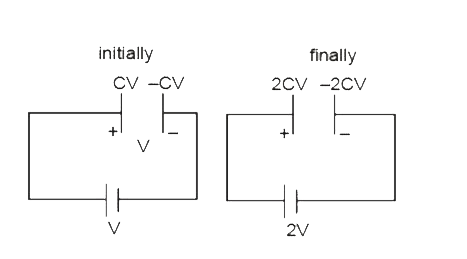
Charge flow through battery =Qt−Qi
=2CV−CV=CV
H = (CV x 2V) −{12C(2V)2−12CV2}
=2CV2−{2CV2−12CV2}
H= 12CV2
Topper's Solved these Questions
- Capacitance BOOK - MOTIONCHAPTER - Capacitance EXERCISE - EXERCISE -1
60 Videos
- Capacitance BOOK - MOTIONCHAPTER - Capacitance EXERCISE - EXERCISE -2
36 Videos
- CALORIMETRY BOOK - MOTIONCHAPTER - CALORIMETRY EXERCISE - EXERCISE - 3 Section - B
4 Videos
- CENTRE OF MASSBOOK - MOTIONCHAPTER - CENTRE OF MASSEXERCISE - Exercise - 4 Level-II
20 Videos
Similar Questions
Knowledge Check
The capacitor of capacitance C can be charged (with the help of a resistance R) by a voltage source V, by closing switch S1 while keeping switch S2 open. The capacitor can be connected in series with an inductor ‘L’ by closing switch S2 and opening S1
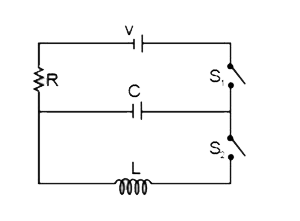
After the capacitor gets fully charged, S1 is opened and S2 is closed so that the inductor is connected in series with the capacitor. Then,Aat t = 0, energy stored in the circuit is purely in the form of magnetic energyBat any time t > 0, current in the circuit is in the same direction.Cat t > 0, there is no exchange of energy between the inductor and capacitorDat any time t > 0, instantaneous current in the circuit may V√CL
At t =0 switch S1 is closed and remains closed for a long time and S2 remains open. Now S1 is opened and S2 is closed. Find out
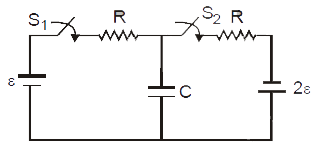
Charge on the capacitor long after that moment.
View SolutionIn given circuit initially switch Sw1 is closed and Sw2 is open, Now Sw1 is opened and Sw2 is closed, what is the heat liberated in resistance R?

View SolutionAt t =0 switch S1 is closed and remains closed for a long time and S2 remains open. Now S1 is opened and S2 is closed. Find out
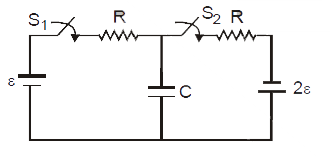
The current through the capacitor immediately after that moment
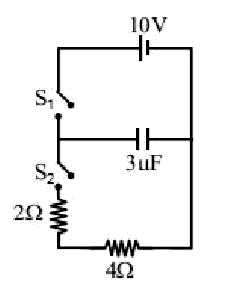
View SolutionIn given circuit initially switch S1 is closed for long time and then opened and now S2 is closed. The total energy dissipated in 4Ω resistor is equal to :-
View SolutionThe circuit shown in figure is in the steady state with switch S1 closed.At t=0,S1 is opened and switch S2 is closed. The first instant t when energy in inductor becomes one third of that in capacitor is equal to π×10−5xsec. Then finout value of x.
View SolutionStatement I : Switch is used to close are open electric circuit .
Statement II : When switch is closed, then circuit is closed and when it is opened, then circuit is open circuit
View SolutionThe circuit shown in figure is in the steady state with switch S1 closed. At t=0, S1 is opened and switch S2 is closed.
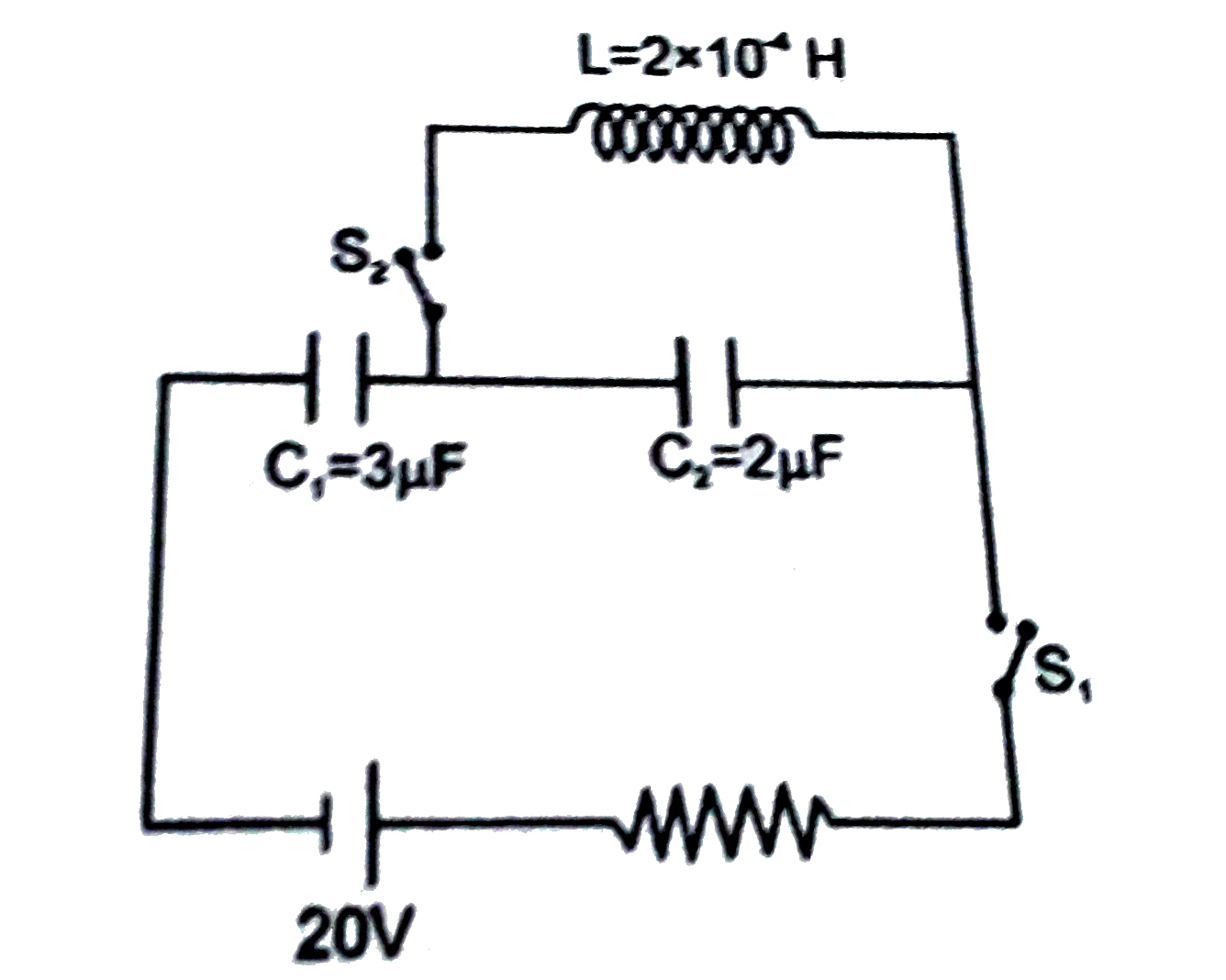
Find the first instant t, when energy in inductor becomes one third of that in capacitor
View Solution