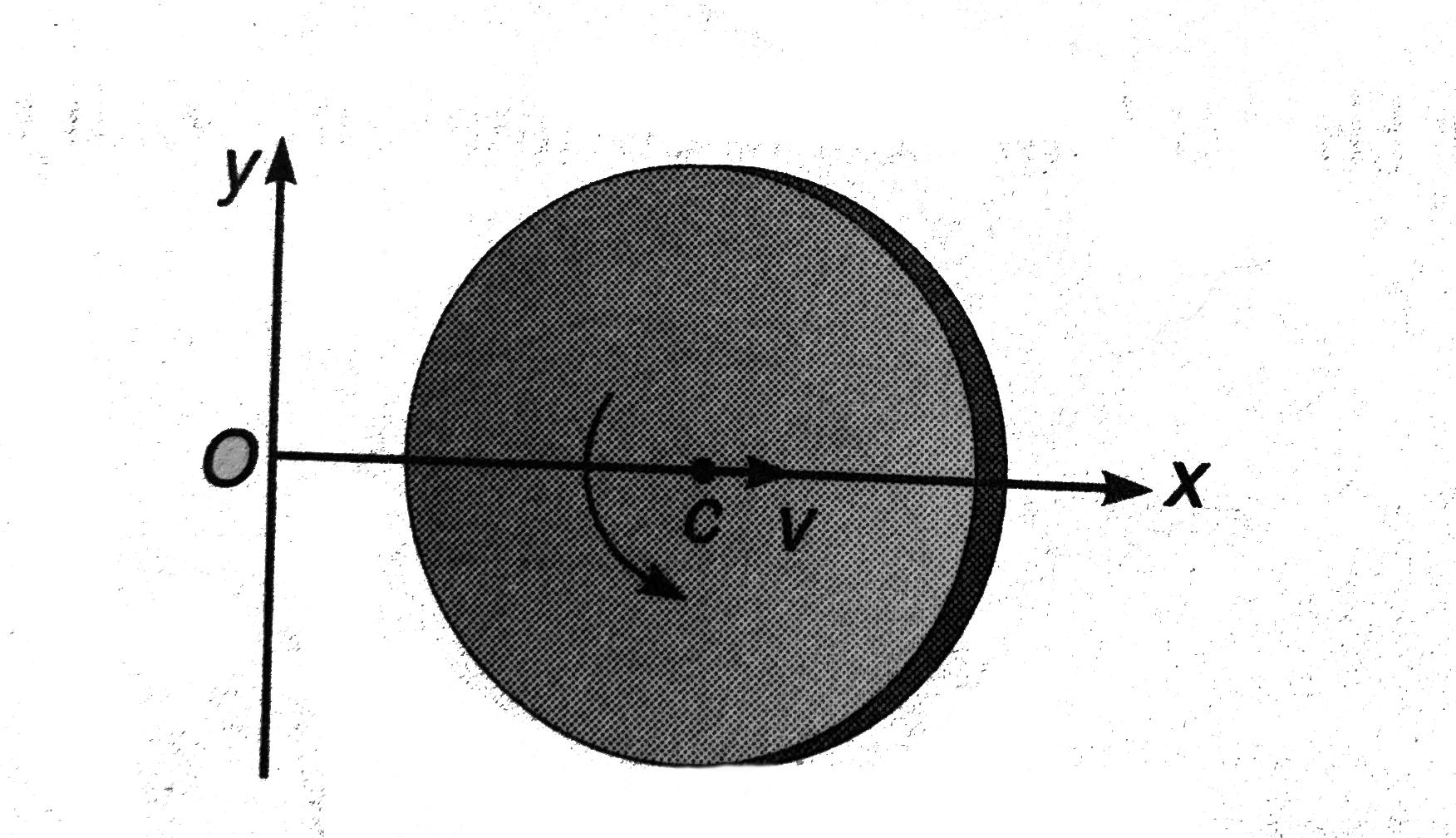
A rotating disc moves in the positive direction of the x-axis. Find the equation y(x) describing the position of the instantaneous axis of rotation if at the initial moment of the centre c of the disc was located at the point O after which it moved with constant velocity v while the disc started rotating counterclockwise with a constant angular acceleration α. the initial angular velocity is equal to zero.
t=xvandω=αt=αxv
The position of IAOR will be at a distance
y=vωory=vαxv
or y=v2αxorxy=v2α = constant
This is the desired x - y equation. This equation represents a rectangular hyperbola.
Topper's Solved these Questions
Similar Questions
A rotating disc moves in the positive direction of x-axis as shown. Find the equation y(x) describing the position of the instantaneous axis of rotation if at the initial moment the centre C of the disc was located at origin after which
a. it moved with constant acceleration a (initial velocity zero) while the disc rotating anticlockwise with constant angular velocity ω. b. it moved with constant velocity v while the disc started rotating anticlockwise with a constant angular acceleration a (with initial angular velocity zero).
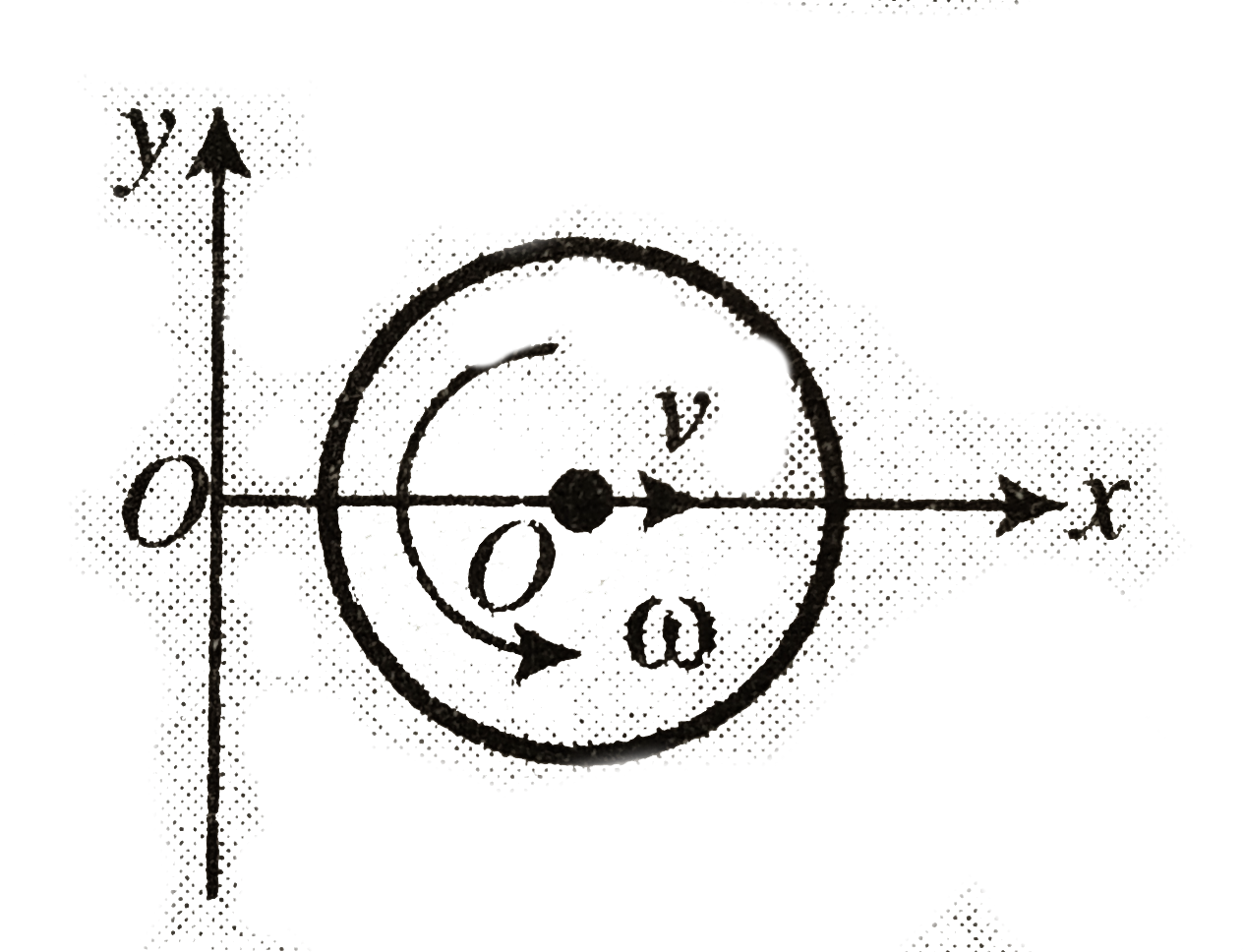
View SolutionFind the position of instantaneous centre of rotation and angular velocity of the disc in the following cases as shown. Radius of disc is R in each case.

View Solution
Knowledge Check
If angular velocity of a disc depends an angle rotated θ as ω=θ2+2θ, then its angular acceleration α at θ=1 rad is :
A8rad/s2B10rad/s2C12rad/s2DNone of theseTwo particles P and Q are located at distance rpandrQ respectively form the centre of rotating disc such that rP>rQ. The disc is rotating with constant angular acceleration. We can say
Aboth P and Q have the same accelerationBboth P and Q do not have the same accelerationCP has greater acceleration than QDQ has greater acceleration than PAn ant is sitting at the edge of a rotating disc. If the ant reaches the other end, after moving along the diameter, the angular velocity of the disc will:-
Aremain constantBfirst decreases and then increasesCfirst increases, then decreaseDIincrease continuously
A disc, initially at rest, starts rotating about its own axis/ with a constant angular acceleration of 0*2 rad/s2. The time taken by the disc to rotate by 10 rad (in seconds) is
View Solution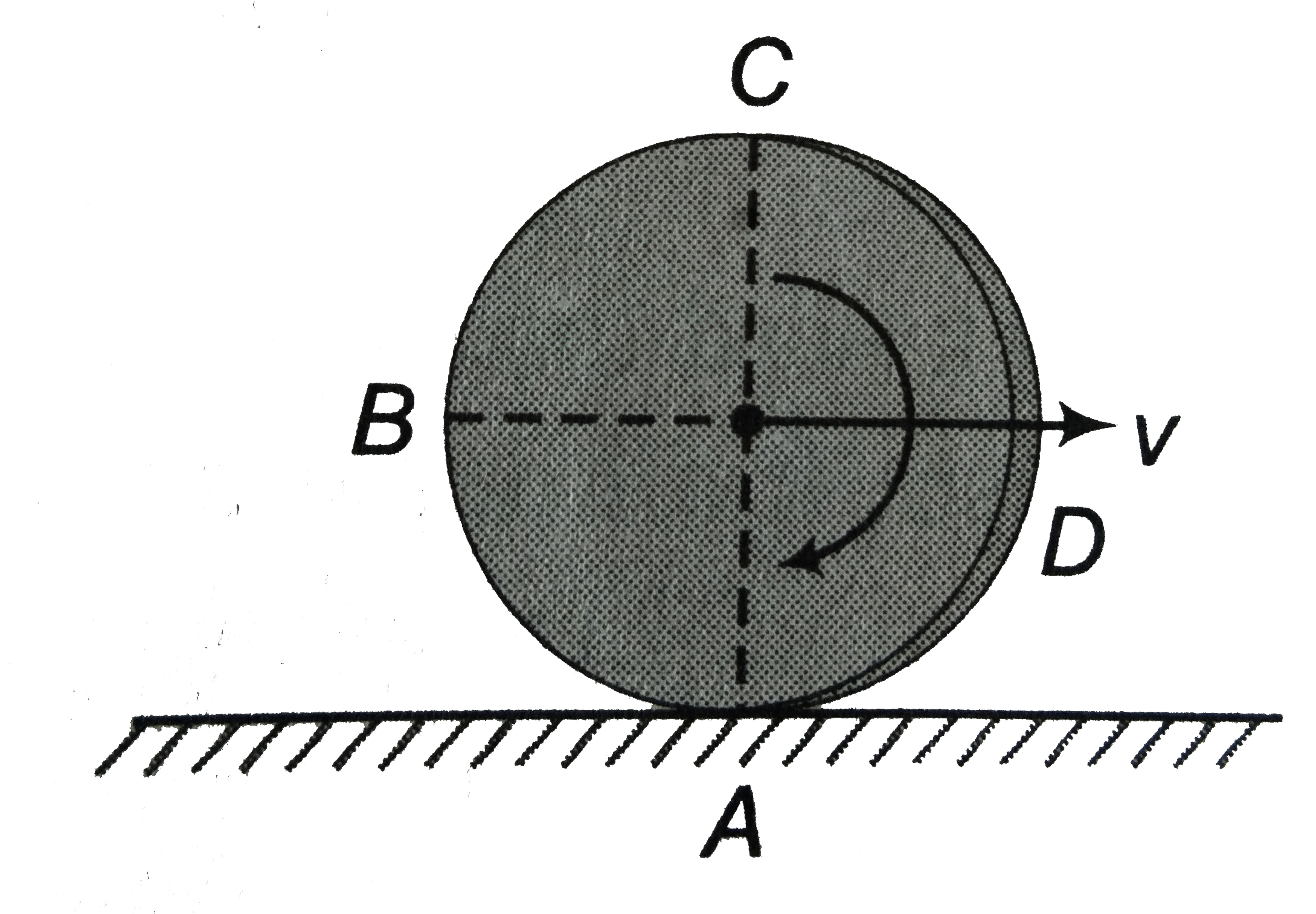
A disc is rolling without slipping with linear velocity v as shown in figure. With the concept of instantaneous axis of rotation, find velocities of point A, B, C and D.
View SolutionA disc rotates with constant angular velocity. Doesa point on its rim have a tangential acceleration or a radial acceleration ? Are these accelerations constant ? '
View SolutionA circular disc is rotating about its own axis at uniform rate completes 30 rotations in one minute.The angular velocity of disc in rad s−1 is
View SolutionA non-conducting disc having unifrom positive charge Q, is rotating about its axis with unifrom angular velocity ω.The magnetic field at the centre of the disc is.

View Solution
