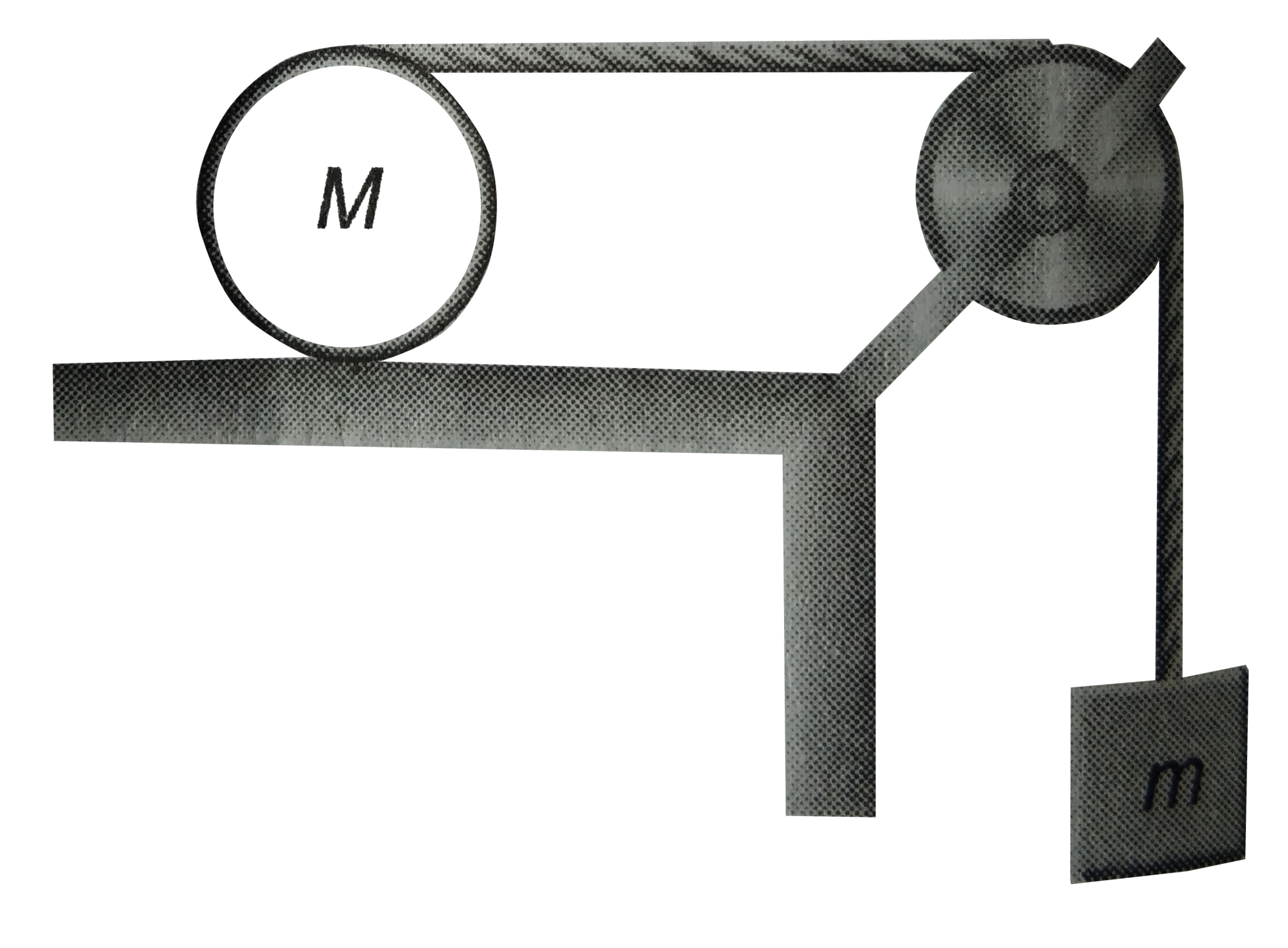
Consider the arrangement shown in figure. The string is wrapped around a uniform cylinder which rolls without slipping. The other end of the string is passed over a masslessm frictionless pulley to a falling weight, determine the acceleration of the falling mass m in terms of only the mass of the cylinder M, the mass m and g
Let T be the tension the string and f the force of (static) friction, between the cylinder and the surface a1= acceleration of centre of mas of cylinder towards right
a2= downward acceleration of block m
α= angular acceleration of cylinder (clockwise)
Equations of motion are :
For block mg - T =ma2 ...(i)
For cylinder, T + f =ma1 ...(ii)
α=(T−f)R12MR2 ...(iii)
The string attaches the mass m to the highest point of the cylinder, hence
vm=vCOM+Rω
Differentiating, we get
a2=a1+Rα ...(iv)
We also have (for rolling without slipping)
a1=Rα ...(v)
Solving these equations, we get a2=8mg3M+8m
Topper's Solved these Questions
Similar Questions
Four identical spheres having mass M and radius R are fixed tightly within a massless ring such that the centres of all spheres lie in the plane of ring. The ring is kept on a rough horizontal table as shown. The string is wrapped around the ring can roll without slipping. The other end of the string is passed over a massless frictionless pulley to a block of mass M. A force F is applied horizontally on the ring, at the same level as the centre, so that the system is in equilibrium.
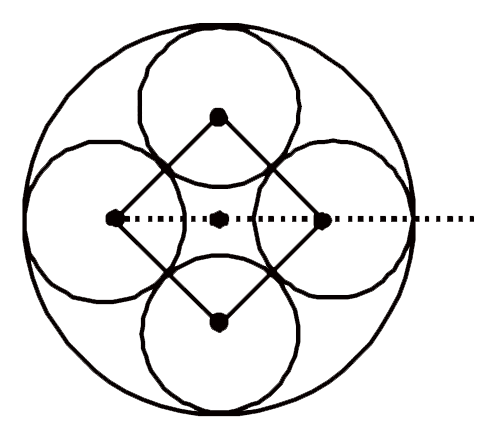
The magnitude of F is
View SolutionFour identical spheres having mass M and radius R are fixed tightly within a massless ring such that the centres of all spheres lie in the plane of ring. The ring is kept on a rough horizontal table as shown. The string is wrapped around the ring can roll without slipping. The other end of the string is passed over a massless frictionless pulley to a block of mass M. A force F is applied horizontally on the ring, at the same level as the centre, so that the system is in equilibrium.
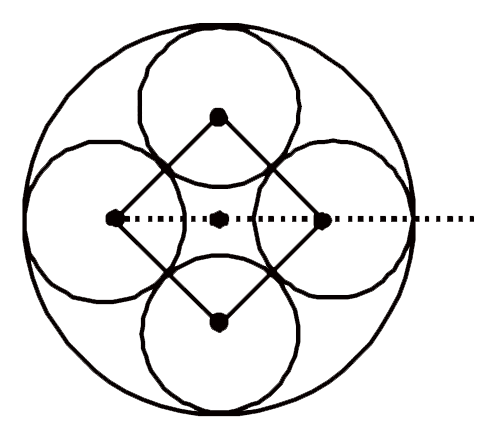
The moment of inertia of the combined ring system about the centre of ring will be
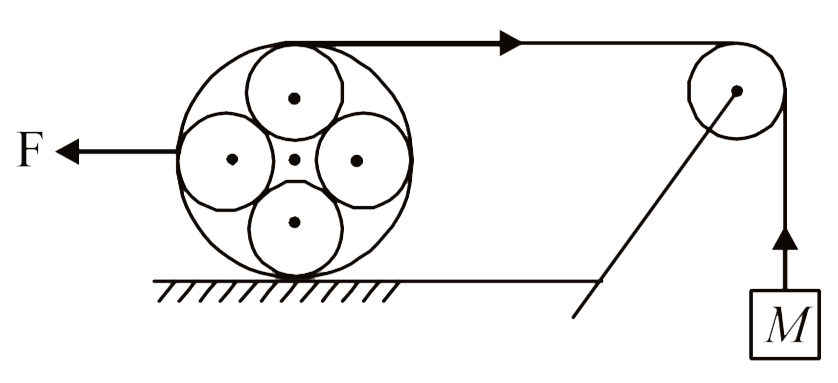
View Solution
Knowledge Check
Two particles of masses m and M(M>m) are connected by a cord that passes over a massless, frictionless pulley. The tension T in the string and the acceleration a of the particles is
AT=2mM(M−m)g,aMm(M+m)gBT=2mM(M+m)g,a=(M−mM+m)gCT=(M−mM+m)g,a=(2mM(M+m))gDT=(Mm(M+m)g,a=(2mM(M+m))gTwo spheres of masses 3 kg and 4 kg are attached to ends of a string which passes over a frictionless pulley. Take g=9.8m/2. The relative acceleration of the system is
A4ms−2B1.4ms−2C3ms−2D2.8ms−2Two masses m and m (m>m) are connected by massless flexible and inextensible string passed over massless and frictionless pulley. The acceleration of centre of mass is
A(m1−m2m1+m2)2gBm1−m2m1+m2gCm1+m2m1−m2gDZero
Two masses M and m are connected at the two ends of an inextensible string. The string passes over a smooth frictionless pulley. Calculate the acceleration of the masses and the tension in the string. Given M>m.
View SolutionA string wrapped around a cyliner of mass m and radius R. The end of the string is connected to block of same mass hanging vertically. No friction exists between the horizontal surface and cylinder.
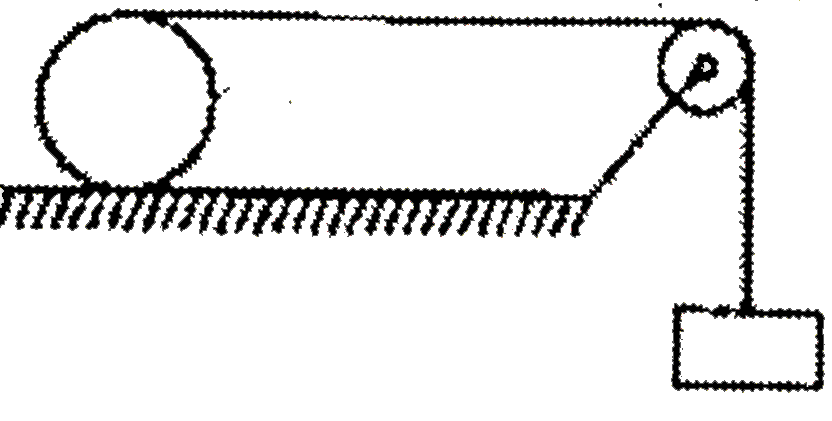
Acceleration of hanging mass is:
View SolutionA string of negligible mass is wrapped on a cylindrical pulley of mass M and radius R. The other end of string is tied to a bucket of mass m. If the pulley rotates about a horizontal axis then the tension in the string is :
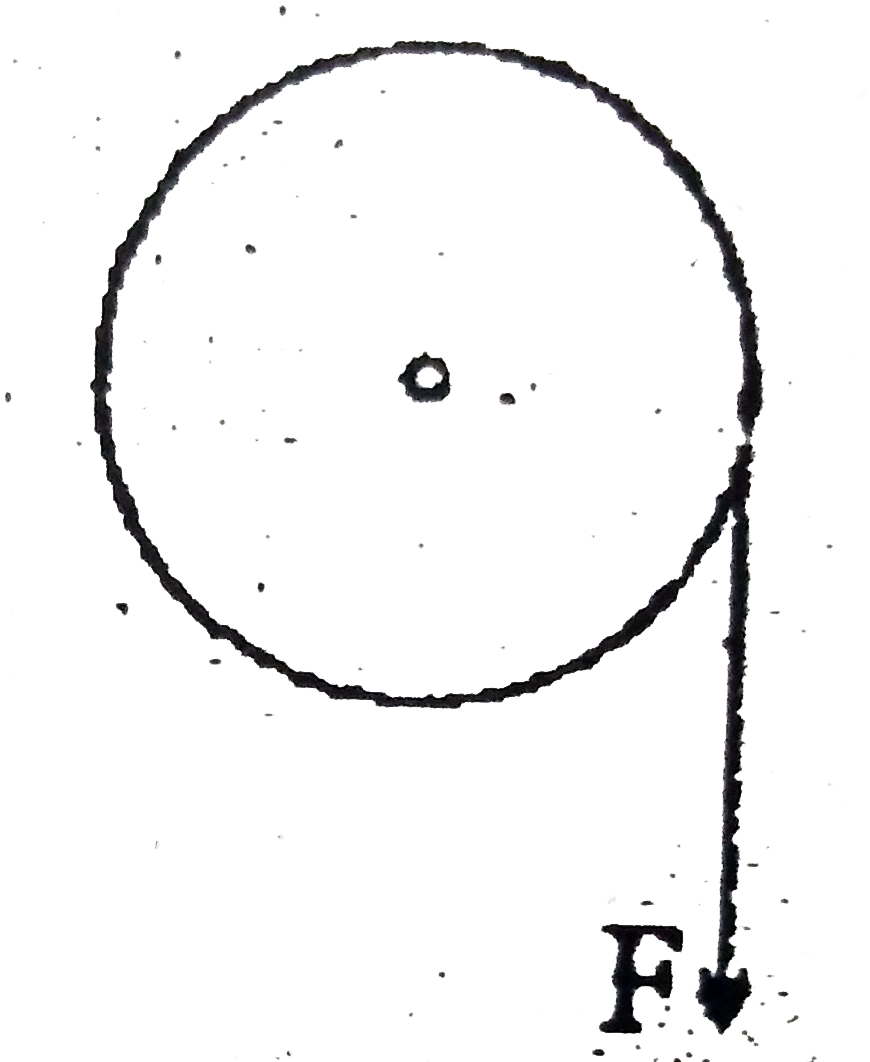
View SolutionA uniform cylinder free to turn around a fixed axis has a string wrapped around it as shown. The string is pulled with a force F equal to the cylinders weight. What is the acceleration of the string?
View SolutionA string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
View Solution