An thin rod of negligible mass and area of cross - section 4×10−6m2, suspended vertically from one end, has a length of 0.5 m at 100∘C. The rod is cooled to 0∘C but prevented from contracting by attaching a mass at the lower end. The value of this mass is ( in Kg )
(Given, coefficient of linear expansion is 10−5∘C−1, Young's modulus is Y=1011Nm−2andg=10ms−2)
The correct Answer is:40
Step by step video, text & image solution for An thin rod of negligible mass and area of cross - section 4xx10^(-6)m^(2), suspended vertically from one end, has a length of 0.5 m at 100^(@)C. The rod is cooled to 0^(@)C but prevented from contracting by attaching a mass at the lower end. The value of this mass is ( in Kg ) (Given, coefficient of linear expansion is 10^(-5^(@))C^(-1), Young's modulus is Y=10^(11)Nm^(-2) and g=10ms^(-2)) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
An thin rod of negligible mass and area of cross - section 4×10−6m2, suspended vertically from one end, has a length of 0.5 m at 100∘C. The rod is cooled to 0∘C but prevented from contracting by attaching a mass at the lower end. The value of this mass is ( in Kg )
(Given, coefficient of linear expansion is 10−5∘C−1, Young's modulus is Y=1011Nm−2andg=10ms−2)
View SolutionAn thin rod of negligible mass and area of cross - section 4×10−6m2, suspended vertically from one end, has a length of 0.5 m at 100∘C. The rod is cooled to 0∘C but prevented from contracting by attaching a mass at the lower end. The value of this mass is ( in Kg )
(Given, coefficient of linear expansion is 10−5∘C−1, Young's modulus is Y=1011Nm−2andg=10ms−2)
View Solution
Knowledge Check
A thin rod of negligible mass and a cross-section of 2×10−6m2 suspended vertically from one end, has a length of 0.5m at 200∘C. The rod is cooled at 0∘C, but prevented from contracting by attaching a mass at the lower end. The value of this mass is : (Young's modulus =1011N/m2, Coefficient of linear expansion 10−5K−1andg=10m/s2):
A20kgB30kgC40kgD50kgA thin rod of negligible mass and area of cross section S is suspended vertically from one end. Length of the rod is L0 at T∘C . A mass m is attached to the lower end of the rod so that when temperature of the rod is reduced to 0∘C its length remains L0 Y is the Young’s modulus of the rod and α is coefficient of linear expansion of rod. Value of m is :
ASYαTgBSYαT2gCSYα2gTDSYαT2gL0A block is hanged by means of two identical wires having cross section area 1mm2 as shown in the diagram. If temperature is lowered by 10∘C, find the mass (in kg) to be added to the hanging mass such that the junction (J) remains at initial position, Given that coefficient of linear expansion α=2×10−5/.∘C, Young's modolus Y=5×1011N/m2 for the wire.
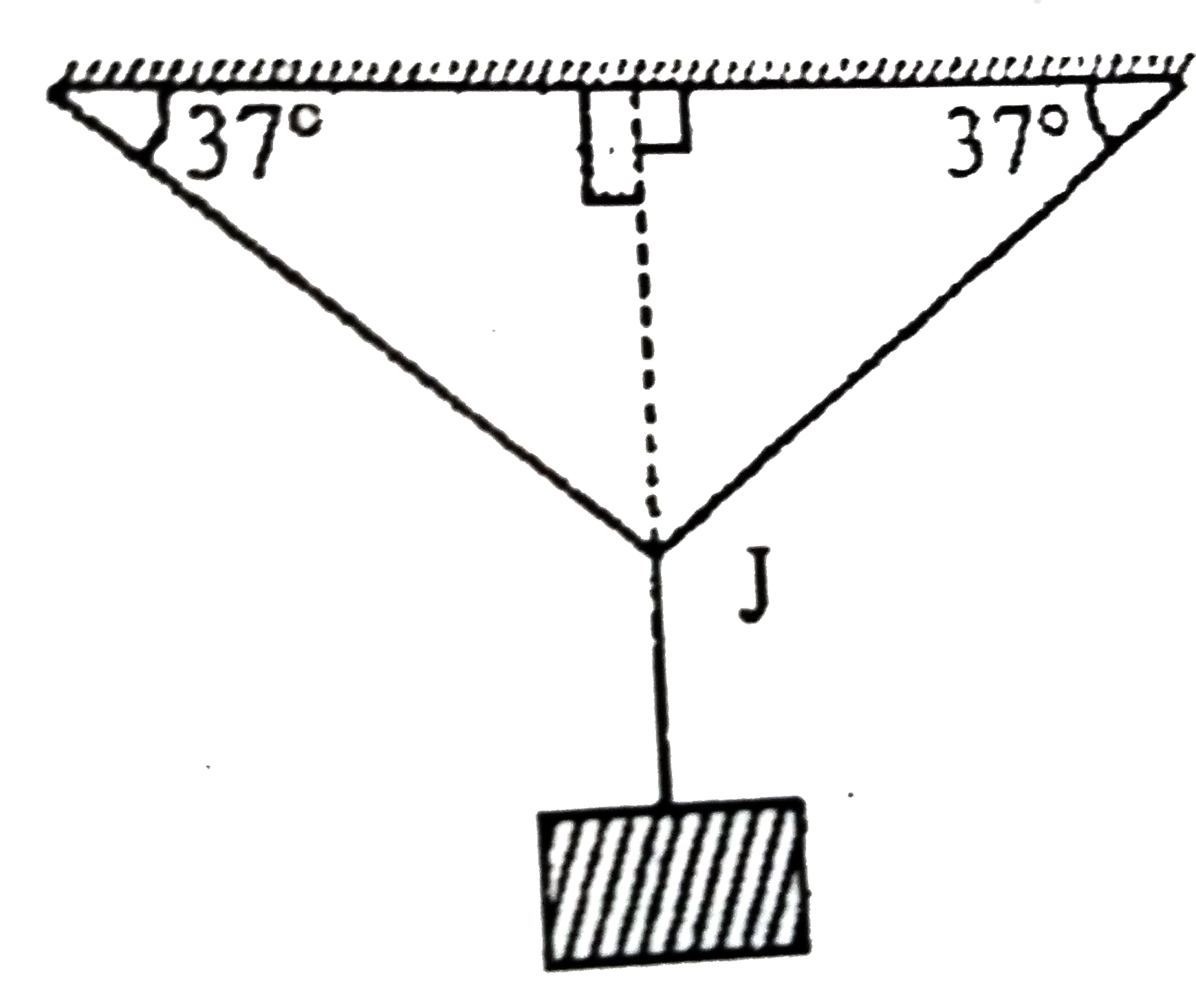 A10B12C14D16
A10B12C14D16
A thin rod of negligible mass and area of cross-section 4×10−6m2, suspended vertically from one end has a length of 0.5m at 10∘C. The rod is colled art 0∘C, but prevented from contracting by attaching a mass at the loqedr end. Find
(i) This mass and
(ii) The energy stored in the rod.
Given for this rod, Y=1011Nm−2, coefficient of linear expansion =10−5K−1 and g=10ms−2.
View SolutionFigure shows a steel rod of cross sectional ara 2×10−6m2 is fixed between two vertical walls. Initially at 20∘C there is no force between the ends of the rod and the walls. Find the force which the rod will exert on walls at 100∘C. Given that the coefficient of linear expansion of steel is 1.2×10−5.∘C−1 and Young's modulus is 2×1011N/m2

View SolutionThe area of cross-section of railway track is 0.01m2. The temperature variation is 10∘C. Coefficient of linear expansion of steel =10−5/∘C. (Young's modulus of steel =1011Nm−2). Calculate the energy stored per meter in the track.
View SolutionA uniform rod of cross-section 4mm2 is heated from 0∘C to 10∘C. The coefficient of linear expansion of the rod, ∝=12×10−6/∘C and Young's modulus =1011N/m2. The strain produced in the rod is
View SolutionAt 40∘C a brass wire of 1mm radius is hung from the ceiling. A small mass, M is hung from the free end of the wire. When the wire is cooled down from40∘C to 20∘C it regains its original length of 0.2 m the value of M is close to (coefficient of linear expansion and young's modulus of brass are 10−5/.∘C and 1011/N/m2 respectively g=10ms−2)
View Solution
