A nucleus is at rest in the laboratory frame of reference. Show that if it disintegrates into two smaller nuclei the products must be emitted in opposite directions.
Let , be the masses of products and , be their respective velocities. Therefore, total linear momentum after disintegration = . Before disintegration , the nucleus is at rest. Therefore, its linear momentum before disintegration is zero . According to the people of conservation of linear momentum,
+ = 0 or = /
Negative sign shows that and are in opposite directions.
Topper's Solved these Questions
Similar Questions
A nucleus is at rest in the laboratory frame of reference Show that if it distintegrates into two smaller nuclei the products must be emitted in opposite directions .
View SolutionFind the proper length of a rod if in the laboratory frame of reference its velocity is v=c/2, the length l=1.00m, and the angle between the rod and its direction of motion is θ=45∘.
View Solution
Knowledge Check
Assertion: Stationary waves are so called because particles are at rest in stationary waves.
Reason: They are formed by the superposition of two identical waves travelling in opposite directions.AIf both Assertion and Reason are true and the Reason is correct explanation of the Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf Assertion is true, but the Reason is false.DIf Assertion is false but the Reason is true.The radioactive nuclei are unstable and emit alpha (.2He4) , beta (.−1e0) and gamma (.0γ0) radiations to acheive states of greater stability. Rutherford and Soddy suggested the following rules governing the radioactive decay, (i) The algebric sum of charges (atomic numbers) before and after disintegration must be the same. (ii) The sum of mass numbers before and after disintegration must also be the same.
The particle emitted in the reaction .90Th234→.91Pa234+........ isAαBβCγDNone of theseA nucleus A, With a finite de-broglie wavelength λA, undergoes spontaneous fission into two nuclei B and C of equal mass. B flies in the same direction as that of A, While C flies in the opposite direction with a velocity equal to half of that of B . The de-Broglie wavelength λB and λB and C are respectively:
AλA,2λAB2λA,λACλA,λA2DλA2,λA
Two relativistic particles move at right angles to each other in a laboratory frame of reference, one with the velocity v1 and the other with the velocity v2. Find their relative velocity.
View SolutionTwo rods having the same proper length l0 move lengthwise toward each other parallel to a common axis with the same velocity v relative to the laboratory frame of reference. What is the length of each rod in the reference frame fixed to the other rod?
View SolutionTwo particles moving in a laboratory frame of reference along the same straight line with the same velocity v=(3/4)c strike against a stationary target with the time interval Δt=50ns. Find the proper distance between the particles prior to their hitting the target.
View SolutionA diatomic molecule two identicla atoms of mass m bound together a distance l apart is confined in a lab reference frame. At a certain time one of the two atoms is observed to have speed v0 and the other, moving in the opposite direction, speed v0/3.
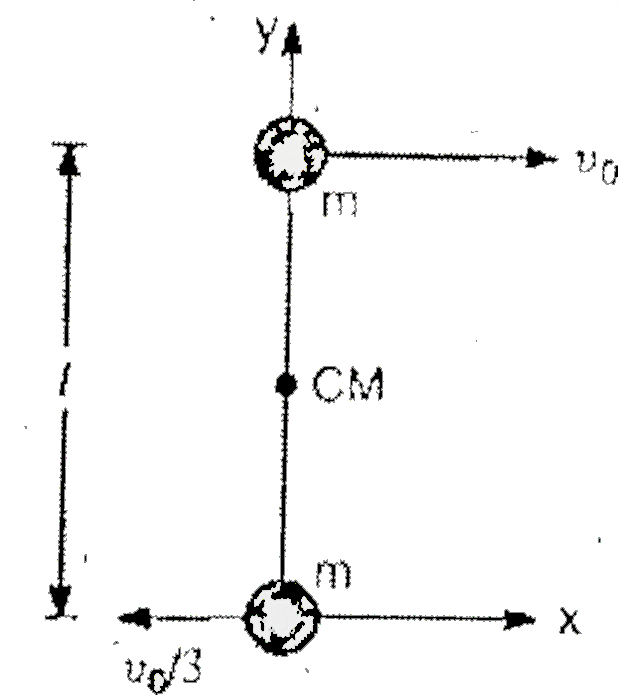
View SolutionA particular type of nucleus whose decay constant is λ is produced at a steady rate of p nuclei per second. Show that the number of nuclei N present t second after the production starts is N=Pλ[1−e~λt].
View Solution
