A block of mass 25 kg is raised by a 50kg man in two different ways as shown in fig. what is the action on the floor by the man in the two cases? If the floor yields to a normal force of 700N, which mode should the man adopt to lift the block without the floor yielding?
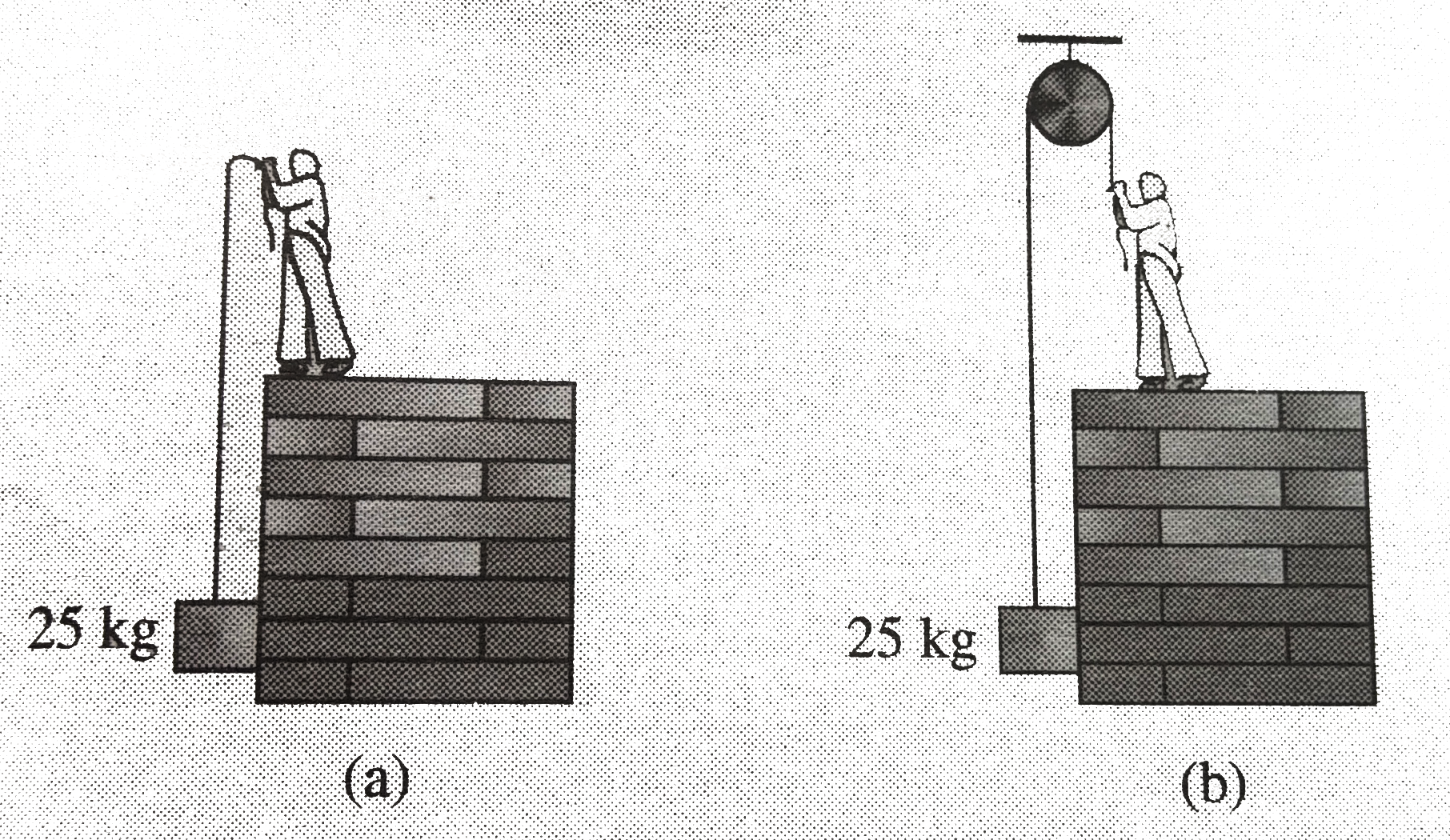
In 1st case , man applies an upward force of 25 kg wt.r ( same as the weight of the block ) . According to Newtons's third law of motion, there will be a downaward reaction on the floor.
The action on the floor by the man , = 50 kg wt + 25 kg wt = 75 kg wt = 75 kg ×10ms−2 = 750 N
In case II , the man applies a downaward force of 25 kg wt. According to Newton's third law, the reaction is in the upward direction.
In this case, action on the floor by the man
= 50 kg wt - 25 kg wt = 25 kg wt = 25kg×10ms−2 = 250 N
Therefore, the men should adopt the second method.
Topper's Solved these Questions
Similar Questions
In fig. the block of mass M is at rest on the floor . At what acceleration with which should a boy of mass m climb along the rope of negligible mass so as to lift the block from the floor?
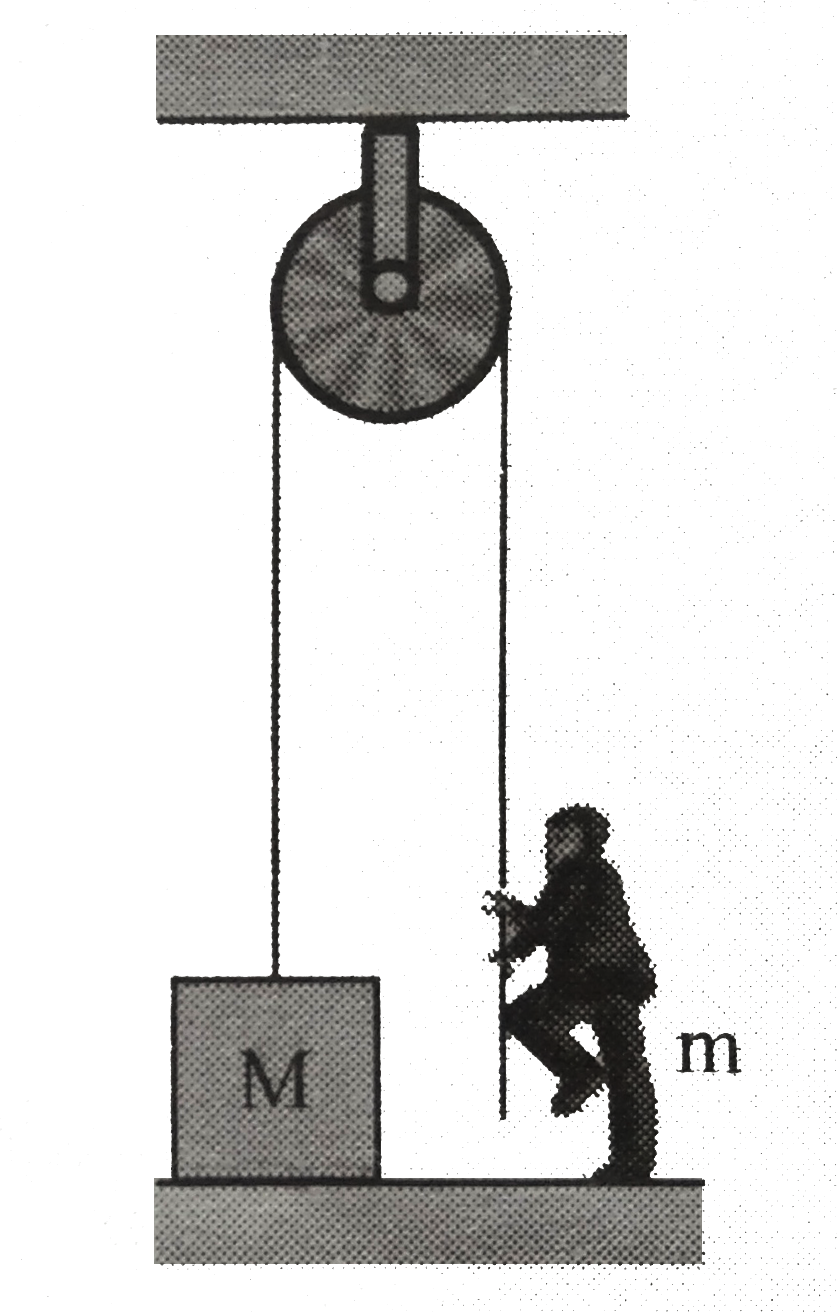
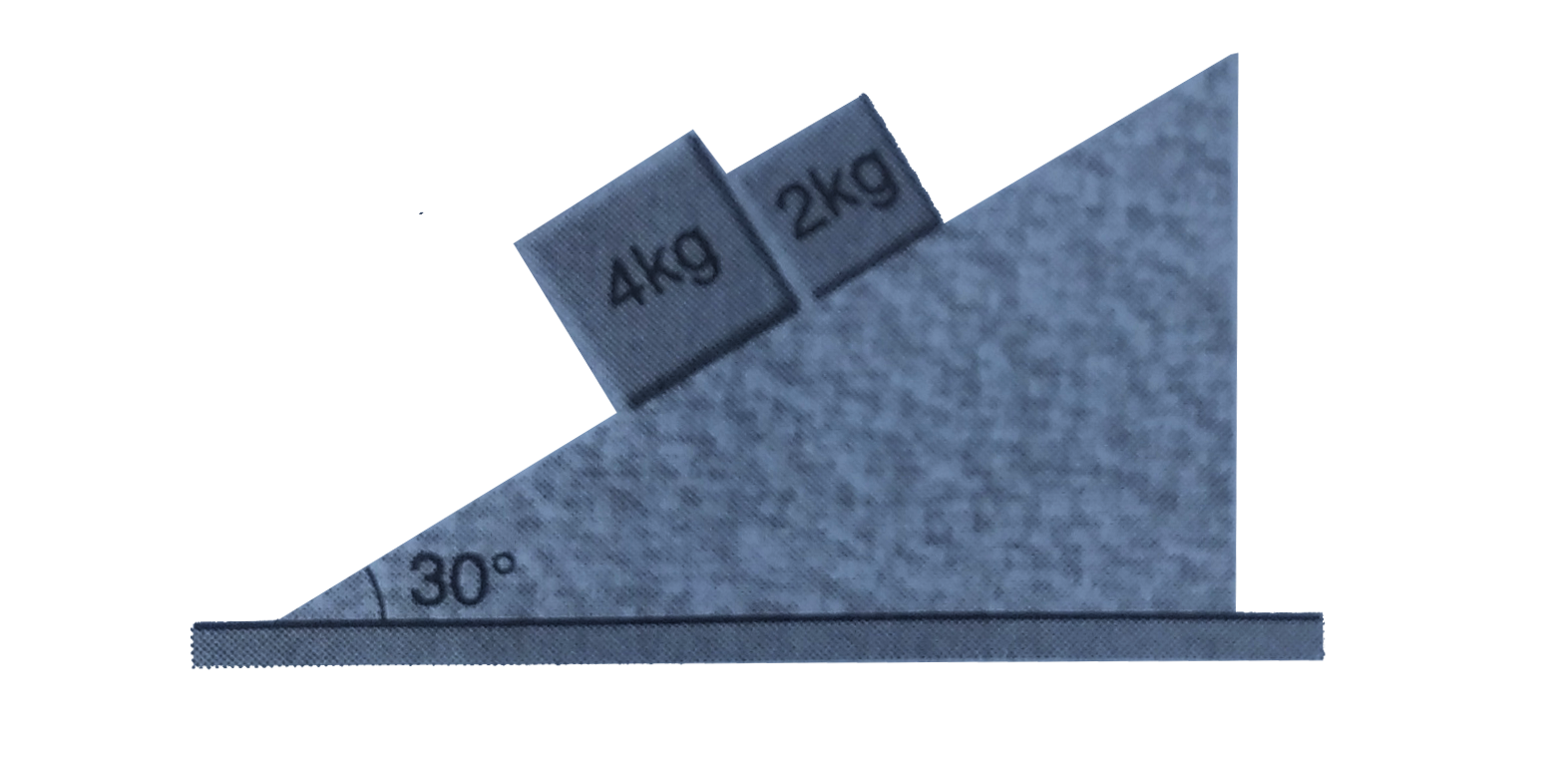
View SolutionTwo block of masses 2kg and 4kg are released from rest over a smooth inclined place of inclination 30∘ as shown in figure what is the normal force between the two blocks?
View Solution
Knowledge Check
A man of mass 90 kg is standing in a lift whose cable broke suddenly. If thelift falls freely, the force exerted by the floor on the man is
A90NB180NCZer ND−90NA block of mass 2 kg is placed on the floor. The coefficient of static friction is 0.4. A force F of 3 N is applied on the block as shown in figure. The force of friction between the block and the floor is (Take g=10ms−2)
 A1.5 NB3 NC4 ND2.5 N
A1.5 NB3 NC4 ND2.5 NA block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
A8.8 NB5.8 NC2.8 ND10.8 N
A man pushes a box of mass 50kg with a force of 80N. What will be the acceleration of the box? What would be the acceleration if the mass were halved?
View SolutionA wooden block of mass 2 kg rests on a soft horizontal floor . When aniron cylinder of mass 25 kg is placed on top of the block , the floor yields steadily , and the block and the cylinder go down with an acceleration of 0.1ms−2 What is the action of the block on the floor (a) before and (b) after the floor yields ? Take g=10ms−2 . Identify the action reaction pairs in the problem .
View SolutionA wooden block of mass 1 kg rests on a soft horizontal floor. When an iron cylinder of mass 20kg is placed on top of the block, the floor yields steadily and booth go down with an acceleration of 0.1ms−2. What is the action of the block on the floor (a) before and (b) after the floor yields? (Take g=10ms−2). Identify the action and reaction pairs in the problem.

View SolutionA block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View SolutionA block A of mass 2 kg rests on another block B of mass 8 kg which rests on a horizontal floor. The coefficient of friction between A and B is 0.2 while that between B and floor is 0.5. when a horizontal force of 25 N is applied on the block B. the force of friction between A and B is
View Solution
