Suppose the frequency of the source in the above example can be varied (a) What is the frequency of the source at which resonance occurs ? (b) Calculate the impedance, the current and power dissipated at the resonant condition.
(a) The frequency at which the resonance occurs is
ω0=1√LC=1√25.48×10−3×796×10−6
=222.1rad/s
vr=ω02π=221.12×3.14Hz=35.4Hz
(b) The impedance Z at resonant condition is equal to the resistance:
Z=R=3Ω
The rms current at resonance is
=VZ=VR=(283√2)13=66.7A
The power dissipated at resonance is
P=I2×R=(66.7)2×3=13.35kW
You can see that in the present case, power dissipated at resonance is more than the power dissipated in Example 7.8.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If the frequency of the source e.m.f. in an AC circuit is n, the power varies with a frequency:
AnB2nCn/2DzeroIf the frequency of the source e.m.f. in an AC circuit is n, the power varies with a frequency:
AnB2nCn/2DzeroIn a series R-L-C circuit, the frequency of the source is half of the resonance frequency. The nature of the circuit will be
AcapacitiveBinductiveCpurely resistiveDdata unsufficient
Figure here, shows a series L-C-R circuit connected to a variable frequency 230 V source. L = 5.0H, C = 80μF and r =40Ω
(a) Determine the source frequency which drives the circuit in resonance.
(b) Obtain the impedance of the circuit and the amplitude of current at the resonating frequency.
(c) Determine the rms potential drops across the three elements of the circuit. show that the potential drop across the L-C combination is zero at the resonating frequency.
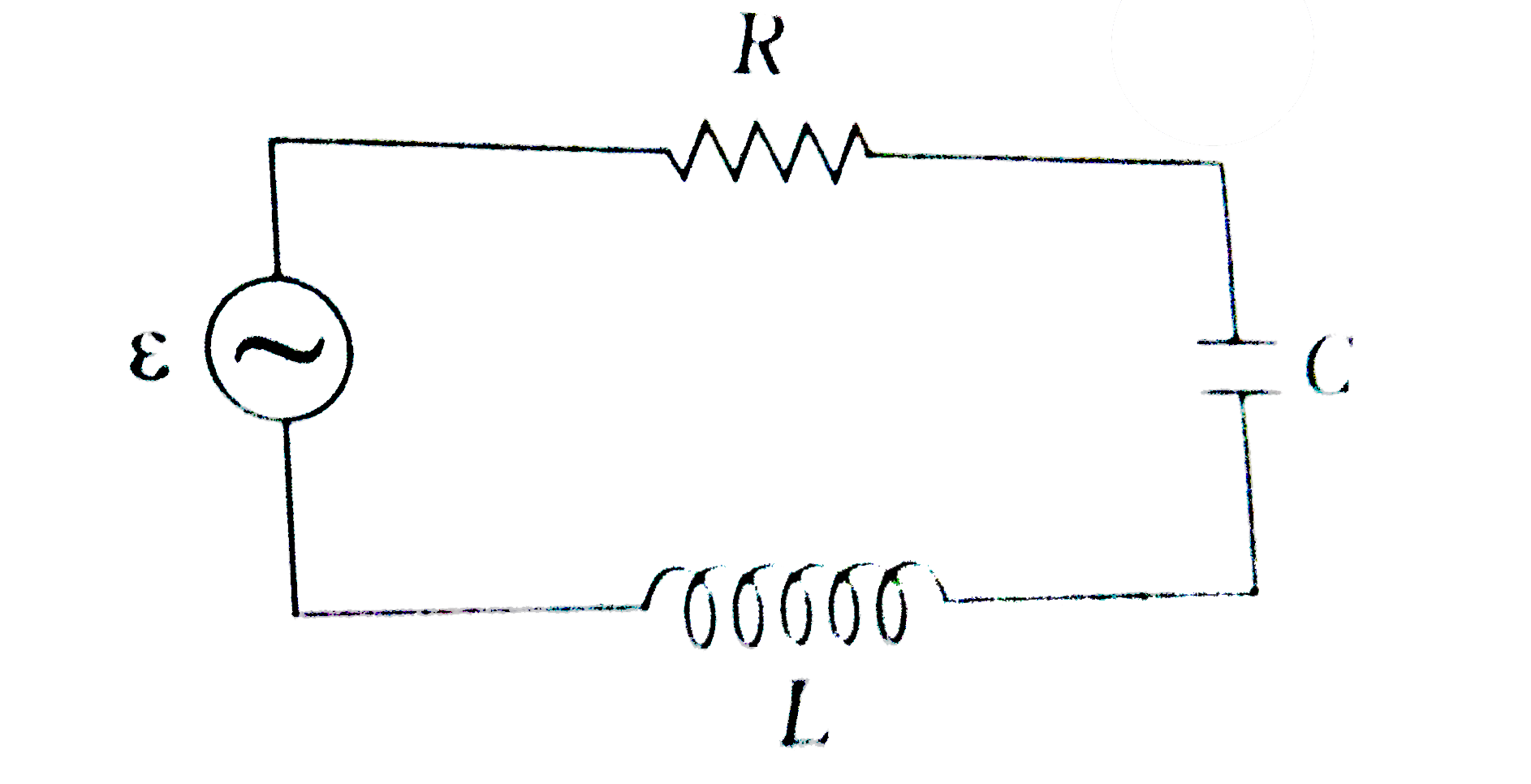
View SolutionA series LCR circuit with L=4.0h,C=100μF and R=60Ω is connected to a varialbe frequency 240 V source. Calcalate
(i) angular frequency of the source which drives the circuit in resonace,
(ii) current at the resonating frequency,
(iii) rms potential drop across the inductro at resonance.
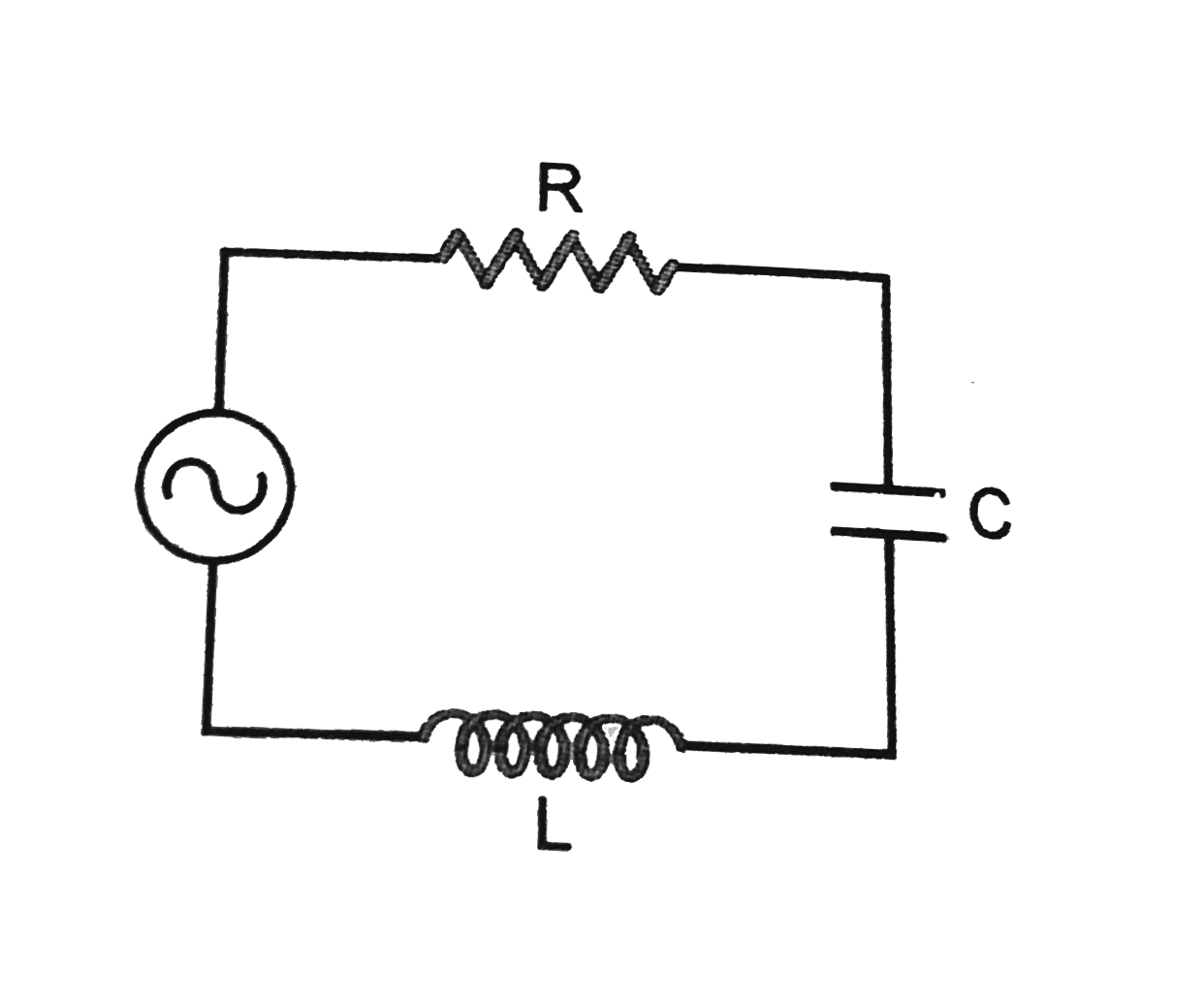
View SolutionA series LCR circuit connected to a variable frequency 230 V source has L = 5.0 H, C=80μF, R=40Ω, Fig.
(a) Determine the source frequency which drives the circuit in resonance.
(b) Obtain the impedance of the circuit and amplitude of current at at resonating frequency.
(c ) Determine the r.m.s. potential drops acorss the three elements the circuits. Show that the potential drop acorss the LC combination is zero at the resonating frequecny.
View SolutionWhen the frequency of the AC source in an LCR circuit equals the resonant frequency, the reactance of the circuit is zero. Does it mean that there is no current through the inductor or the capacitor?
View SolutionA 2 uF capacitor, 100 Ω resistor and 8 H inductor are connected in series with an a.c. source. Find the frequency of the a.c. source for which the current drawn in the circuit is maximum. If the peak value of emf of the source is 200 V, calculate the
(i) maximum current, and (ii) inductive and capacitive reactance of the circuit at resonance.
View Solution
