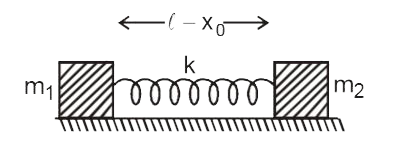
Two blocks of mass m1 and m2 are connected with a spring of natural length l and spring constant k. The system is lying on a smooth horizontal surface. Initially spring is compressed by x0 as shown in figure. Show that the two blocks will perform SHM about their equilibrium position. Also (a) find the time period, (b) find amplitude of each block and (c) length of spring as a function of time.
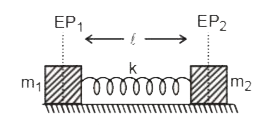
(a) Here both the blocks will be in equilibrium at the same time when spring is in its natural length. Let EP1 and EP2 be equilibrium positions of block A and B as shown in figure.
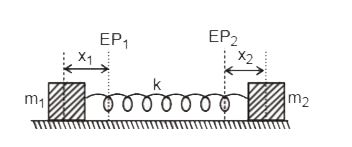
Let at any time during oscillations, blocks are at a distance of x1 and x2 from their equilibrium positions.
As no external force is acting on the spring block system
∴(m1+m2)Δxcm=m1x1−m2n2=0
or m1x1=m2x2
For 1st particle, force equation can be written as
k(x1+m1m2x1)=−m1a1
or a1=−k(m1+m2)m1m2x1
∴ ω1=−k(m1+m2)m1m2
Hence T = 2π√m1m2k(m1+m2)=2π√μK
were μ=m1m2(m1+m2) which is know as reduced mass
(b) Let the amplitude of blocks be A1 and A2
m1A1=m2A2
By energy conservation ,
12k(A1+A2)2=12kx20
or A1+A2=x0
or A1+A2=x0
or , A1+m1m2A1=x0
or A1=m2x0m1+m2
A2=m1x0m1+m2
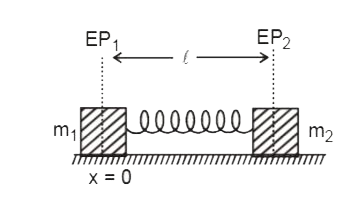
(c) Let equilibrium position of 1st particle be origin, i.e.,
x = 0.
x co-ordinate of particles can be written as
x1=A1cosωt and x2=l−A2cosωt
Hence, length of spring can be written as :
length =x2−x1
=l−(A1+A2)cosωt
Topper's Solved these Questions
- SIMPLE HARONIC MOTION BOOK - MOTIONCHAPTER - SIMPLE HARONIC MOTION EXERCISE - EXERCISE -1 ( SECTION-A )
8 Videos
- SIMPLE HARONIC MOTION BOOK - MOTIONCHAPTER - SIMPLE HARONIC MOTION EXERCISE - EXERCISE -1 ( SECTION-B ) (Time per iod and angu larfrequency in SHM)
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - MOTIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - EXERCISE -3 Section - B Previous Year Problems | JEE MAIN
23 Videos
- SOUND WAVESBOOK - MOTIONCHAPTER - SOUND WAVESEXERCISE - Exercise - 3 (Section - B)
14 Videos
Similar Questions
The block of mass m1 and m2 are connected with a spring of netural length l and spring constant k. The systeam is lying on a smoth horizontal surface. Initially spring is compressed by x0 as shown in figure.
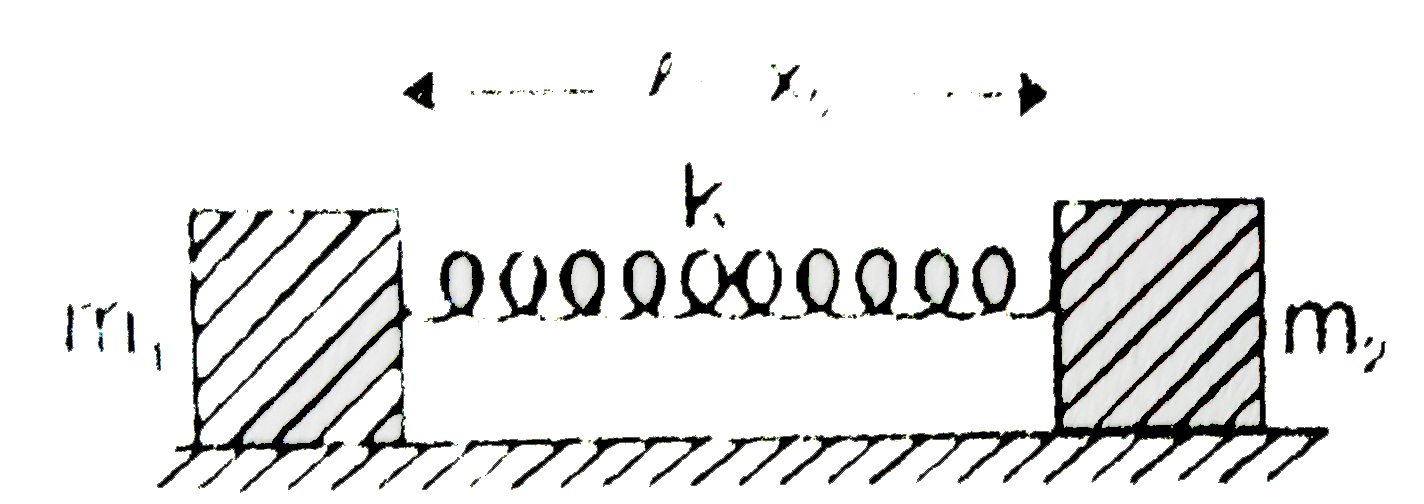
Show that the two blocks will perform SHM about their equilibrium position. Also (a) find the time period, (b) find amplitude of each block and (c) length of spring as a funcation of time.
View SolutionThe block of mass m1 and m2 are connected with a spring of netural length l and spring constant k. The systeam is lying on a smoth horizontal surface. Initially spring is compressed by x0 as shown in figure.
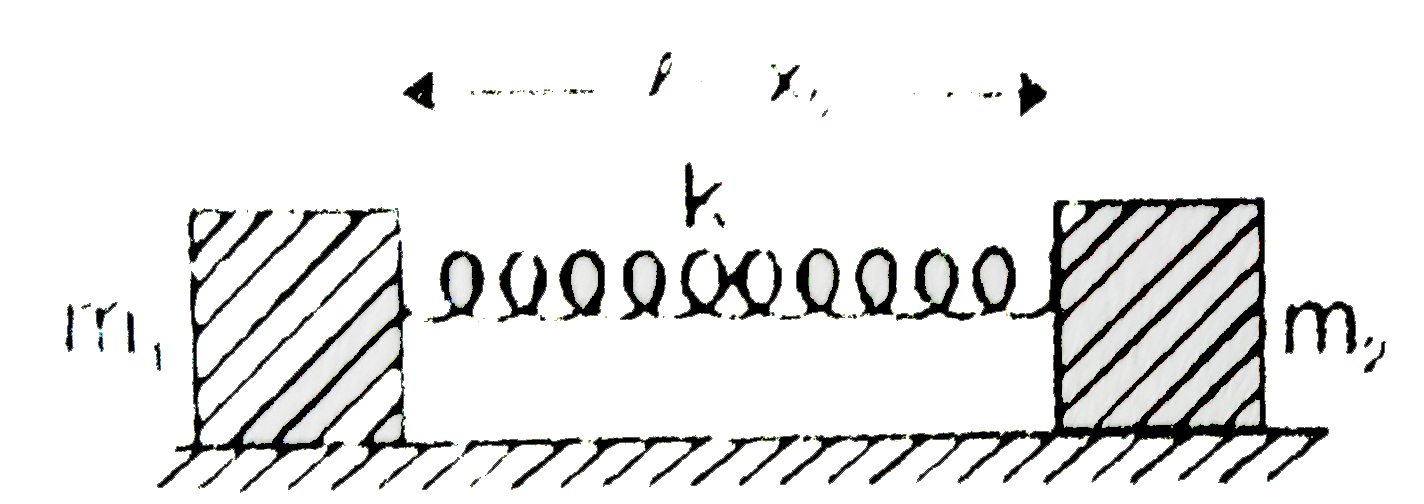
Show that the two blocks will perform SHM about their equilibrium position. Also (a) find the time period, (b) find amplitude of each block and (c) length of spring as a funcation of time.
View Solution
Knowledge Check
Two blocks of masses m1 and m2 are connected by an ideal sprit, of force constant k. The blocks are placed on smooth horizontal surface. A horizontal force F acts on the block m1. Initially spring is relaxed, both the blocks are at rest.
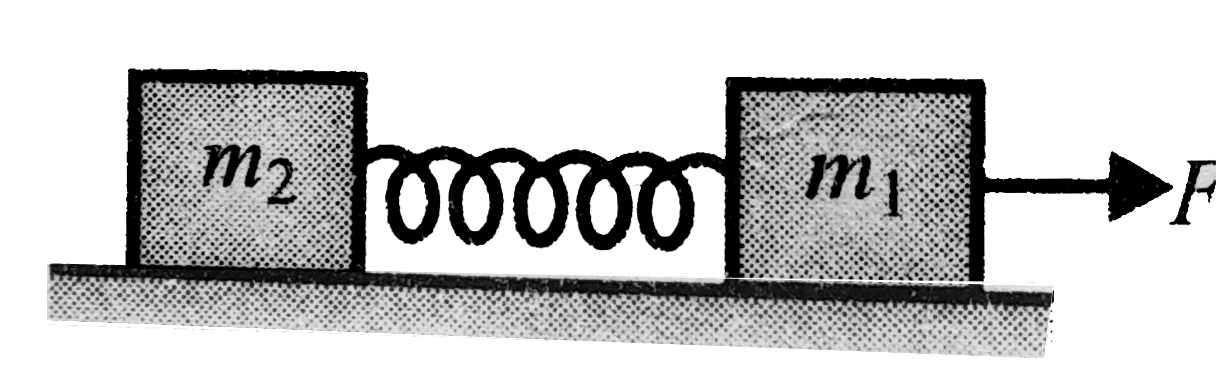
What is maximum elongation of spring.A2m1F(m1+m2)kBm21F2(m1+m2)kC2m2Fk(m1+m2)Dm22F2(m1+m2)2kTwo blocks of masses m1 and m2 are connected by a massless spring and placed on smooth surface. The spring initially stretched and released. Then :
Athe momentum of each particle remains constant separatelyBthe magnitude of momentums of each body are equal to each otherCthe mechanical energy of system remains constantDboth (C) and (B) are correctTwo blocks A and B, each of mass m, are connected by a masslesss spring of natural length L and spring constant K. The blocks are initially resting on a smooth horizontal floor with the spring at its natural length, as shown in fig. A third identical block C, also of mass m, moves on the floor with a speed v along the line joining A and B, and collides elastically with A. Then
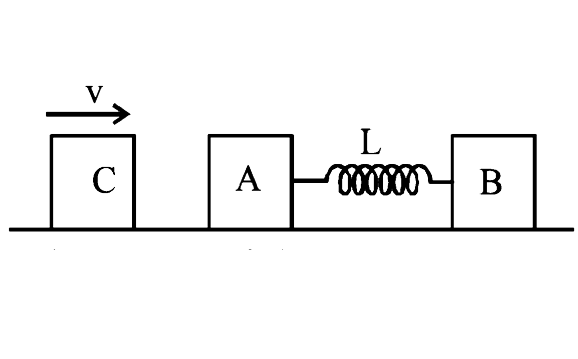 Athe kinetic energy of the A-B system, at maximum compression of the spring, is zero.Bthe kinetic energy of the A-B system, at maximum compression of the spring, is mv2/4.Cthe maximum compression of the spring is v√(m/K)Dthe maximum compression of the spring is v√(m/2K)
Athe kinetic energy of the A-B system, at maximum compression of the spring, is zero.Bthe kinetic energy of the A-B system, at maximum compression of the spring, is mv2/4.Cthe maximum compression of the spring is v√(m/K)Dthe maximum compression of the spring is v√(m/2K)
Block of mass 2m is given v0 towards the right. If L is the natural length of spring constant k, find the maximum elongation of the spring.

View SolutionThe block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionTwo blocks A and B of masses m and 2m are connected by a massless spring of natural length L and spring constant k. The blocks are intially resting on a smooth horizontal floor with the spring at its natural length , as shown . A third identical block C of mass m moves on the floor with a speed v0 along the line joining A and B and collides elastically with A. FInd (a) the velocity of c.m. of system (blockA+B+spring) and (b) the minimum compression of spring.

View SolutionTwo blocks A and B of mass m and 2m respectively are connected by a light spring of force constant k. They are placed on a smooth horizontal surface. Spring is stretched by a length x and then released. Find the relative velocity of the blocks when the spring comes to its natural length

View SolutionTwo blocks of masses m1 and m2 are connected by an ideal sprit, of force constant k. The blocks are placed on smooth horizontal surface. A horizontal force F acts on the block m1. Initially spring is relaxed, both the blocks are at rest.

What is acceleration of centre of mass of system at the instant of maximum elongation of spring
View Solution
